Opinion de-polarization of social networks with GNNs

Nowadays, social media is the ground for political debate and exchange of opinions. There is a significant amount of research that suggests that social media are highly polarized. A phenomenon that is commonly observed is the echo chamber structure, where users are organized in polarized communities and form connections only with similar-minded individuals, limiting themselves to consume specific content. In this paper we explore a way to decrease the polarization of networks with two echo chambers. Particularly, we observe that if some users adopt a moderate opinion about a topic, the polarization of the network decreases. Based on this observation, we propose an efficient algorithm to identify a good set of K users, such that if they adopt a moderate stance around a topic, the polarization is minimized. Our algorithm employs a Graph Neural Network and thus it can handle large graphs more effectively than other approaches

FedDec: Peer-to-peer Aided Federated Learning

Federated learning (FL) has enabled training machine learning models exploiting the data of multiple agents without compromising privacy. However, FL is known to be vulnerable to data heterogeneity, partial device participation, and infrequent communication with the server, which are nonetheless three distinctive characteristics of this framework. While much of the recent literature has tackled these weaknesses using different tools, only a few works have explored the possibility of exploiting inter-agent communication to improve FL's performance. In this work, we present FedDec, an algorithm that interleaves peer-to-peer communication and parameter averaging (similar to decentralized learning in networks) between the local gradient updates of FL. We analyze the convergence of FedDec under the assumptions of non-iid data distribution, partial device participation, and smooth and strongly convex costs, and show that inter-agent communication alleviates the negative impact of infrequent communication rounds with the server by reducing the dependence on the number of local updates $H$ from $O(H^2)$ to $O(H)$. Furthermore, our analysis reveals that the term improved in the bound is multiplied by a constant that depends on the spectrum of the inter-agent communication graph, and that vanishes quickly the more connected the network is. We confirm the predictions of our theory in numerical simulations, where we show that FedDec converges faster than FedAvg, and that the gains are greater as either $H$ or the connectivity of the network increase.

Pick your Neighbor: Local Gauss-Southwell Rule for Fast Asynchronous Decentralized Optimization

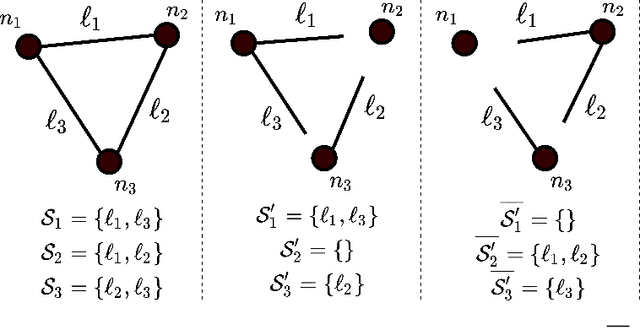
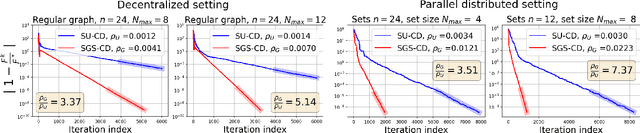
In decentralized optimization environments, each agent $i$ in a network of $n$ optimization nodes possesses a private function $f_i$, and nodes communicate with their neighbors to cooperatively minimize the aggregate objective $\sum_{i=1}^n f_i$. In this setting, synchronizing the nodes' updates incurs significant communication overhead and computational costs, so much of the recent literature has focused on the analysis and design of asynchronous optimization algorithms where agents activate and communicate at arbitrary times, without requiring a global synchronization enforcer. Nonetheless, in most of the work on the topic, active nodes select a neighbor to contact based on a fixed probability (e.g., uniformly at random), a choice that ignores the optimization landscape at the moment of activation. Instead, in this work we introduce an optimization-aware selection rule that chooses the neighbor with the highest dual cost improvement (a quantity related to a consensus-based dualization of the problem at hand). This scheme is related to the coordinate descent (CD) method with a Gauss-Southwell (GS) rule for coordinate updates; in our setting however, only a subset of coordinates is accessible at each iteration (because each node is constrained to communicate only with its direct neighbors), so the existing literature on GS methods does not apply. To overcome this difficulty, we develop a new analytical framework for smooth and strongly convex $f_i$ that covers the class of set-wise CD algorithms -- a class that directly applies to decentralized scenarios, but is not limited to them -- and we show that the proposed set-wise GS rule achieves a speedup by a factor of up to the maximum degree in the network (which is of the order of $\Theta(n)$ in highly connected graphs). The speedup predicted by our theoretical analysis is subsequently validated in numerical experiments with synthetic data.

Model-Driven Artificial Intelligence for Online Network Optimization

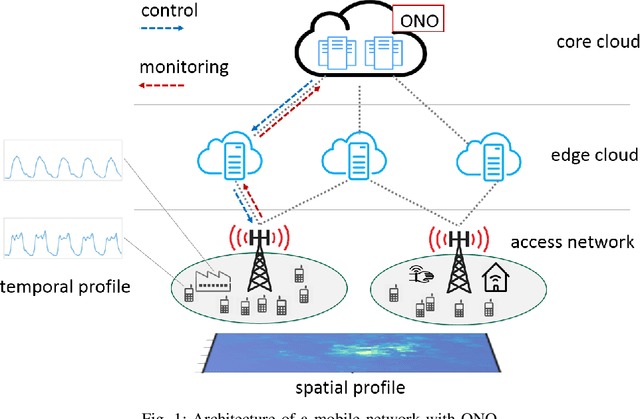
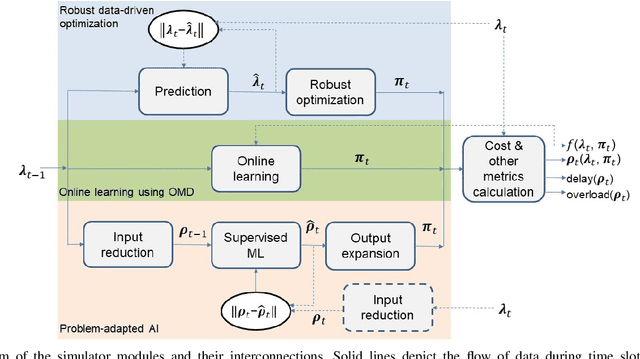
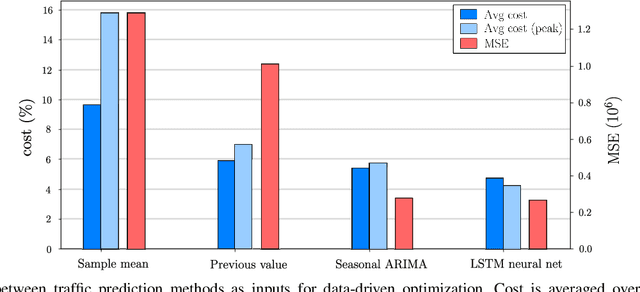
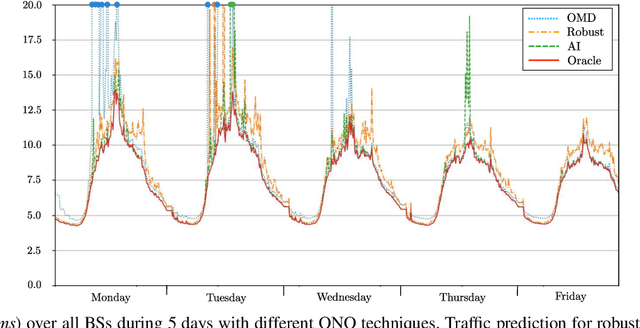
Future 5G wireless networks will rely on agile and automated network management, where the usage of diverse resources must be jointly optimized with surgical accuracy. A number of key wireless network functionalities (e.g., traffic steering, energy savings) give rise to hard optimization problems. What is more, high spatio-temporal traffic variability coupled with the need to satisfy strict per slice/service SLAs in modern networks, suggest that these problems must be constantly (re-)solved, to maintain close-to-optimal performance. To this end, in this paper we propose the framework of Online Network Optimization (ONO), which seeks to maintain both agile and efficient control over time, using an arsenal of data-driven, adaptive, and AI-based techniques. Since the mathematical tools and the studied regimes vary widely among these methodologies, a theoretical comparison is often out of reach. Therefore, the important question "what is the right ONO technique?" remains open to date. In this paper, we discuss the pros and cons of each technique and further attempt a direct quantitative comparison for a specific use case, using real data. Our results suggest that carefully combining the insights of problem modeling with state-of-the-art AI techniques provides significant advantages at reasonable complexity.