Score-Based Diffusion Models for Photoacoustic Tomography Image Reconstruction

Photoacoustic tomography (PAT) is a rapidly-evolving medical imaging modality that combines optical absorption contrast with ultrasound imaging depth. One challenge in PAT is image reconstruction with inadequate acoustic signals due to limited sensor coverage or due to the density of the transducer array. Such cases call for solving an ill-posed inverse reconstruction problem. In this work, we use score-based diffusion models to solve the inverse problem of reconstructing an image from limited PAT measurements. The proposed approach allows us to incorporate an expressive prior learned by a diffusion model on simulated vessel structures while still being robust to varying transducer sparsity conditions.

Fuzzy Forests For Feature Selection in High-Dimensional Survey Data: An Application to the 2020 U.S. Presidential Election

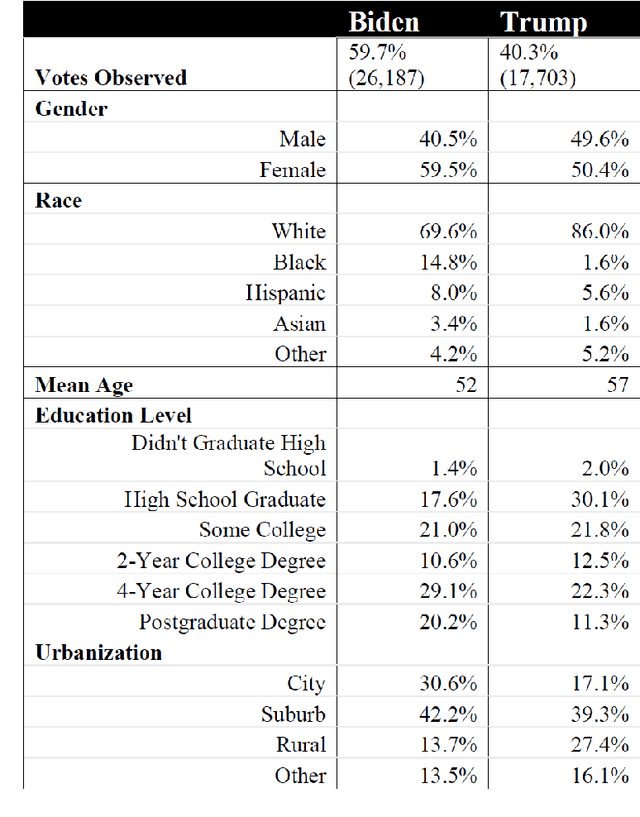
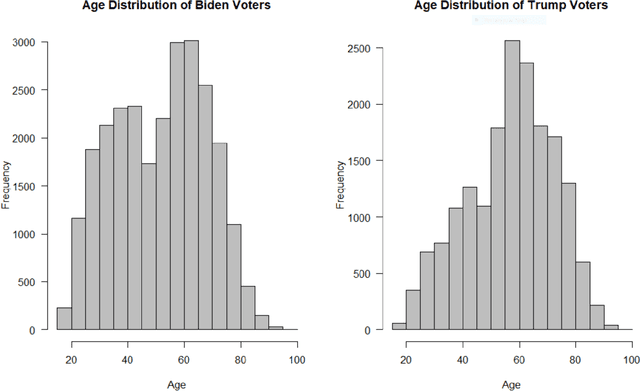
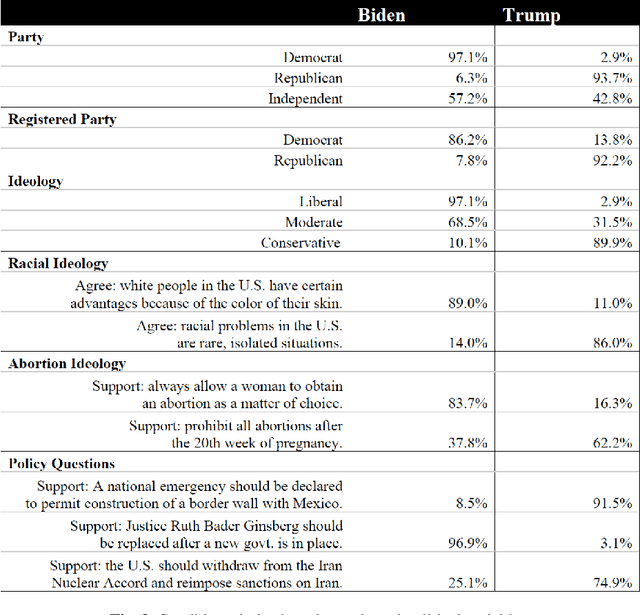
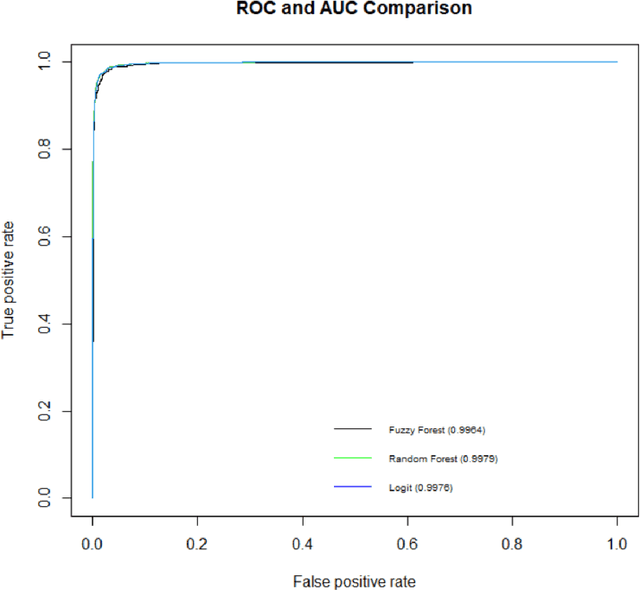
An increasingly common methodological issue in the field of social science is high-dimensional and highly correlated datasets that are unamenable to the traditional deductive framework of study. Analysis of candidate choice in the 2020 Presidential Election is one area in which this issue presents itself: in order to test the many theories explaining the outcome of the election, it is necessary to use data such as the 2020 Cooperative Election Study Common Content, with hundreds of highly correlated features. We present the Fuzzy Forests algorithm, a variant of the popular Random Forests ensemble method, as an efficient way to reduce the feature space in such cases with minimal bias, while also maintaining predictive performance on par with common algorithms like Random Forests and logit. Using Fuzzy Forests, we isolate the top correlates of candidate choice and find that partisan polarization was the strongest factor driving the 2020 presidential election.