Quantum-Based Feature Selection for Multi-classification Problem in Complex Systems with Edge Computing

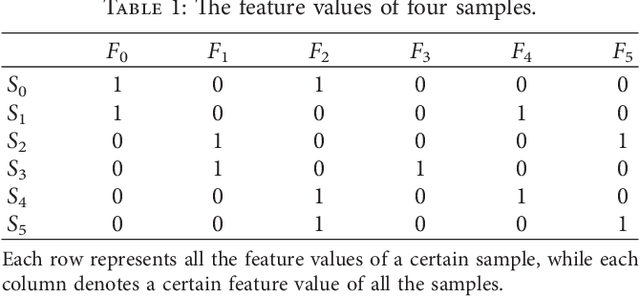
The complex systems with edge computing require a huge amount of multi-feature data to extract appropriate insights for their decision making, so it is important to find a feasible feature selection method to improve the computational efficiency and save the resource consumption. In this paper, a quantum-based feature selection algorithm for the multi-classification problem, namely, QReliefF, is proposed, which can effectively reduce the complexity of algorithm and improve its computational efficiency. First, all features of each sample are encoded into a quantum state by performing operations CMP and R_y, and then the amplitude estimation is applied to calculate the similarity between any two quantum states (i.e., two samples). According to the similarities, the Grover-Long method is utilized to find the nearest k neighbor samples, and then the weight vector is updated. After a certain number of iterations through the above process, the desired features can be selected with regards to the final weight vector and the threshold {\tau}. Compared with the classical ReliefF algorithm, our algorithm reduces the complexity of similarity calculation from O(MN) to O(M), the complexity of finding the nearest neighbor from O(M) to O(sqrt(M)), and resource consumption from O(MN) to O(MlogN). Meanwhile, compared with the quantum Relief algorithm, our algorithm is superior in finding the nearest neighbor, reducing the complexity from O(M) to O(sqrt(M)). Finally, in order to verify the feasibility of our algorithm, a simulation experiment based on Rigetti with a simple example is performed.

A Unitary Weights Based One-Iteration Quantum Perceptron Algorithm for Non-Ideal Training Sets

In order to solve the problem of non-ideal training sets (i.e., the less-complete or over-complete sets) and implement one-iteration learning, a novel efficient quantum perceptron algorithm based on unitary weights is proposed, where the singular value decomposition of the total weight matrix from the training set is calculated to make the weight matrix to be unitary. The example validation of quantum gates {H, S, T, CNOT, Toffoli, Fredkin} shows that our algorithm can accurately implement arbitrary quantum gates within one iteration. The performance comparison between our algorithm and other quantum perceptron algorithms demonstrates the advantages of our algorithm in terms of applicability, accuracy, and availability. For further validating the applicability of our algorithm, a quantum composite gate which consists of several basic quantum gates is also illustrated.