Physics-Constrained Neural Network for the Analysis and Feature-Based Optimization of Woven Composites

Woven composites are produced by interlacing warp and weft fibers in a pattern or weave style. By changing the pattern or material, the mechanical properties of woven composites can be significantly changed; however, the role of woven composite architecture (pattern, material) on the mechanical properties is not well understood. In this paper, we explore the relationship between woven composite architectures (weave pattern, weave material sequence) and the corresponding modulus through our proposed Physics-Constrained Neural Network (PCNN). Furthermore, we apply statistical learning methods to optimize the woven composite architecture to improve mechanical responses. Our results show that PCNN can effectively predict woven architecture for the desired modulus with much higher accuracy than several baseline models. PCNN can be further combined with feature-based optimization to determine the optimal woven composite architecture at the initial design stage. In addition to relating woven composite architecture to its mechanical responses, our research also provides an in-depth understanding of how architectural features govern mechanical responses. We anticipate our proposed frameworks will primarily facilitate the woven composite analysis and optimization process and be a starting point to introduce Physics knowledge-guided Neural Networks into the complex structural analysis.

Optimal tool path planning for 3D printing with spatio-temporal and thermal constraints

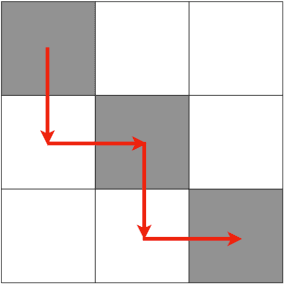
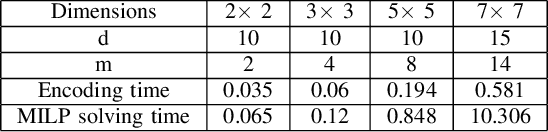
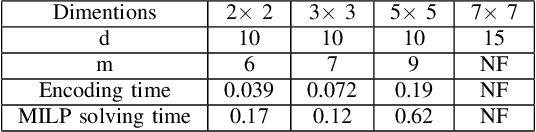
In this paper, we address the problem of synthesizing optimal path plans in a 2D subject to spatio-temporal and thermal constraints. Our solution consists of reducing the path planning problem to a Mixed Integer Linear Programming (MILP) problem. The challenge is in encoding the implication constraints in the path planning problem using only conjunctions that are permitted by the MILP formulation. Our experimental analysis using an implementation of the encoding in a Python toolbox demonstrates the feasibility of our approach in generating the optimal plans.

Difference-Based Deep Learning Framework for Stress Predictions in Heterogeneous Media

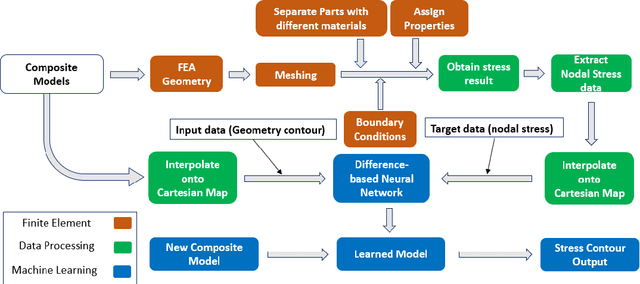
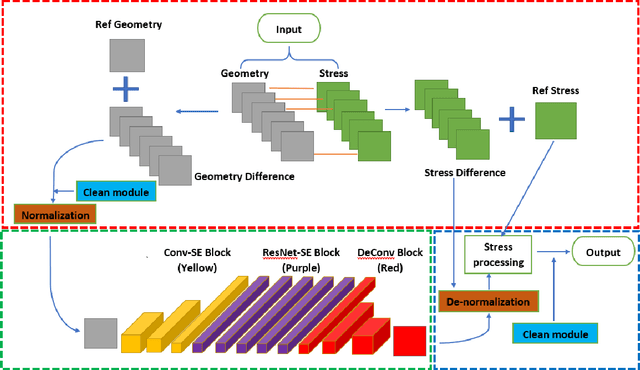
Stress analysis of heterogeneous media, like composite materials, using finite element method (FEM) has become commonplace in design and analysis. However, calculating stresses and determining stress distributions in heterogeneous media using FEM can be computationally expensive in situations like optimization and multi-scaling, where several design iterations are required to be tested iteratively until convergence. In this paper, we utilize deep learning and develop a set of Difference-based Neural Network (DNN) frameworks based on engineering and statistics knowledge to determine stress distribution in heterogeneous media with special focus on discontinuous domains that manifest high stress concentrations. To evaluate the performance of DNN frameworks, we consider four different types of geometric models that are commonly used in the analysis of composite materials: plate with circular cutout, square packed fiber reinforced, hexagonal packed fiber reinforced and hollow particle reinforced models. The proposed DNN structure consists of a normalization module (DNN-N) for all geometries considered, while we additionally introduce a clean module with DNN-N, named DNN-NC, for geometries with discontinuities. Results show that the DNN structures (DNN-N and DNN-NC) significantly enhance the accuracy of stress prediction compared to existing structures for all four models considered, especially when localized high stress concentrations are present in the geometric models.