Performance Analysis of Flexible Duplex Inter-Satellite Links in LEO Networks

This paper investigates energy-efficient inter-satellite communication in Low Earth Orbit (LEO) networks, where satellites exchange both buffered and newly generated data through half-duplex inter-satellite links (ISLs). Due to orbital motion and interference-prone directional asymmetry, the achievable ISL capacities in opposite directions vary dynamically, leading to inefficient utilization under conventional fixed or alternating duplex modes. To address this, we propose a Flexible Duplex (FlexD) scheme that adaptively selects the ISL transmission direction in each slot to maximize instantaneous end-to-end sky-to-ground throughput, jointly accounting for ISL quality, downlink conditions, and queue backlogs. A unified analytical framework is developed that transforms the bottleneck rate structure into an equivalent SINR domain, enabling closed-form derivations of throughput outage probability and energy efficiency under deterministic ISLs and Rician satellite-to-ground fading. The analysis reveals distinct operating regions governed by ISL and backlog constraints and provides tractable bounds for ergodic rate and energy efficiency. Numerical results confirm that FlexD achieves higher reliability and up to 30% improvement in energy efficiency compared with conventional half- and full-duplex schemes under realistic inter-satellite interference conditions.

Standard Condition Number-Based Detection for MIMO ISAC Systems under Noise Uncertainty

This paper presents a unified analytical and optimization framework for Standard Condition Number (SCN)-based detection in MIMO Integrated Sensing and Communication (ISAC) systems operating under noise uncertainty. Conventional detectors such as the Likelihood Ratio Test (LRT) and Energy Detector (ED) suffer from false-alarm inflation when interference or jamming alters the noise covariance. To overcome this limitation, the SCN detector, defined as the ratio of the largest to smallest eigenvalues of the sample covariance matrix is analytically characterized for the first time in an ISAC setting. Closed-form expressions for the false-alarm and detection probabilities are derived using random matrix theory for a two-antenna sensing receiver and generalized to arbitrary MIMO dimensions. The analysis proves that the SCN maintains a constant false alarm rate (CFAR) property and remains resilient to covariance mismatch, providing theoretical justification for its robustness in dynamic environments. Leveraging these results, a tractable ISAC power-allocation problem is formulated to minimize total detection error subject to communication rate and power constraints, yielding an interpretable sequential solution. Numerical evaluations verify the theory and demonstrate that the proposed SCN detector consistently outperforms LRT and eigenvalue-based benchmarks, particularly under strong interference and jamming typical of modern multiuser networks.

Performance Analysis for Multi-User Holographic MIMO Downlink with Matched Filter Precoding

Holographic MIMO (HMIMO) has emerged as a promising solution for future wireless systems by enabling ultra-dense, spatially continuous antenna deployments. While prior studies have primarily focused on electromagnetic (EM) modeling or simulation-based performance analysis, a rigorous communication-theoretic framework remains largely unexplored. This paper presents the first analytical performance study of a multi-user HMIMO downlink system with matched filter (MF) precoding - a low-complexity baseline scheme. By incorporating multipath propagation, mutual coupling, and element excitation, we derive a novel closed-form expression for the MF signal-to-interference-plus-noise ratio (SINR) using an equivalent random variable model. Leveraging bivariate gamma distributions, we then develop tractable throughput approximations under full, partial, and no channel state information (CSI) scenarios. Additionally, we formulate a max-min beamforming problem to benchmark optimal user fairness performance. Numerical results validate the accuracy of the proposed framework and reveal that MF precoding achieves competitive performance with strong robustness to low SINR and CSI uncertainty.

Null-Shaping for Interference Mitigation in LEO Satellites Under Location Uncertainty

Radio frequency interference (RFI) poses a growing challenge to satellite communications, particularly in uplink channels of Low Earth Orbit (LEO) systems, due to increasing spectrum congestion and uncertainty in the location of terrestrial interferers. This paper addresses the impact of RFI source position uncertainty on beamforming-based interference mitigation. First, we analytically characterize how geographic uncertainty in RFI location translates into angular deviation as observed from the satellite. Building on this, we propose a robust null-shaping framework to increase resilience in the communication links by incorporating the probability density function (PDF) of the RFI location uncertainty into the beamforming design via stochastic optimization. This allows adaptive shaping of the antenna array's nulling pattern to enhance interference suppression under uncertainty. Extensive Monte Carlo simulations, incorporating realistic satellite orbital dynamics and various RFI scenarios, demonstrate that the proposed approach achieves significantly improved mitigation performance compared to conventional deterministic designs.

Eigenvalue-Based Detection in MIMO Systems for Integrated Sensing and Communication

This paper considers a MIMO Integrated Sensing and Communication (ISAC) system, where a base station simultaneously serves a MIMO communication user and a remote MIMO sensing receiver, without channel state information (CSI) at the transmitter. Existing MIMO ISAC literature often prioritizes communication rate or detection probability, typically under constant false-alarm rate (CFAR) assumptions, without jointly analyzing detection reliability and communication constraints. To address this gap, we adopt an eigenvalue-based detector for robust sensing and use a performance metric, the total detection error, that jointly captures false-alarm and missed-detection probabilities. We derive novel closed-form expressions for both probabilities under the eigenvalue detector, enabling rigorous sensing analysis. Using these expressions, we formulate and solve a joint power allocation and threshold optimization problem that minimizes total detection error while meeting a minimum communication rate requirement. Simulation results demonstrate that the proposed joint design substantially outperforms conventional CFAR-based schemes, highlighting the benefits of power- and threshold-aware optimization in MIMO ISAC systems.

Uniform Planar Array Based Weighted Cooperative Spectrum Sensing for Cognitive Radio Networks

Cooperative spectrum sensing (CSS) is essential for improving the spectrum efficiency and reliability of cognitive radio applications. Next-generation wireless communication networks increasingly employ uniform planar arrays (UPA) due to their ability to steer beamformers towards desired directions, mitigating interference and eavesdropping. However, the application of UPA-based CSS in cognitive radio remains largely unexplored. This paper proposes a multi-beam UPA-based weighted CSS (WCSS) framework to enhance detection reliability, applicable to various cognitive radio networks, including cellular, vehicular, and satellite communications. We first propose a weighting factor for commonly used energy detection (ED) and eigenvalue detection (EVD) techniques, based on the spatial variation of signal strengths resulting from UPA antenna beamforming. We then analytically characterize the performance of both weighted ED and weighted EVD by deriving closed-form expressions for false alarm and detection probabilities. Our numerical results, considering both static and dynamic user behaviors, demonstrate the superiority of WCSS in enhancing sensing performance compared to uniformly weighted detectors.

Dynamic Cooperative MAC Optimization in RSU-Enhanced VANETs: A Distributed Approach

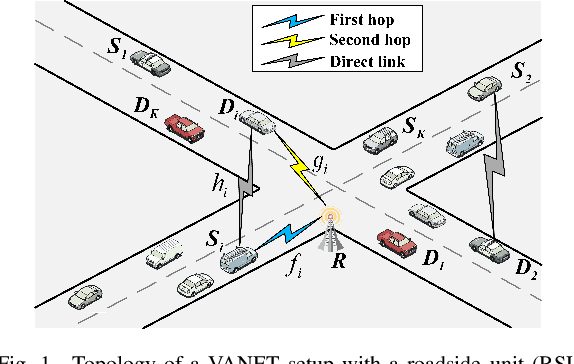
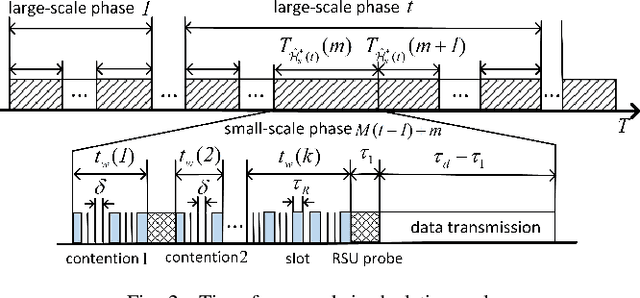
This paper presents an optimization approach for cooperative Medium Access Control (MAC) techniques in Vehicular Ad Hoc Networks (VANETs) equipped with Roadside Unit (RSU) to enhance network throughput. Our method employs a distributed cooperative MAC scheme based on Carrier Sense Multiple Access with Collision Avoidance (CSMA/CA) protocol, featuring selective RSU probing and adaptive transmission. It utilizes a dual timescale channel access framework, with a ``large-scale'' phase accounting for gradual changes in vehicle locations and a ``small-scale'' phase adapting to rapid channel fluctuations. We propose the RSU Probing and Cooperative Access (RPCA) strategy, a two-stage approach based on dynamic inter-vehicle distances from the RSU. Using optimal sequential planned decision theory, we rigorously prove its optimality in maximizing average system throughput per large-scale phase. For practical implementation in VANETs, we develop a distributed MAC algorithm with periodic location updates. It adjusts thresholds based on inter-vehicle and vehicle-RSU distances during the large-scale phase and accesses channels following the RPCA strategy with updated thresholds during the small-scale phase. Simulation results confirm the effectiveness and efficiency of our algorithm.

Detection of Signals in Colored Noise: Leading Eigenvalue Test for Non-central $F$-matrices

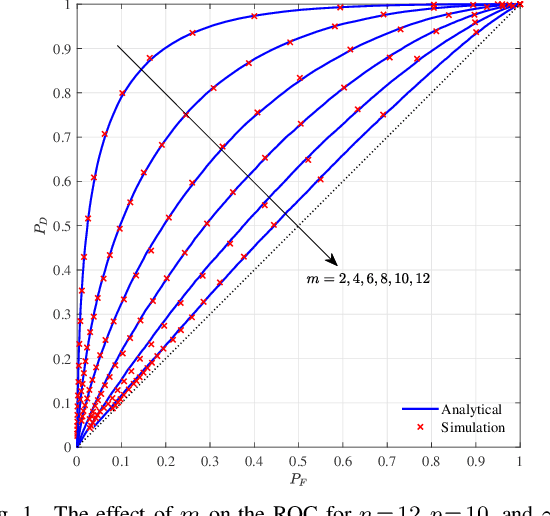
This paper investigates the signal detection problem in colored noise with an unknown covariance matrix. In particular, we focus on detecting an unknown non-random signal by capitalizing on the leading eigenvalue of the whitened sample covariance matrix as the test statistic (a.k.a. Roy's largest root test). Since the unknown signal is non-random, the whitened sample covariance matrix turns out to have a non-central $F$-distribution. This distribution assumes a singular or non-singular form depending on whether the number of observations $p\lessgtr$ the system dimensionality $m$. Therefore, we statistically characterize the leading eigenvalue of the singular and non-singular $F$-matrices by deriving their cumulative distribution functions (c.d.f.). Subsequently, they have been utilized in deriving the corresponding receiver operating characteristic (ROC) profiles. We also extend our analysis into the high dimensional domain. It turns out that, when the signal is sufficiently strong, the maximum eigenvalue can reliably detect it in this regime. Nevertheless, weak signals cannot be detected in the high dimensional regime with the leading eigenvalue.

Deep Learning Methods for Device Identification Using Symbols Trace Plot

Devices authentication is one crucial aspect of any communication system. Recently, the physical layer approach radio frequency (RF) fingerprinting has gained increased interest as it provides an extra layer of security without requiring additional components. In this work, we propose an RF fingerprinting based transmitter authentication approach density trace plot (DTP) to exploit device-identifiable fingerprints. By considering IQ imbalance solely as the feature source, DTP can efficiently extract device-identifiable fingerprints from symbol transition trajectories and density center drifts. In total, three DTP modalities based on constellation, eye and phase traces are respectively generated and tested against three deep learning classifiers: the 2D-CNN, 2D-CNN+biLSTM and 3D-CNN. The feasibility of these DTP and classifier pairs is verified using a practical dataset collected from the ADALM-PLUTO software-defined radios (SDRs).

Generalized Eigenvalue Based Detection of Signals in Colored Noise: A Sample Deficient Analysis

This paper investigates the signal detection problem in colored noise with an unknown covariance matrix. To be specific, we consider a scenario in which the number of signal bearing samples ($n$) is strictly smaller than the dimensionality of the signal space ($m$). Our test statistic is the leading generalized eigenvalue of the whitened sample covariance matrix (a.k.a. $F$-matrix) which is constructed by whitening the signal bearing sample covariance matrix with noise-only sample covariance matrix. The sample deficiency (i.e., $m>n$) in turn makes this $F$-matrix rank deficient, thereby singular. Therefore, an exact statistical characterization of the leading generalized eigenvalue (l.g.e.) of a singular $F$-matrix is of paramount importance to assess the performance of the detector (i.e., the receiver operating characteristics (ROC)). To this end, we employ the powerful orthogonal polynomial approach to derive a new finite dimensional c.d.f. expression for the l.g.e. of a singular $F$-matrix. It turns out that when the noise only sample covariance matrix is nearly rank deficient and the signal-to-noise ratio is $O(m)$, the ROC profile converges to a limit.