Ivana Palunko
Entanglement Definitions for Tethered Robots: Exploration and Analysis
Feb 07, 2024



Abstract:In this article we consider the problem of tether entanglement for tethered robots. In many applications, such as maintenance of underwater structures, aerial inspection, and underground exploration, tethered robots are often used in place of standalone (i.e., untethered) ones. However, the presence of a tether also introduces the risk for it to get entangled with obstacles present in the environment or with itself. To avoid these situations, a non-entanglement constraint can be considered in the motion planning problem for tethered robots. This constraint can be expressed either as a set of specific tether configurations that must be avoided, or as a quantitative measure of a `level of entanglement' that can be minimized. However, the literature lacks a generally accepted definition of entanglement, with existing definitions being limited and partial. Namely, the existing entanglement definitions either require a taut tether to come into contact with an obstacle or with another tether, or they require for the tether to do a full loop around an obstacle. In practice, this means that the existing definitions do not effectively cover all instances of tether entanglement. Our goal in this article is to bridge this gap and provide new definitions of entanglement, which, together with the existing ones, can be effectively used to qualify the entanglement state of a tethered robot in diverse situations. The new definitions find application mainly in motion planning for tethered robot systems, where they can be used to obtain more safe and robust entanglement-free trajectories. The present article focuses exclusively on the presentation and analysis of the entanglement definitions. The application of the definitions to the motion planning problem is left for future work.
Recovering the 3D UUV Position using UAV Imagery in Shallow-Water Environments
Jan 08, 2024Abstract:In this paper we propose a novel approach aimed at recovering the 3D position of an UUV from UAV imagery in shallow-water environments. Through combination of UAV and UUV measurements, we show that our method can be utilized as an accurate and cost-effective alternative when compared to acoustic sensing methods, typically required to obtain ground truth information in underwater localization problems. Furthermore, our approach allows for a seamless conversion to geo-referenced coordinates which can be utilized for navigation purposes. To validate our method, we present the results with data collected through a simulation environment and field experiments, demonstrating the ability to successfully recover the UUV position with sub-meter accuracy.
Two-Channel Extended Kalman Filtering with Intermittent Measurements
Dec 18, 2023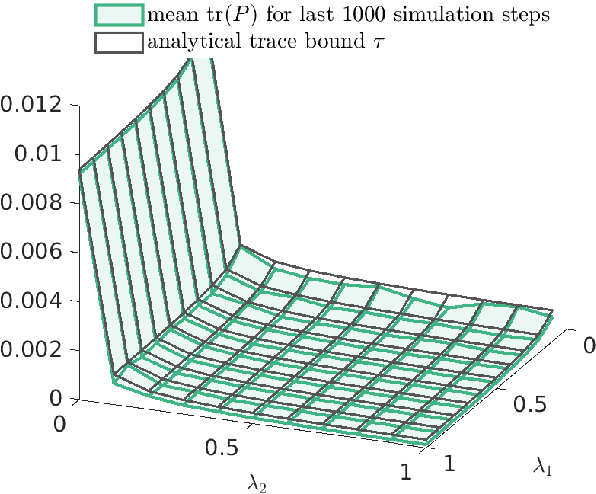
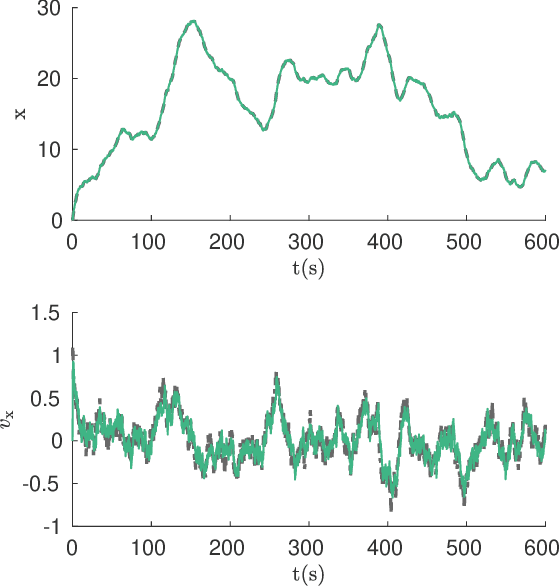
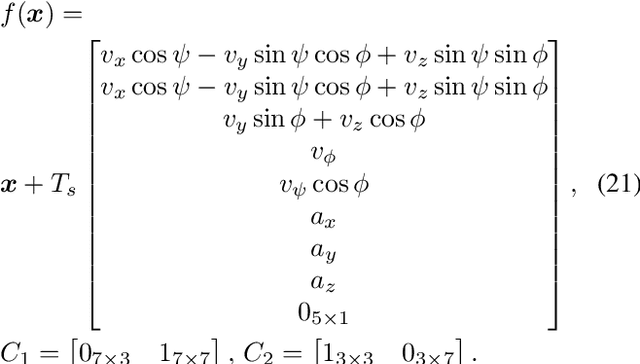
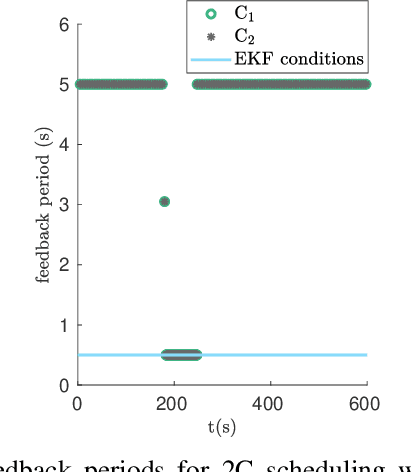
Abstract:We consider two nonlinear state estimation problems in a setting where an extended Kalman filter receives measurements from two sets of sensors via two channels (2C). In the stochastic-2C problem, the channels drop measurements stochastically, whereas in 2C scheduling, the estimator chooses when to read each channel. In the first problem, we generalize linear-case 2C analysis to obtain -- for a given pair of channel arrival rates -- boundedness conditions for the trace of the error covariance, as well as a worst-case upper bound. For scheduling, an optimization problem is solved to find arrival rates that balance low channel usage with low trace bounds, and channels are read deterministically with the expected periods corresponding to these arrival rates. We validate both solutions in simulations for linear and nonlinear dynamics; as well as in a real experiment with an underwater robot whose position is being intermittently found in a UAV camera image.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge