Dynamic metastability in the self-attention model

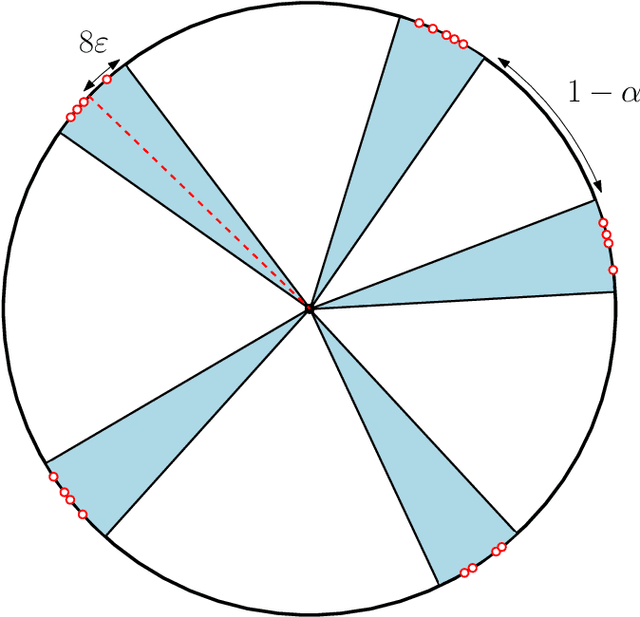
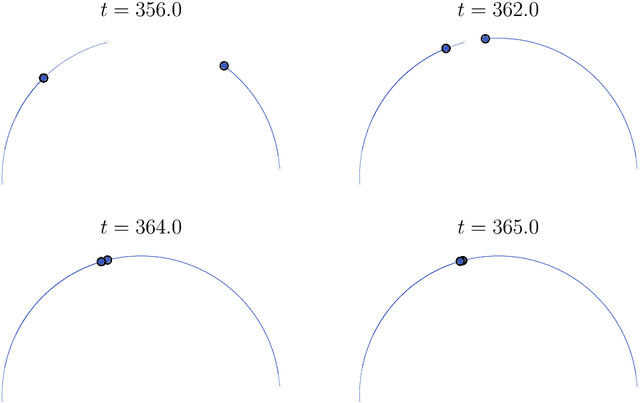
We consider the self-attention model - an interacting particle system on the unit sphere, which serves as a toy model for Transformers, the deep neural network architecture behind the recent successes of large language models. We prove the appearance of dynamic metastability conjectured in [GLPR23] - although particles collapse to a single cluster in infinite time, they remain trapped near a configuration of several clusters for an exponentially long period of time. By leveraging a gradient flow interpretation of the system, we also connect our result to an overarching framework of slow motion of gradient flows proposed by Otto and Reznikoff [OR07] in the context of coarsening and the Allen-Cahn equation. We finally probe the dynamics beyond the exponentially long period of metastability, and illustrate that, under an appropriate time-rescaling, the energy reaches its global maximum in finite time and has a staircase profile, with trajectories manifesting saddle-to-saddle-like behavior, reminiscent of recent works in the analysis of training dynamics via gradient descent for two-layer neural networks.

The Impact of LoRA on the Emergence of Clusters in Transformers

In this paper, we employ the mathematical framework on Transformers developed by \citet{sander2022sinkformers,geshkovski2023emergence,geshkovski2023mathematical} to explore how variations in attention parameters and initial token values impact the structural dynamics of token clusters. Our analysis demonstrates that while the clusters within a modified attention matrix dynamics can exhibit significant divergence from the original over extended periods, they maintain close similarities over shorter intervals, depending on the parameter differences. This work contributes to the fine-tuning field through practical applications to the LoRA algorithm \cite{hu2021lora,peft}, enhancing our understanding of the behavior of LoRA-enhanced Transformer models.