On the Problem of Best Arm Retention

This paper presents a comprehensive study on the problem of Best Arm Retention (BAR), which has recently found applications in streaming algorithms for multi-armed bandits. In the BAR problem, the goal is to retain $m$ arms with the best arm included from $n$ after some trials, in stochastic multi-armed bandit settings. We first investigate pure exploration for the BAR problem under different criteria, and then minimize the regret with specific constraints, in the context of further exploration in streaming algorithms. - We begin by revisiting the lower bound for the $(\varepsilon,\delta)$-PAC algorithm for Best Arm Identification (BAI) and adapt the classical KL-divergence argument to derive optimal bounds for $(\varepsilon,\delta)$-PAC algorithms for BAR. - We further study another variant of the problem, called $r$-BAR, which requires the expected gap between the best arm and the optimal arm retained is less than $r$. We prove tight sample complexity for the problem. - We explore the regret minimization problem for $r$-BAR and develop algorithm beyond pure exploration. We conclude with a conjecture on the optimal regret in this setting.

Tight Regret Bounds for Fixed-Price Bilateral Trade

We examine fixed-price mechanisms in bilateral trade through the lens of regret minimization. Our main results are twofold. (i) For independent values, a near-optimal $\widetilde{\Theta}(T^{2/3})$ tight bound for $\textsf{Global Budget Balance}$ fixed-price mechanisms with two-bit/one-bit feedback. (ii) For correlated/adversarial values, a near-optimal $\Omega(T^{3/4})$ lower bound for $\textsf{Global Budget Balance}$ fixed-price mechanisms with two-bit/one-bit feedback, which improves the best known $\Omega(T^{5/7})$ lower bound obtained in the work \cite{BCCF24} and, up to polylogarithmic factors, matches the $\widetilde{\mathcal{O}}(T^{3 / 4})$ upper bound obtained in the same work. Our work in combination with the previous works \cite{CCCFL24mor, CCCFL24jmlr, AFF24, BCCF24} (essentially) gives a thorough understanding of regret minimization for fixed-price bilateral trade. En route, we have developed two technical ingredients that might be of independent interest: (i) A novel algorithmic paradigm, called $\textit{{fractal elimination}}$, to address one-bit feedback and independent values. (ii) A new $\textit{lower-bound construction}$ with novel proof techniques, to address the $\textsf{Global Budget Balance}$ constraint and correlated values.

On Interpolating Experts and Multi-Armed Bandits

Learning with expert advice and multi-armed bandit are two classic online decision problems which differ on how the information is observed in each round of the game. We study a family of problems interpolating the two. For a vector $\mathbf{m}=(m_1,\dots,m_K)\in \mathbb{N}^K$, an instance of $\mathbf{m}$-MAB indicates that the arms are partitioned into $K$ groups and the $i$-th group contains $m_i$ arms. Once an arm is pulled, the losses of all arms in the same group are observed. We prove tight minimax regret bounds for $\mathbf{m}$-MAB and design an optimal PAC algorithm for its pure exploration version, $\mathbf{m}$-BAI, where the goal is to identify the arm with minimum loss with as few rounds as possible. We show that the minimax regret of $\mathbf{m}$-MAB is $\Theta\left(\sqrt{T\sum_{k=1}^K\log (m_k+1)}\right)$ and the minimum number of pulls for an $(\epsilon,0.05)$-PAC algorithm of $\mathbf{m}$-BAI is $\Theta\left(\frac{1}{\epsilon^2}\cdot \sum_{k=1}^K\log (m_k+1)\right)$. Both our upper bounds and lower bounds for $\mathbf{m}$-MAB can be extended to a more general setting, namely the bandit with graph feedback, in terms of the clique cover and related graph parameters. As consequences, we obtained tight minimax regret bounds for several families of feedback graphs.

Understanding Bandits with Graph Feedback

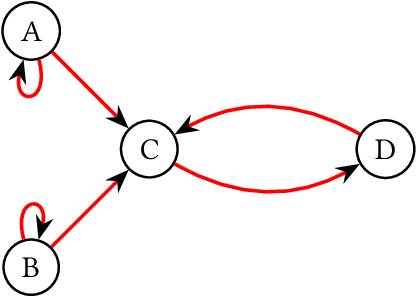
The bandit problem with graph feedback, proposed in [Mannor and Shamir, NeurIPS 2011], is modeled by a directed graph $G=(V,E)$ where $V$ is the collection of bandit arms, and once an arm is triggered, all its incident arms are observed. A fundamental question is how the structure of the graph affects the min-max regret. We propose the notions of the fractional weak domination number $\delta^*$ and the $k$-packing independence number capturing upper bound and lower bound for the regret respectively. We show that the two notions are inherently connected via aligning them with the linear program of the weakly dominating set and its dual -- the fractional vertex packing set respectively. Based on this connection, we utilize the strong duality theorem to prove a general regret upper bound $O\left(\left( \delta^*\log |V|\right)^{\frac{1}{3}}T^{\frac{2}{3}}\right)$ and a lower bound $\Omega\left(\left(\delta^*/\alpha\right)^{\frac{1}{3}}T^{\frac{2}{3}}\right)$ where $\alpha$ is the integrality gap of the dual linear program. Therefore, our bounds are tight up to a $\left(\log |V|\right)^{\frac{1}{3}}$ factor on graphs with bounded integrality gap for the vertex packing problem including trees and graphs with bounded degree. Moreover, we show that for several special families of graphs, we can get rid of the $\left(\log |V|\right)^{\frac{1}{3}}$ factor and establish optimal regret.