The geometry of BERT

Transformer neural networks, particularly Bidirectional Encoder Representations from Transformers (BERT), have shown remarkable performance across various tasks such as classification, text summarization, and question answering. However, their internal mechanisms remain mathematically obscure, highlighting the need for greater explainability and interpretability. In this direction, this paper investigates the internal mechanisms of BERT proposing a novel perspective on the attention mechanism of BERT from a theoretical perspective. The analysis encompasses both local and global network behavior. At the local level, the concept of directionality of subspace selection as well as a comprehensive study of the patterns emerging from the self-attention matrix are presented. Additionally, this work explores the semantic content of the information stream through data distribution analysis and global statistical measures including the novel concept of cone index. A case study on the classification of SARS-CoV-2 variants using RNA which resulted in a very high accuracy has been selected in order to observe these concepts in an application. The insights gained from this analysis contribute to a deeper understanding of BERT's classification process, offering potential avenues for future architectural improvements in Transformer models and further analysis in the training process.

Vision Transformers for femur fracture classification

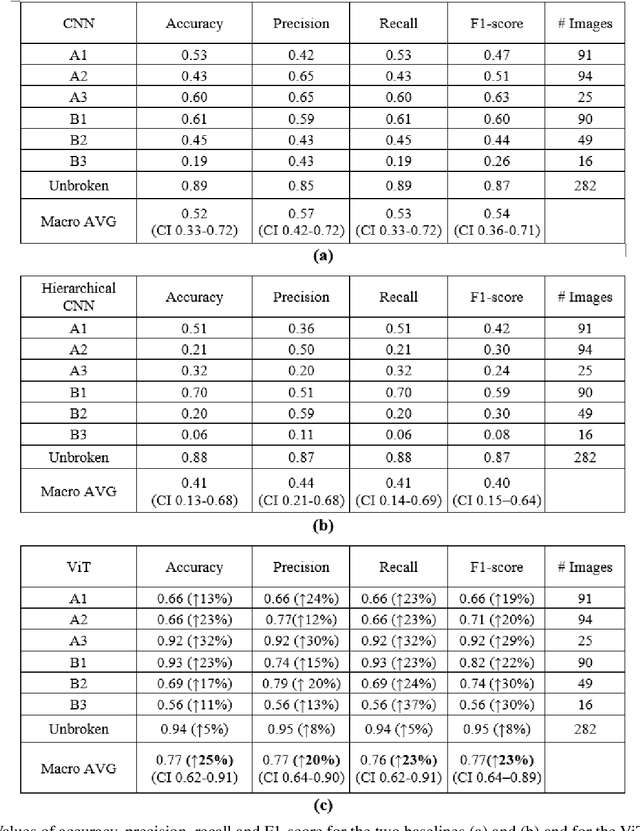
Objectives: In recent years, the scientific community has focused on the development of Computer-Aided Diagnosis (CAD) tools that could improve bone fractures' classification. However, the results of the classification of fractures in subtypes with the proposed datasets were far from optimal. This paper proposes a very recent and outperforming deep learning technique, the Vision Transformer (ViT), in order to improve the fracture classification, by exploiting its self-attention mechanism. Methods: 4207 manually annotated images were used and distributed, by following the AO/OTA classification, in different fracture types, the largest labeled dataset of proximal femur fractures used in literature. The ViT architecture was used and compared with a classic Convolutional Neural Network (CNN) and a multistage architecture composed by successive CNNs in cascade. To demonstrate the reliability of this approach, 1) the attention maps were used to visualize the most relevant areas of the images, 2) the performance of a generic CNN and ViT was also compared through unsupervised learning techniques, and 3) 11 specialists were asked to evaluate and classify 150 proximal femur fractures' images with and without the help of the ViT. Results: The ViT was able to correctly predict 83% of the test images. Precision, recall and F1-score were 0.77 (CI 0.64-0.90), 0.76 (CI 0.62-0.91) and 0.77 (CI 0.64-0.89), respectively. The average specialists' diagnostic improvement was 29%. Conclusions: This paper showed the potential of Transformers in bone fracture classification. For the first time, good results were obtained in sub-fractures with the largest and richest dataset ever.

Gradient-based Competitive Learning: Theory

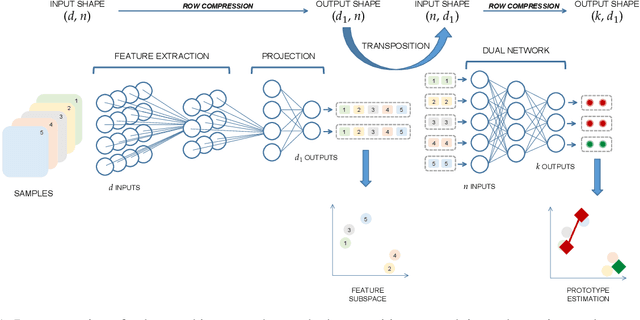
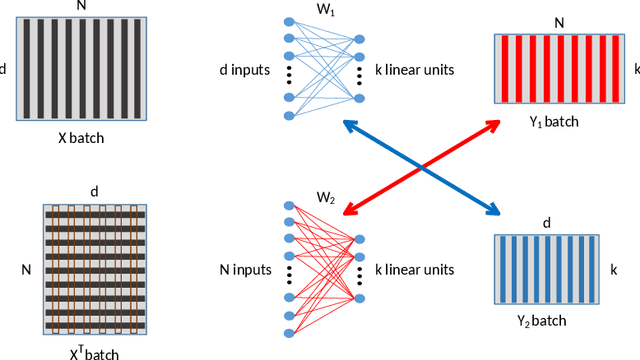
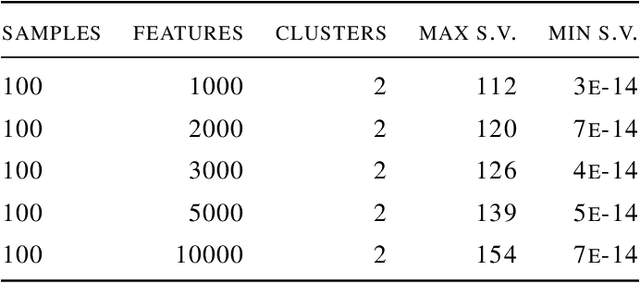
Deep learning has been widely used for supervised learning and classification/regression problems. Recently, a novel area of research has applied this paradigm to unsupervised tasks; indeed, a gradient-based approach extracts, efficiently and autonomously, the relevant features for handling input data. However, state-of-the-art techniques focus mostly on algorithmic efficiency and accuracy rather than mimic the input manifold. On the contrary, competitive learning is a powerful tool for replicating the input distribution topology. This paper introduces a novel perspective in this area by combining these two techniques: unsupervised gradient-based and competitive learning. The theory is based on the intuition that neural networks are able to learn topological structures by working directly on the transpose of the input matrix. At this purpose, the vanilla competitive layer and its dual are presented. The former is just an adaptation of a standard competitive layer for deep clustering, while the latter is trained on the transposed matrix. Their equivalence is extensively proven both theoretically and experimentally. However, the dual layer is better suited for handling very high-dimensional datasets. The proposed approach has a great potential as it can be generalized to a vast selection of topological learning tasks, such as non-stationary and hierarchical clustering; furthermore, it can also be integrated within more complex architectures such as autoencoders and generative adversarial networks.

Topological Gradient-based Competitive Learning

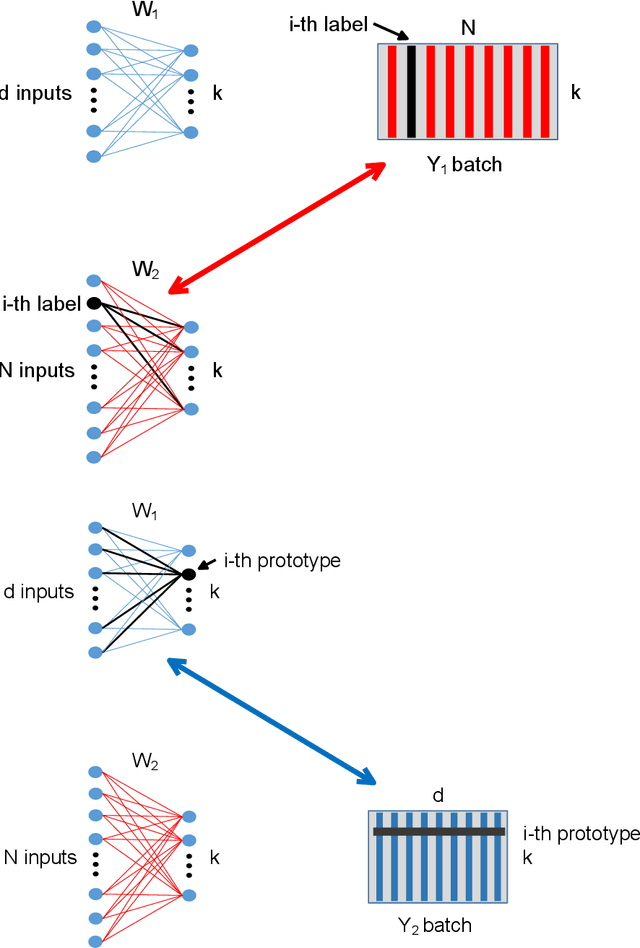
Topological learning is a wide research area aiming at uncovering the mutual spatial relationships between the elements of a set. Some of the most common and oldest approaches involve the use of unsupervised competitive neural networks. However, these methods are not based on gradient optimization which has been proven to provide striking results in feature extraction also in unsupervised learning. Unfortunately, by focusing mostly on algorithmic efficiency and accuracy, deep clustering techniques are composed of overly complex feature extractors, while using trivial algorithms in their top layer. The aim of this work is to present a novel comprehensive theory aspiring at bridging competitive learning with gradient-based learning, thus allowing the use of extremely powerful deep neural networks for feature extraction and projection combined with the remarkable flexibility and expressiveness of competitive learning. In this paper we fully demonstrate the theoretical equivalence of two novel gradient-based competitive layers. Preliminary experiments show how the dual approach, trained on the transpose of the input matrix i.e. $X^T$, lead to faster convergence rate and higher training accuracy both in low and high-dimensional scenarios.