Conditioning and AGM-like belief change in the Desirability-Indifference framework

We show how the AGM framework for belief change (expansion, revision, contraction) can be extended to deal with conditioning in the so-called Desirability-Indifference framework, based on abstract notions of accepting and rejecting options, as well as on abstract notions of events. This level of abstraction allows us to deal simultaneously with classical and quantum probability theory.

Conditioning through indifference in quantum mechanics

We can learn (more) about the state a quantum system is in through measurements. We look at how to describe the uncertainty about a quantum system's state conditional on executing such measurements. We show that by exploiting the interplay between desirability, coherence and indifference, a general rule for conditioning can be derived. We then apply this rule to conditioning on measurement outcomes, and show how it generalises to conditioning on a set of measurement outcomes.

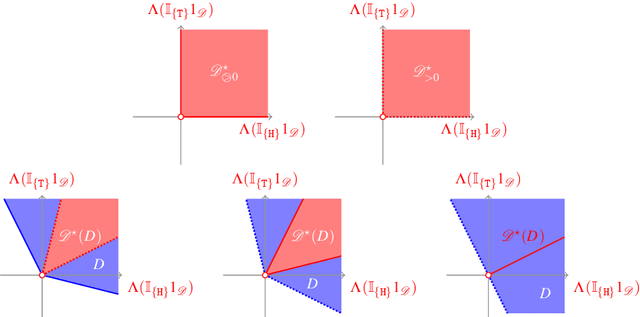
The logic behind desirable sets of things, and its filter representation

We identify the logic behind the recent theory of coherent sets of desirable (sets of) things, which generalise desirable (sets of) gambles and coherent choice functions, and show that this identification allows us to establish various representation results for such coherent models in terms of simpler ones.

Decision-making with E-admissibility given a finite assessment of choices

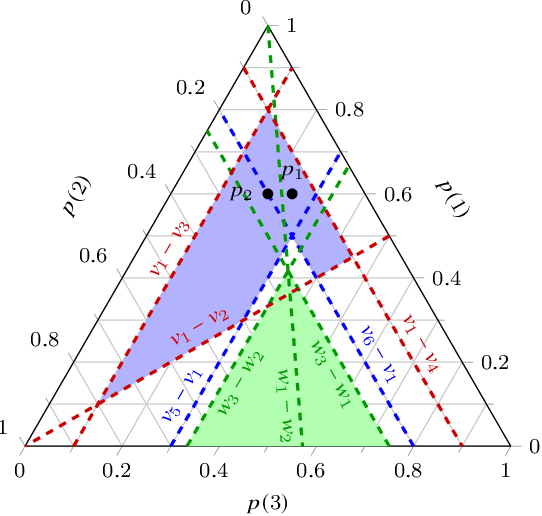
Given information about which options a decision-maker definitely rejects from given finite sets of options, we study the implications for decision-making with E-admissibility. This means that from any finite set of options, we reject those options that no probability mass function compatible with the given information gives the highest expected utility. We use the mathematical framework of choice functions to specify choices and rejections, and specify the available information in the form of conditions on such functions. We characterise the most conservative extension of the given information to a choice function that makes choices based on E-admissibility, and provide an algorithm that computes this extension by solving linear feasibility problems.

On a notion of independence proposed by Teddy Seidenfeld

Teddy Seidenfeld has been arguing for quite a long time that binary preference models are not powerful enough to deal with a number of crucial aspects of imprecision and indeterminacy in uncertain inference and decision making. It is at his insistence that we initiated our study of so-called sets of desirable option sets, which we have argued elsewhere provides an elegant and powerful approach to dealing with general, binary as well as non-binary, decision-making under uncertainty. We use this approach here to explore an interesting notion of irrelevance (and independence), first suggested by Seidenfeld in an example intended as a criticism of a number of specific decision methodologies based on (convex) binary preferences. We show that the consequences of making such an irrelevance or independence assessment are very strong, and might be used to argue for the use of so-called mixing choice functions, and E-admissibility as the resulting decision scheme.

Inference with Choice Functions Made Practical

We study how to infer new choices from previous choices in a conservative manner. To make such inferences, we use the theory of choice functions: a unifying mathematical framework for conservative decision making that allows one to impose axioms directly on the represented decisions. We here adopt the coherence axioms of De Bock and De Cooman (2019). We show how to naturally extend any given choice assessment to such a coherent choice function, whenever possible, and use this natural extension to make new choices. We present a practical algorithm to compute this natural extension and provide several methods that can be used to improve its scalability.

Coherent and Archimedean choice in general Banach spaces

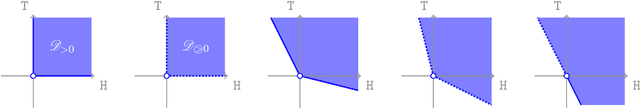
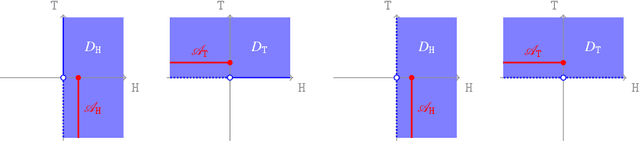
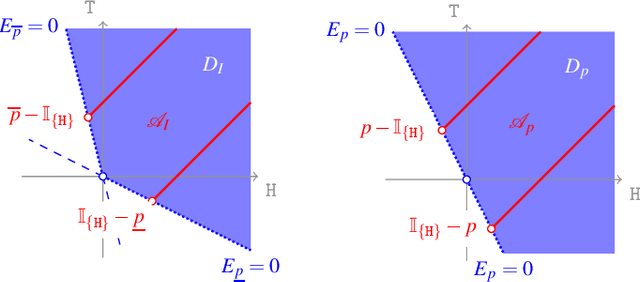
I introduce and study a new notion of Archimedeanity for binary and non-binary choice between options that live in an abstract Banach space, through a very general class of choice models, called sets of desirable option sets. In order to be able to bring horse lottery options into the fold, I pay special attention to the case where these linear spaces do not include all `constant' options. I consider the frameworks of conservative inference associated with Archimedean (and coherent) choice models, and also pay quite a lot of attention to representation of general (non-binary) choice models in terms of the simpler, binary ones. The representation theorems proved here provide an axiomatic characterisation of, amongst other choice methods, Levi's E-admissibility and Walley--Sen maximality.

An alternative approach to coherent choice functions

Choice functions constitute a simple, direct and very general mathematical framework for modelling choice under uncertainty. In particular, they are able to represent the set-valued choices that appear in imprecise-probabilistic decision making. We provide these choice functions with a clear interpretation in terms of desirability, use this interpretation to derive a set of basic coherence axioms, and show that this notion of coherence leads to a representation in terms of sets of strict preference orders. By imposing additional properties such as totality, the mixing property and Archimedeanity, we obtain representation in terms of sets of strict total orders, lexicographic probability systems, coherent lower previsions or linear previsions.

A Desirability-Based Axiomatisation for Coherent Choice Functions

Choice functions constitute a simple, direct and very general mathematical framework for modelling choice under uncertainty. In particular, they are able to represent the set-valued choices that typically arise from applying decision rules to imprecise-probabilistic uncertainty models. We provide them with a clear interpretation in terms of attitudes towards gambling, borrowing ideas from the theory of sets of desirable gambles, and we use this interpretation to derive a set of basic axioms. We show that these axioms lead to a full-fledged theory of coherent choice functions, which includes a representation in terms of sets of desirable gambles, and a conservative inference method.

Lexicographic choice functions

We investigate a generalisation of the coherent choice functions considered by Seidenfeld et al. (2010), by sticking to the convexity axiom but imposing no Archimedeanity condition. We define our choice functions on vector spaces of options, which allows us to incorporate as special cases both Seidenfeld et al.'s (2010) choice functions on horse lotteries and sets of desirable gambles (Quaeghebeur, 2014), and to investigate their connections. We show that choice functions based on sets of desirable options (gambles) satisfy Seidenfeld's convexity axiom only for very particular types of sets of desirable options, which are in a one-to-one relationship with the lexicographic probabilities. We call them lexicographic choice functions. Finally, we prove that these choice functions can be used to determine the most conservative convex choice function associated with a given binary relation.