Optimally Deceiving a Learning Leader in Stackelberg Games

Recent results in the ML community have revealed that learning algorithms used to compute the optimal strategy for the leader to commit to in a Stackelberg game, are susceptible to manipulation by the follower. Such a learning algorithm operates by querying the best responses or the payoffs of the follower, who consequently can deceive the algorithm by responding as if his payoffs were much different than what they actually are. For this strategic behavior to be successful, the main challenge faced by the follower is to pinpoint the payoffs that would make the learning algorithm compute a commitment so that best responding to it maximizes the follower's utility, according to his true payoffs. While this problem has been considered before, the related literature only focused on the simplified scenario in which the payoff space is finite, thus leaving the general version of the problem unanswered. In this paper, we fill in this gap, by showing that it is always possible for the follower to compute (near-)optimal payoffs for various scenarios about the learning interaction between leader and follower.

Learning Convex Partitions and Computing Game-theoretic Equilibria from Best Response Queries

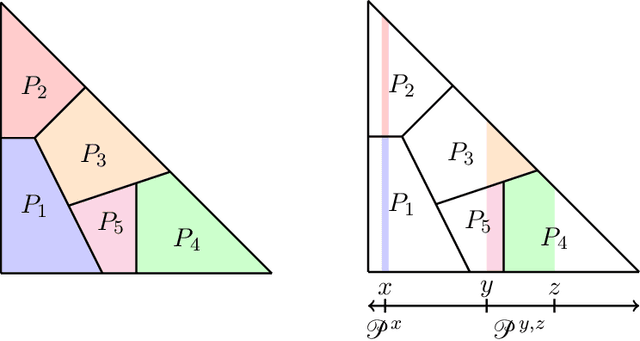
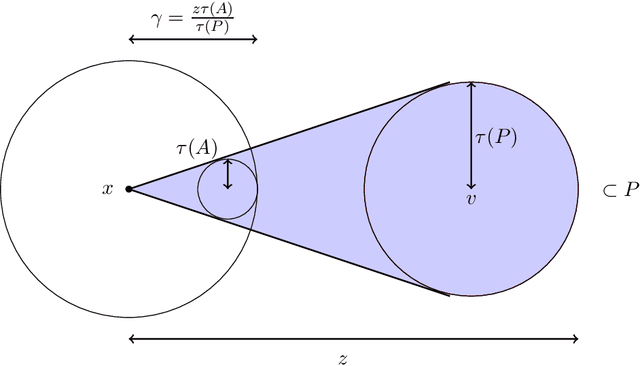
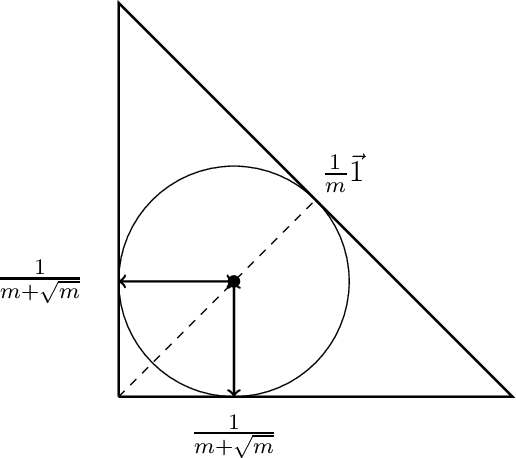
Suppose that an $m$-simplex is partitioned into $n$ convex regions having disjoint interiors and distinct labels, and we may learn the label of any point by querying it. The learning objective is to know, for any point in the simplex, a label that occurs within some distance $\epsilon$ from that point. We present two algorithms for this task: Constant-Dimension Generalised Binary Search (CD-GBS), which for constant $m$ uses $poly(n, \log \left( \frac{1}{\epsilon} \right))$ queries, and Constant-Region Generalised Binary Search (CR-GBS), which uses CD-GBS as a subroutine and for constant $n$ uses $poly(m, \log \left( \frac{1}{\epsilon} \right))$ queries. We show via Kakutani's fixed-point theorem that these algorithms provide bounds on the best-response query complexity of computing approximate well-supported equilibria of bimatrix games in which one of the players has a constant number of pure strategies. We also partially extend our results to games with multiple players, establishing further query complexity bounds for computing approximate well-supported equilibria in this setting.