Improving 3D Cellular Positioning Integrity with Bayesian RAIM

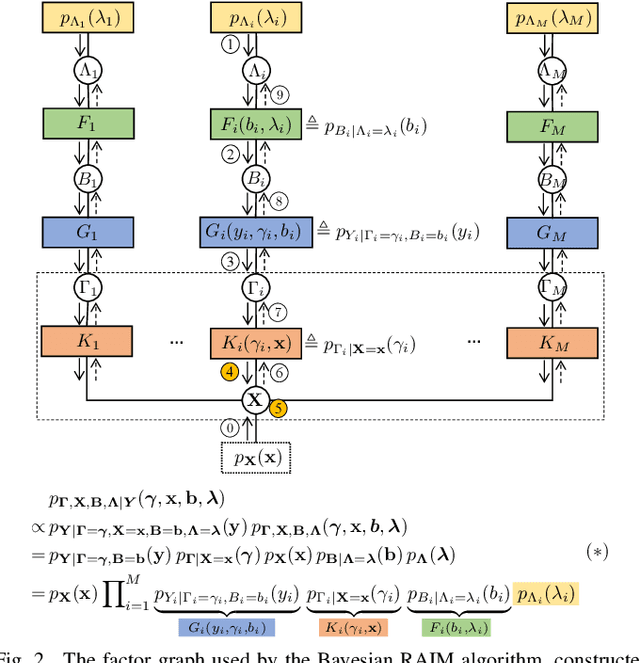
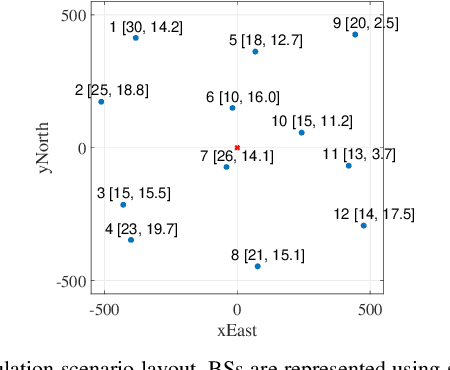
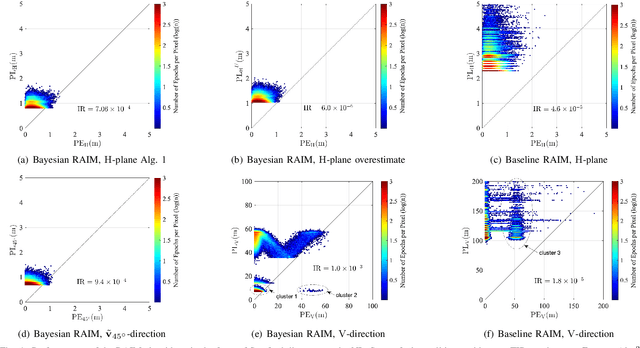
Ensuring positioning integrity amid faulty measurements is crucial for safety-critical applications, making receiver autonomous integrity monitoring (RAIM) indispensable. This paper introduces a Bayesian RAIM algorithm with a streamlined architecture for snapshot-type 3D cellular positioning. Unlike traditional frequentist-type RAIM algorithms, it computes the exact posterior probability density function (PDF) of the position vector as a Gaussian mixture (GM) model using efficient message passing along a factor graph. This Bayesian approach retains all crucial information from the measurements, eliminates the need to discard faulty measurements, and results in tighter protection levels (PLs) in 3D space and 1D/2D subspaces that meet target integrity risk (TIR) requirements. Numerical simulations demonstrate that the Bayesian RAIM algorithm significantly outperforms a baseline algorithm, achieving over $50\%$ PL reduction at a comparable computational cost.

Bayesian Integrity Monitoring for Cellular Positioning -- A Simplified Case Study

Bayesian receiver autonomous integrity monitoring (RAIM) algorithms are developed for the snapshot cellular positioning problem in a simplified one-dimensional (1D) linear Gaussian setting. Position estimation, multi-fault detection and exclusion, and protection level (PL) computation are enabled by the efficient and exact computation of the position posterior probabilities via message passing along a factor graph. Computer simulations demonstrate the significant performance improvement of the proposed Bayesian RAIM algorithms over a baseline advanced RAIM algorithm, as it obtains tighter PLs that meet the target integrity risk (TIR) requirements.