Classification of the Fashion-MNIST Dataset on a Quantum Computer

The potential impact of quantum machine learning algorithms on industrial applications remains an exciting open question. Conventional methods for encoding classical data into quantum computers are not only too costly for a potential quantum advantage in the algorithms but also severely limit the scale of feasible experiments on current hardware. Therefore, recent works, despite claiming the near-term suitability of their algorithms, do not provide experimental benchmarking on standard machine learning datasets. We attempt to solve the data encoding problem by improving a recently proposed variational algorithm [1] that approximately prepares the encoded data, using asymptotically shallow circuits that fit the native gate set and topology of currently available quantum computers. We apply the improved algorithm to encode the Fashion-MNIST dataset [2], which can be directly used in future empirical studies of quantum machine learning algorithms. We deploy simple quantum variational classifiers trained on the encoded dataset on a current quantum computer ibmq-kolkata [3] and achieve moderate accuracies, providing a proof of concept for the near-term usability of our data encoding method.

Let Quantum Neural Networks Choose Their Own Frequencies

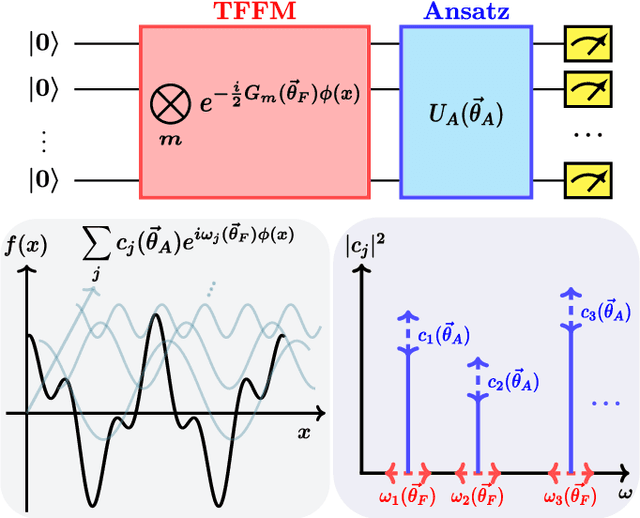
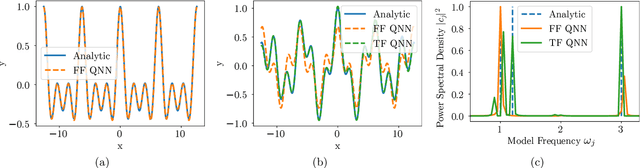
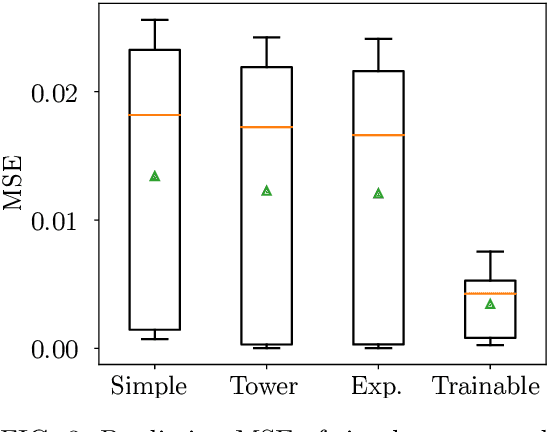
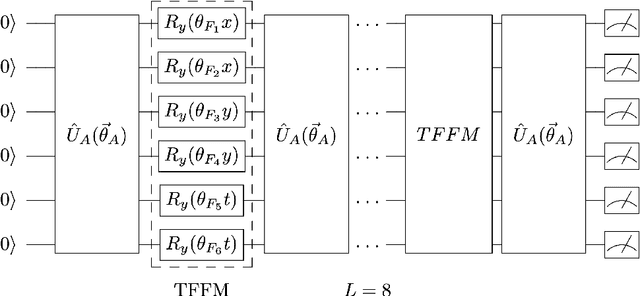
Parameterized quantum circuits as machine learning models are typically well described by their representation as a partial Fourier series of the input features, with frequencies uniquely determined by the feature map's generator Hamiltonians. Ordinarily, these data-encoding generators are chosen in advance, fixing the space of functions that can be represented. In this work we consider a generalization of quantum models to include a set of trainable parameters in the generator, leading to a trainable frequency (TF) quantum model. We numerically demonstrate how TF models can learn generators with desirable properties for solving the task at hand, including non-regularly spaced frequencies in their spectra and flexible spectral richness. Finally, we showcase the real-world effectiveness of our approach, demonstrating an improved accuracy in solving the Navier-Stokes equations using a TF model with only a single parameter added to each encoding operation. Since TF models encompass conventional fixed frequency models, they may offer a sensible default choice for variational quantum machine learning.