Distributed Combinatorial Optimization of Downlink User Assignment in mmWave Cell-free Massive MIMO Using Graph Neural Networks

Millimeter wave (mmWave) cell-free massive MIMO (CF mMIMO) is a promising solution for future wireless communications. However, its optimization is non-trivial due to the challenging channel characteristics. We show that mmWave CF mMIMO optimization is largely an assignment problem between access points (APs) and users due to the high path loss of mmWave channels, the limited output power of the amplifier, and the almost orthogonal channels between users given a large number of AP antennas. The combinatorial nature of the assignment problem, the requirement for scalability, and the distributed implementation of CF mMIMO make this problem difficult. In this work, we propose an unsupervised machine learning (ML) enabled solution. In particular, a graph neural network (GNN) customized for scalability and distributed implementation is introduced. Moreover, the customized GNN architecture is hierarchically permutation-equivariant (HPE), i.e., if the APs or users of an AP are permuted, the output assignment is automatically permuted in the same way. To address the combinatorial problem, we relax it to a continuous problem, and introduce an information entropy-inspired penalty term. The training objective is then formulated using the augmented Lagrangian method (ALM). The test results show that the realized sum-rate outperforms that of the generalized serial dictatorship (GSD) algorithm and is very close to the upper bound in a small network scenario, while the upper bound is impossible to obtain in a large network scenario.

Robust Energy-Efficient Resource Management, SIC Ordering, and Beamforming Design for MC MISO-NOMA Enabled 6G

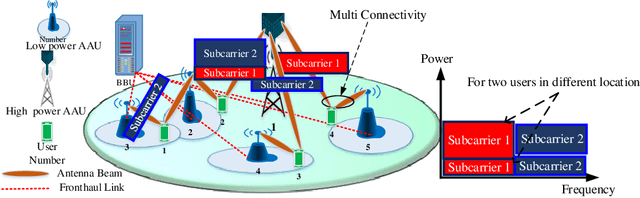
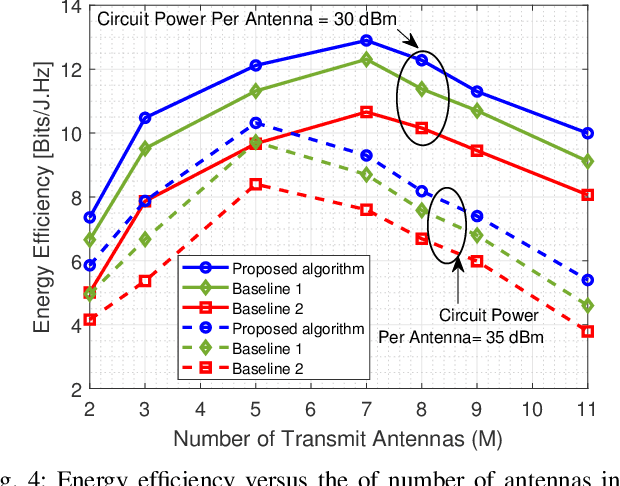
This paper studies a novel approach for successive interference cancellation (SIC) ordering and beamforming in a multiple antennas non-orthogonal multiple access (NOMA) network with multi-carrier multi-user setup. To this end, we formulate a joint beamforming design, subcarrier allocation, user association, and SIC ordering algorithm to maximize the worst-case energy efficiency (EE). The formulated problem is a non-convex mixed integer non-linear programming (MINLP) which is generally difficult to solve. To handle it, we first adopt the linearizion technique as well as relaxing the integer variables, and then we employ the Dinkelbach algorithm to convert it into a more mathematically tractable form. The adopted non-convex optimization problem is transformed into an equivalent rank-constrained semidefinite programming (SDP) and is solved by SDP relaxation and exploiting sequential fractional programming. Furthermore, to strike a balance between complexity and performance, a low complex approach based on alternative optimization is adopted. Numerical results unveil that the proposed SIC ordering method outperforms the conventional existing works addressed in the literature.