Exploring the Potential of Hybrid Machine-Learning/Physics-Based Modeling for Atmospheric/Oceanic Prediction Beyond the Medium Range

This paper explores the potential of a hybrid modeling approach that combines machine learning (ML) with conventional physics-based modeling for weather prediction beyond the medium range. It extends the work of Arcomano et al. (2022), which tested the approach for short- and medium-range weather prediction, and the work of Arcomano et al. (2023), which investigated its potential for climate modeling. The hybrid model used for the forecast experiments of the paper is based on the low-resolution, simplified parameterization atmospheric general circulation model (AGCM) SPEEDY. In addition to the hybridized prognostic variables of SPEEDY, the current version of the model has three purely ML-based prognostic variables. One of these is 6~h cumulative precipitation, another is the sea surface temperature, while the third is the heat content of the top 300 m deep layer of the ocean. The model has skill in predicting the El Ni\~no cycle and its global teleconnections with precipitation for 3-7 months depending on the season. The model captures equatorial variability of the precipitation associated with Kelvin and Rossby waves and MJO. Predictions of the precipitation in the equatorial region have skill for 15 days in the East Pacific and 11.5 days in the West Pacific. Though the model has low spatial resolution, for these tasks it has prediction skill comparable to what has been published for high-resolution, purely physics-based, conventional operational forecast models.

Attention-Based Ensemble Pooling for Time Series Forecasting

A common technique to reduce model bias in time-series forecasting is to use an ensemble of predictive models and pool their output into an ensemble forecast. In cases where each predictive model has different biases, however, it is not always clear exactly how each model forecast should be weighed during this pooling. We propose a method for pooling that performs a weighted average over candidate model forecasts, where the weights are learned by an attention-based ensemble pooling model. We test this method on two time-series forecasting problems: multi-step forecasting of the dynamics of the non-stationary Lorenz `63 equation, and one-step forecasting of the weekly incident deaths due to COVID-19. We find that while our model achieves excellent valid times when forecasting the non-stationary Lorenz `63 equation, it does not consistently perform better than the existing ensemble pooling when forecasting COVID-19 weekly incident deaths.

Using Machine Learning to Anticipate Tipping Points and Extrapolate to Post-Tipping Dynamics of Non-Stationary Dynamical Systems

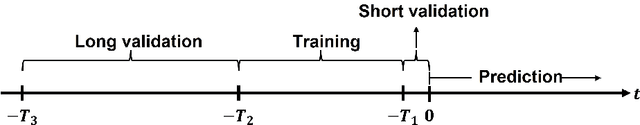
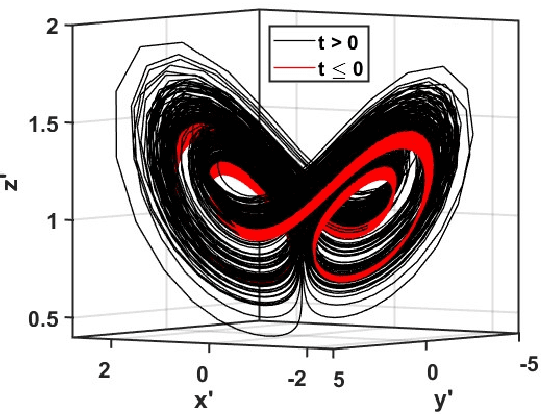
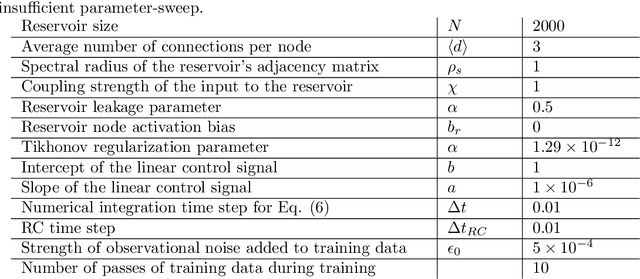
In this paper we consider the machine learning (ML) task of predicting tipping point transitions and long-term post-tipping-point behavior associated with the time evolution of an unknown (or partially unknown), non-stationary, potentially noisy and chaotic, dynamical system. We focus on the particularly challenging situation where the past dynamical state time series that is available for ML training predominantly lies in a restricted region of the state space, while the behavior to be predicted evolves on a larger state space set not fully observed by the ML model during training. In this situation, it is required that the ML prediction system have the ability to extrapolate to different dynamics past that which is observed during training. We investigate the extent to which ML methods are capable of accomplishing useful results for this task, as well as conditions under which they fail. In general, we found that the ML methods were surprisingly effective even in situations that were extremely challenging, but do (as one would expect) fail when ``too much" extrapolation is required. For the latter case, we investigate the effectiveness of combining the ML approach with conventional modeling based on scientific knowledge, thus forming a hybrid prediction system which we find can enable useful prediction even when its ML-based and knowledge-based components fail when acting alone. We also found that achieving useful results may require using very carefully selected ML hyperparameters and we propose a hyperparameter optimization strategy to address this problem. The main conclusion of this paper is that ML-based approaches are promising tools for predicting the behavior of non-stationary dynamical systems even in the case where the future evolution (perhaps due to the crossing of a tipping point) includes dynamics on a set outside of that explored by the training data.