LG-LSQ: Learned Gradient Linear Symmetric Quantization

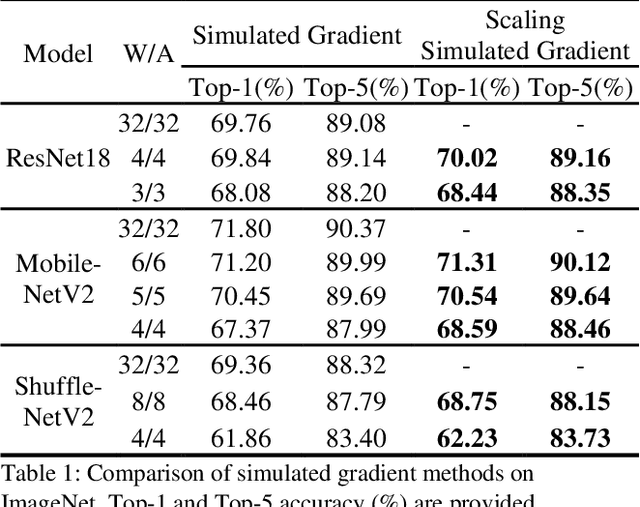
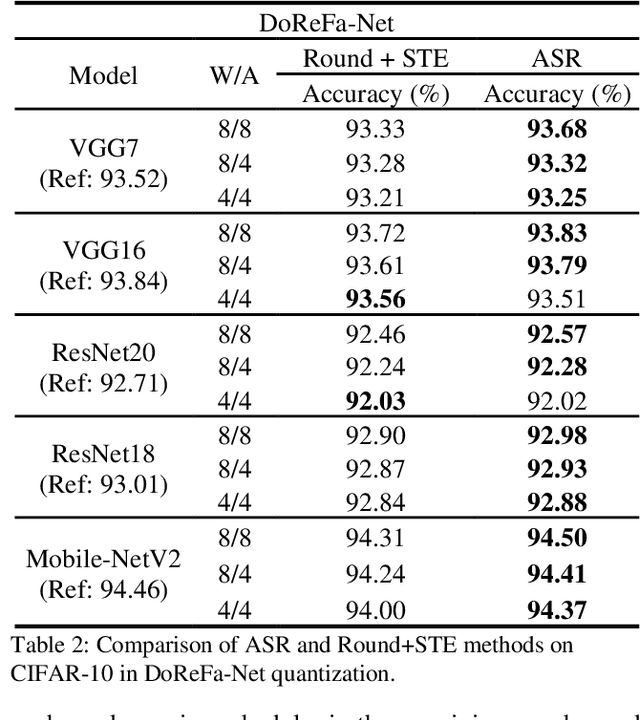
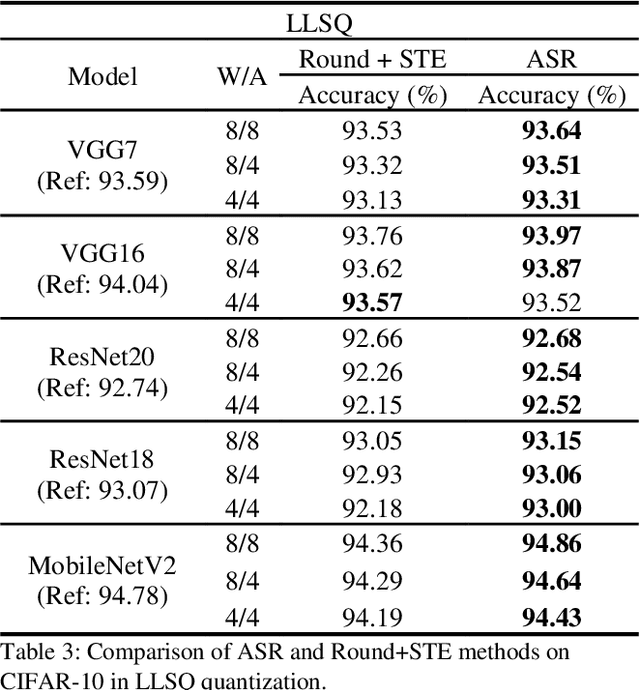
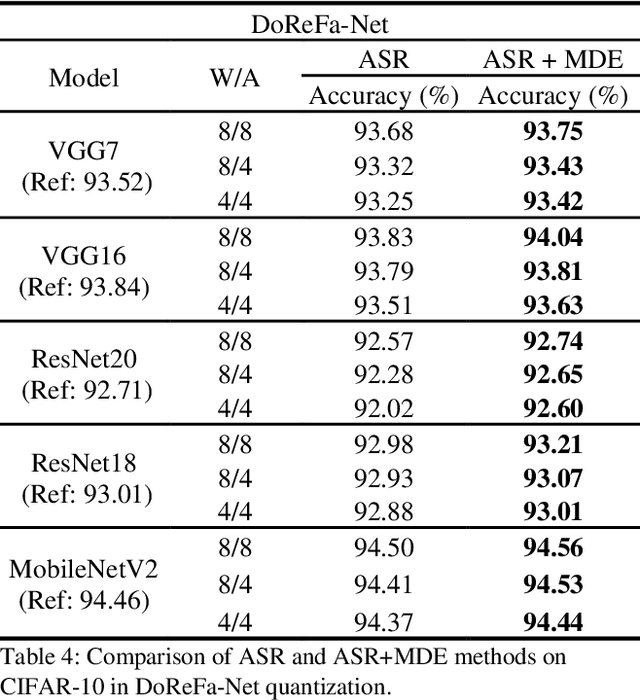
Deep neural networks with lower precision weights and operations at inference time have advantages in terms of the cost of memory space and accelerator power. The main challenge associated with the quantization algorithm is maintaining accuracy at low bit-widths. We propose learned gradient linear symmetric quantization (LG-LSQ) as a method for quantizing weights and activation functions to low bit-widths with high accuracy in integer neural network processors. First, we introduce the scaling simulated gradient (SSG) method for determining the appropriate gradient for the scaling factor of the linear quantizer during the training process. Second, we introduce the arctangent soft round (ASR) method, which differs from the straight-through estimator (STE) method in its ability to prevent the gradient from becoming zero, thereby solving the discrete problem caused by the rounding process. Finally, to bridge the gap between full-precision and low-bit quantization networks, we propose the minimize discretization error (MDE) method to determine an accurate gradient in backpropagation. The ASR+MDE method is a simple alternative to the STE method and is practical for use in different uniform quantization methods. In our evaluation, the proposed quantizer achieved full-precision baseline accuracy in various 3-bit networks, including ResNet18, ResNet34, and ResNet50, and an accuracy drop of less than 1% in the quantization of 4-bit weights and 4-bit activations in lightweight models such as MobileNetV2 and ShuffleNetV2.

MARS: Multi-macro Architecture SRAM CIM-Based Accelerator with Co-designed Compressed Neural Networks

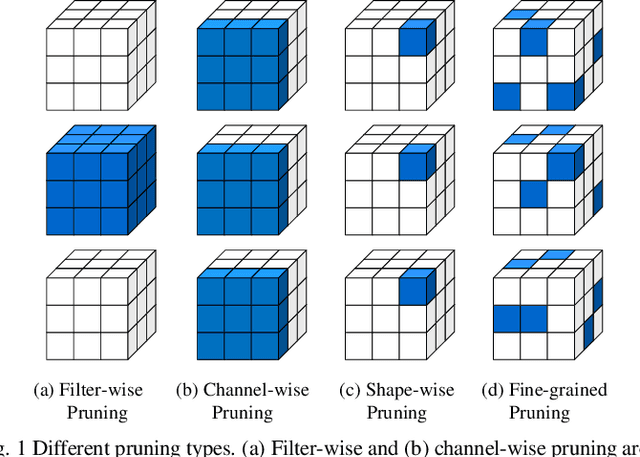
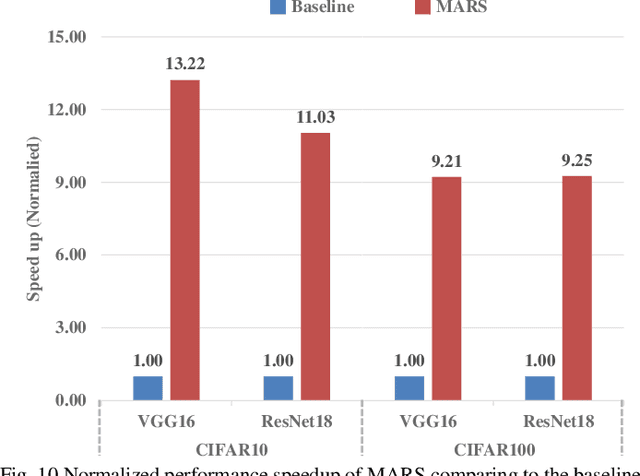
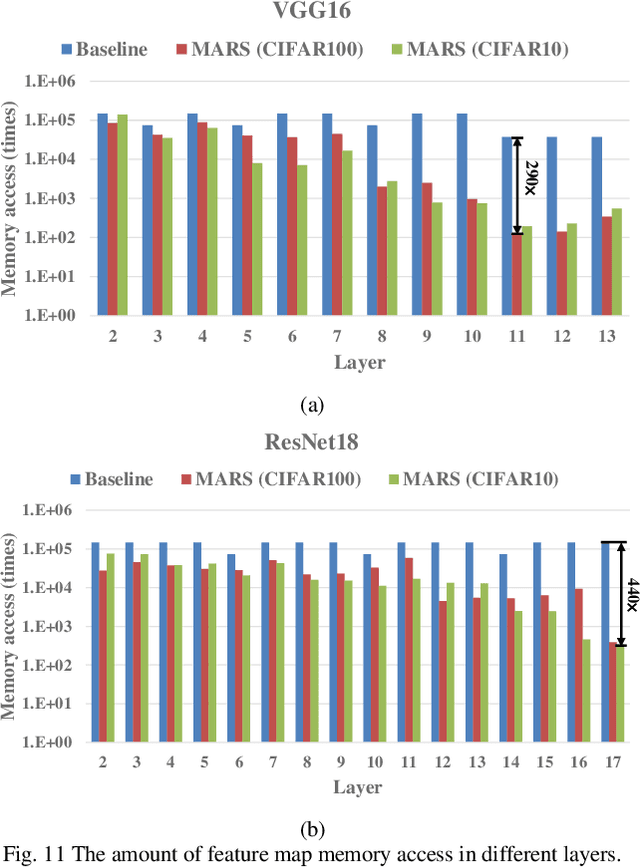
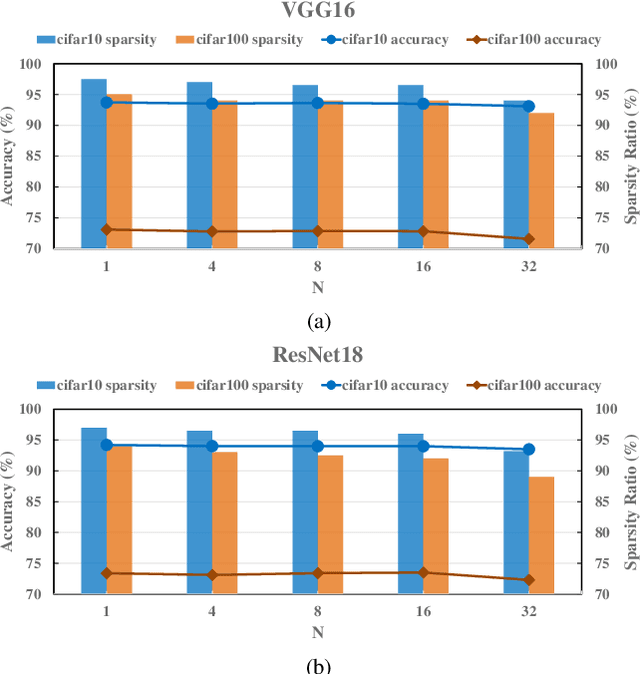
Convolutional neural networks (CNNs) play a key role in deep learning applications. However, the large storage overheads and the substantial computation cost of CNNs are problematic in hardware accelerators. Computing-in-memory (CIM) architecture has demonstrated great potential to effectively compute large-scale matrix-vector multiplication. However, the intensive multiply and accumulation (MAC) operations executed at the crossbar array and the limited capacity of CIM macros remain bottlenecks for further improvement of energy efficiency and throughput. To reduce computation costs, network pruning and quantization are two widely studied compression methods to shrink the model size. However, most of the model compression algorithms can only be implemented in digital-based CNN accelerators. For implementation in a static random access memory (SRAM) CIM-based accelerator, the model compression algorithm must consider the hardware limitations of CIM macros, such as the number of word lines and bit lines that can be turned on at the same time, as well as how to map the weight to the SRAM CIM macro. In this study, a software and hardware co-design approach is proposed to design an SRAM CIM-based CNN accelerator and an SRAM CIM-aware model compression algorithm. To lessen the high-precision MAC required by batch normalization (BN), a quantization algorithm that can fuse BN into the weights is proposed. Furthermore, to reduce the number of network parameters, a sparsity algorithm that considers a CIM architecture is proposed. Last, MARS, a CIM-based CNN accelerator that can utilize multiple SRAM CIM macros as processing units and support a sparsity neural network, is proposed.