${\rm P{\small ROOF}W{\small ALA}}$: Multilingual Proof Data Synthesis and Theorem-Proving

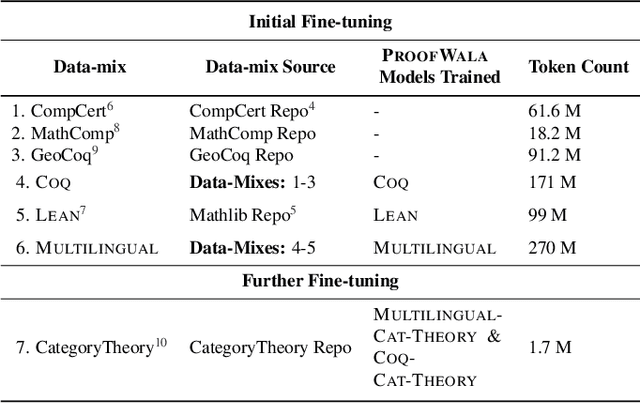
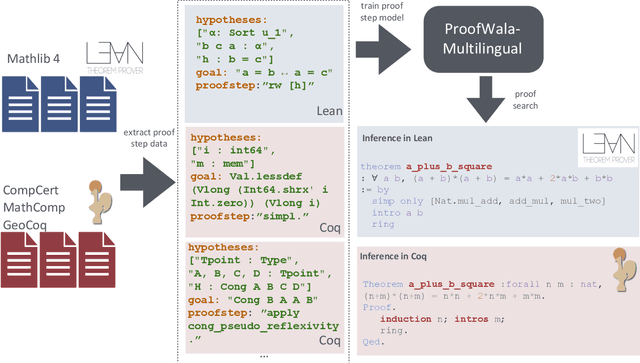
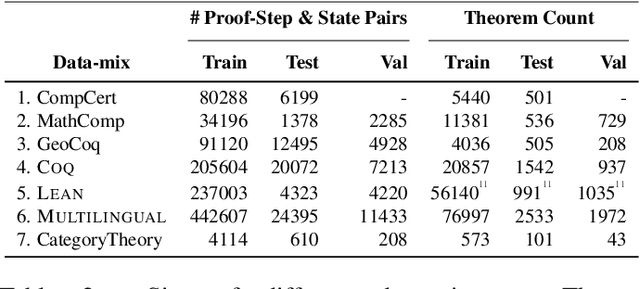
Neural networks have shown substantial promise at automatic theorem-proving in interactive proof assistants (ITPs) like Lean and Coq. However, most neural theorem-proving models are restricted to specific ITPs, leaving out opportunities for cross-lingual $\textit{transfer}$ between ITPs. We address this weakness with a multilingual proof framework, ${\rm P{\small ROOF}W{\small ALA}}$, that allows a standardized form of interaction between neural theorem-provers and two established ITPs (Coq and Lean). It enables the collection of multilingual proof step data -- data recording the result of proof actions on ITP states -- for training neural provers. ${\rm P{\small ROOF}W{\small ALA}}$ allows the systematic evaluation of a model's performance across different ITPs and problem domains via efficient parallel proof search algorithms. We show that multilingual training enabled by ${\rm P{\small ROOF}W{\small ALA}}$ can lead to successful transfer across ITPs. Specifically, a model trained on a mix of ${\rm P{\small ROOF}W{\small ALA}}$-generated Coq and Lean data outperforms Lean-only and Coq-only models on the standard prove-at-$k$ metric. We open source all code including code for the $\href{https://github.com/trishullab/proof-wala}{ProofWala\; Framework}$, and the $\href{https://github.com/trishullab/itp-interface}{Multilingual\; ITP\; interaction\; framework}$.

PutnamBench: Evaluating Neural Theorem-Provers on the Putnam Mathematical Competition

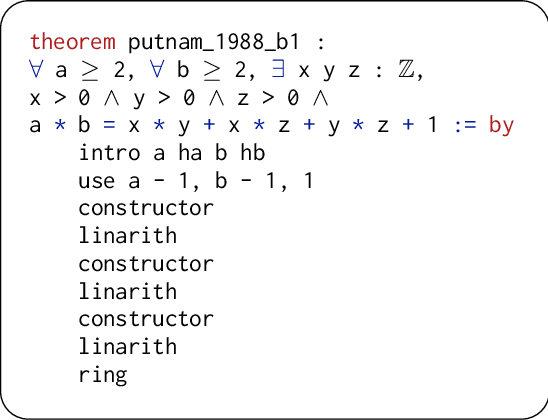
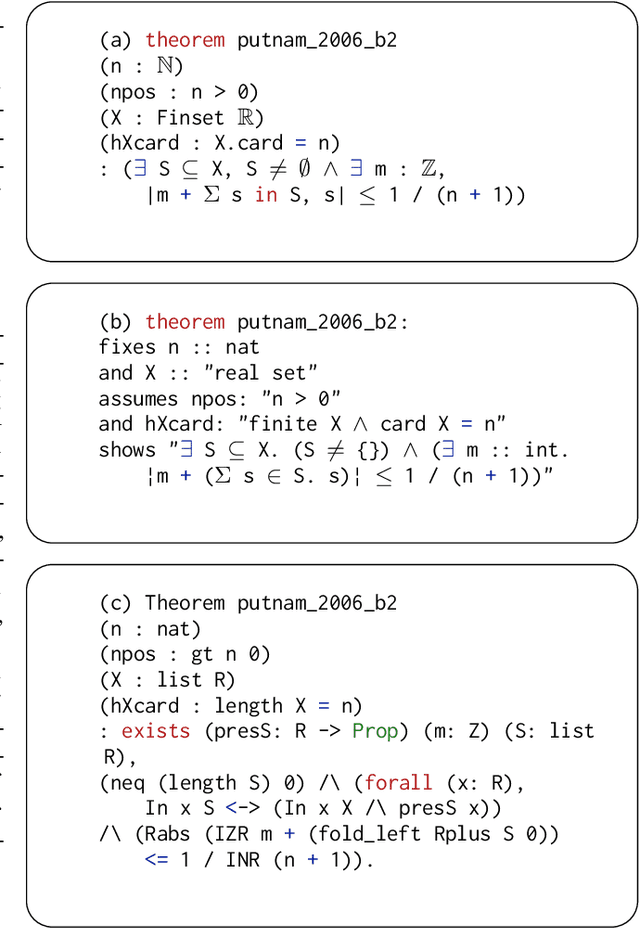
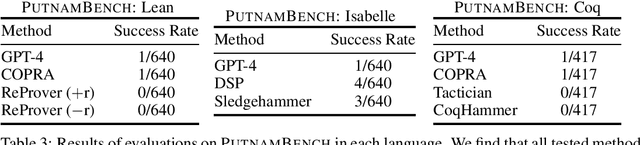
We present PutnamBench, a new multilingual benchmark for evaluating the ability of neural theorem-provers to solve competition mathematics problems. PutnamBench consists of 1697 hand-constructed formalizations of 640 theorems sourced from the William Lowell Putnam Mathematical Competition, the premier undergraduate-level mathematics competition in North America. All the theorems have formalizations in Lean 4 and Isabelle; a substantial subset also has Coq formalizations. Proving the theorems requires significant problem-solving ability and proficiency in a broad range of topics taught in undergraduate mathematics courses. We use PutnamBench to evaluate several established neural and symbolic theorem-provers. These approaches can only solve a handful of the PutnamBench problems, establishing the benchmark as a difficult open challenge for research on neural theorem-proving. PutnamBench is available at https://github.com/trishullab/PutnamBench.

A Language-Agent Approach to Formal Theorem-Proving

Language agents, which use a large language model (LLM) capable of in-context learning to interact with an external environment, have recently emerged as a promising approach to control tasks. We present the first language-agent approach to formal theorem-proving. Our method, COPRA, uses a high-capacity, black-box LLM (GPT-4) as part of a policy for a stateful backtracking search. During the search, the policy can select proof tactics and retrieve lemmas and definitions from an external database. Each selected tactic is executed in the underlying proof framework, and the execution feedback is used to build the prompt for the next policy invocation. The search also tracks selected information from its history and uses it to reduce hallucinations and unnecessary LLM queries. We evaluate COPRA on the miniF2F benchmark for Lean and a set of Coq tasks from the Compcert project. On these benchmarks, COPRA is significantly better than one-shot invocations of GPT-4, as well as state-of-the-art models fine-tuned on proof data, at finding correct proofs quickly.