RIOT: Recursive Inertial Odometry Transformer for Localisation from Low-Cost IMU Measurements

Inertial localisation is an important technique as it enables ego-motion estimation in conditions where external observers are unavailable. However, low-cost inertial sensors are inherently corrupted by bias and noise, which lead to unbound errors, making straight integration for position intractable. Traditional mathematical approaches are reliant on prior system knowledge, geometric theories and are constrained by predefined dynamics. Recent advances in deep learning, that benefit from ever-increasing volumes of data and computational power, allow for data driven solutions that offer more comprehensive understanding. Existing deep inertial odometry solutions rely on estimating the latent states, such as velocity, or are dependant on fixed sensor positions and periodic motion patterns. In this work we propose taking the traditional state estimation recursive methodology and applying it in the deep learning domain. Our approach, which incorporates the true position priors in the training process, is trained on inertial measurements and ground truth displacement data, allowing recursion and to learn both motion characteristics and systemic error bias and drift. We present two end-to-end frameworks for pose invariant deep inertial odometry that utilise self-attention to capture both spatial features and long-range dependencies in inertial data. We evaluate our approaches against a custom 2-layer Gated Recurrent Unit, trained in the same manner on the same data, and tested each approach on a number of different users, devices and activities. Each network had a sequence length weighted relative trajectory error mean $\leq0.4594$m, highlighting the effectiveness of our learning process used in the development of the models.

Gravity aided navigation using Viterbi map matching algorithm

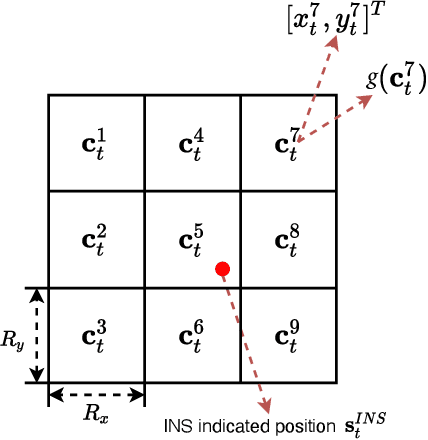
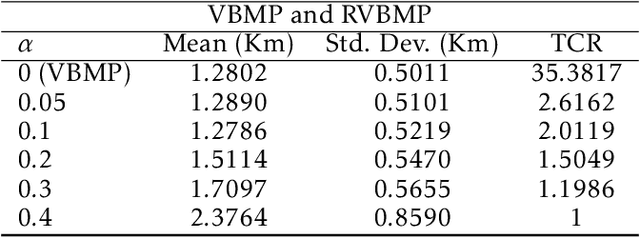
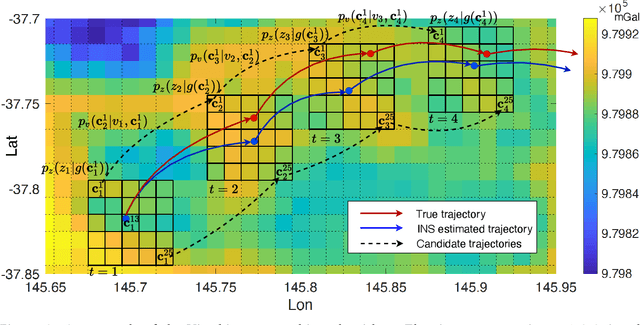
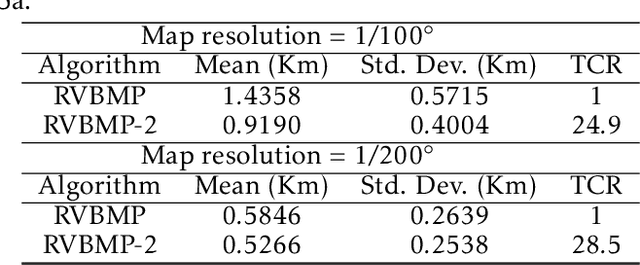
In GNSS-denied environments, aiding a vehicle's inertial navigation system (INS) is crucial to reducing the accumulated navigation drift caused by sensor errors (e.g. bias and noise). One potential solution is to use measurements of gravity as an aiding source. The measurements are matched to a geo-referenced map of Earth's gravity in order to estimate the vehicle's position. In this paper, we propose a novel formulation of the map matching problem using a hidden Markov model (HMM). Specifically, we treat the spatial cells of the map as the hidden states of the HMM and present a Viterbi style algorithm to estimate the most likely sequence of states, i.e. most likely sequence of vehicle positions, that results in the sequence of observed gravity measurements. Using a realistic gravity map, we demonstrate the accuracy of our Viterbi map matching algorithm in a navigation scenario and illustrate its robustness compared to existing methods.

Probabilistic Map Matching for Robust Inertial Navigation Aiding

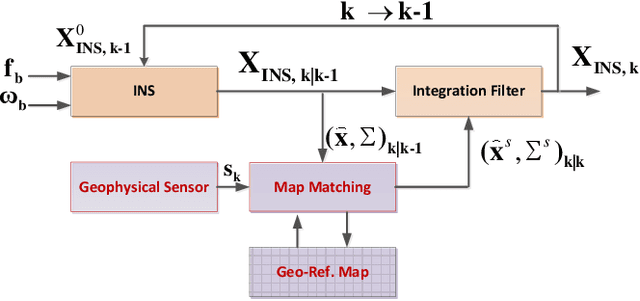
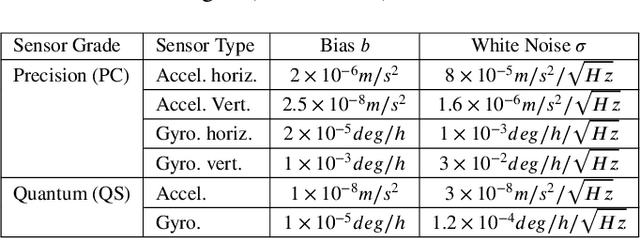
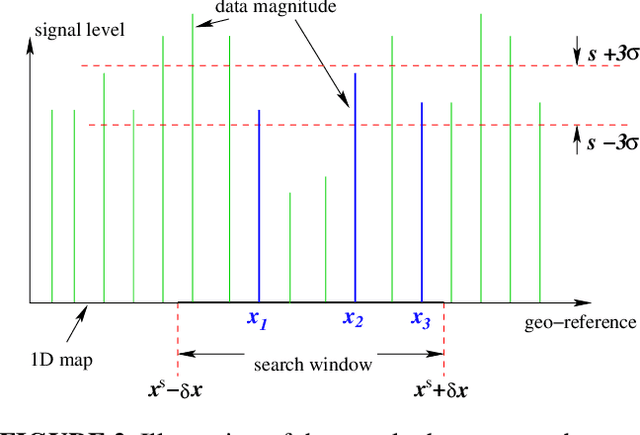
Robust aiding of inertial navigation systems in GNSS-denied environments is critical for the removal of accumulated navigation error caused by the drift and bias inherent in inertial sensors. One way to perform such an aiding uses matching of geophysical measurements, such as gravimetry, gravity gradiometry or magnetometry, with a known geo-referenced map. Although simple in concept, this map matching procedure is challenging: the measurements themselves are noisy; their associated spatial location is uncertain; and the measurements may match multiple points within the map (i.e. non-unique solution). In this paper, we propose a probabilistic multiple hypotheses tracker to solve the map matching problem and allow robust inertial navigation aiding. Our approach addresses the problem both locally, via probabilistic data association, and temporally by incorporating the underlying platform kinematic constraints into the tracker. The map matching output is then integrated into the navigation system using an unscented Kalman filter. Additionally, we present a statistical measure of local map information density -- the map feature variability -- and use it to weight the output covariance of the proposed algorithm. The effectiveness and robustness of the proposed algorithm are demonstrated using a navigation scenario involving gravitational map matching.