Robust Localized Multi-view Subspace Clustering
Paper and Code
May 22, 2017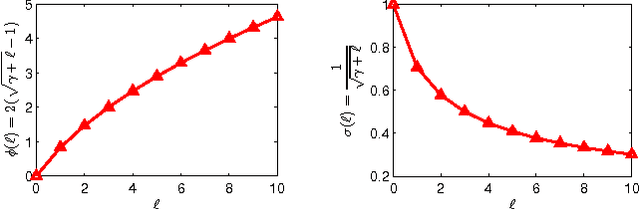
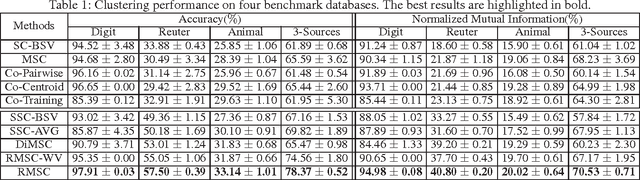
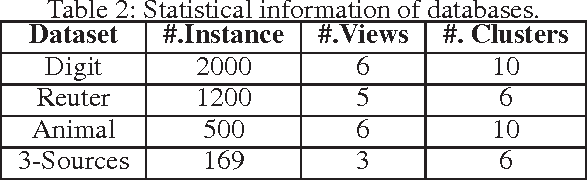

In multi-view clustering, different views may have different confidence levels when learning a consensus representation. Existing methods usually address this by assigning distinctive weights to different views. However, due to noisy nature of real-world applications, the confidence levels of samples in the same view may also vary. Thus considering a unified weight for a view may lead to suboptimal solutions. In this paper, we propose a novel localized multi-view subspace clustering model that considers the confidence levels of both views and samples. By assigning weight to each sample under each view properly, we can obtain a robust consensus representation via fusing the noiseless structures among views and samples. We further develop a regularizer on weight parameters based on the convex conjugacy theory, and samples weights are determined in an adaptive manner. An efficient iterative algorithm is developed with a convergence guarantee. Experimental results on four benchmarks demonstrate the correctness and effectiveness of the proposed model.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge