Modelling Behavioural Diversity for Learning in Open-Ended Games
Paper and Code
Mar 14, 2021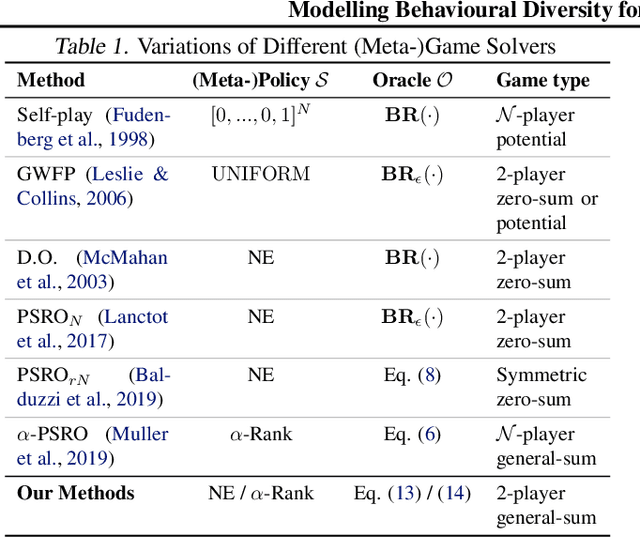

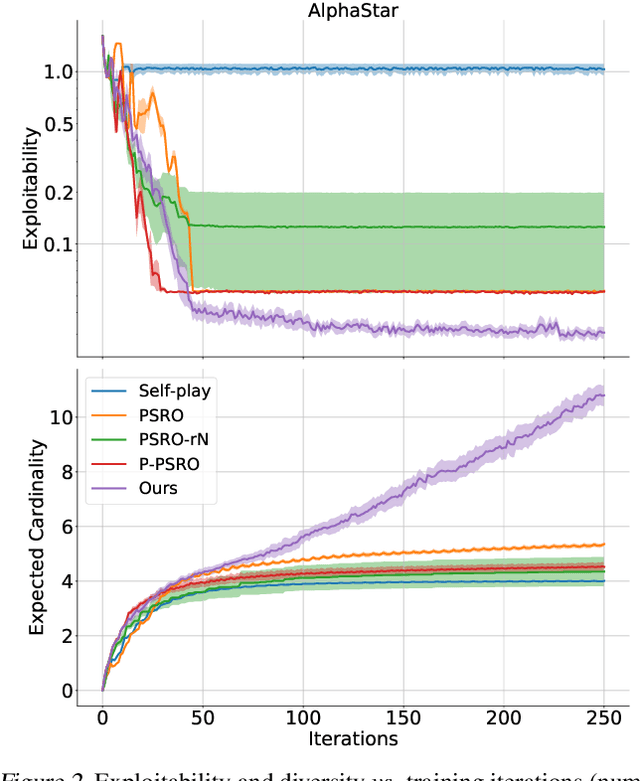
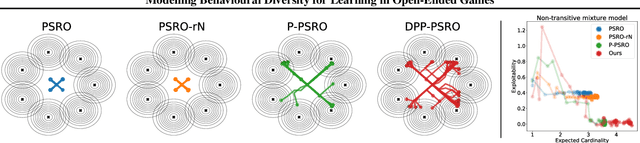
Promoting behavioural diversity is critical for solving games with non-transitive dynamics where strategic cycles exist, and there is no consistent winner (e.g., Rock-Paper-Scissors). Yet, there is a lack of rigorous treatment for defining diversity and constructing diversity-aware learning dynamics. In this work, we offer a geometric interpretation of behavioural diversity in games and introduce a novel diversity metric based on \emph{determinantal point processes} (DPP). By incorporating the diversity metric into best-response dynamics, we develop \emph{diverse fictitious play} and \emph{diverse policy-space response oracle} for solving normal-form games and open-ended games. We prove the uniqueness of the diverse best response and the convergence of our algorithms on two-player games. Importantly, we show that maximising the DPP-based diversity metric guarantees to enlarge the \emph{gamescape} -- convex polytopes spanned by agents' mixtures of strategies. To validate our diversity-aware solvers, we test on tens of games that show strong non-transitivity. Results suggest that our methods achieve much lower exploitability than state-of-the-art solvers by finding effective and diverse strategies.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge