Feedback Linearization for Unknown Systems via Reinforcement Learning
Paper and Code
Oct 29, 2019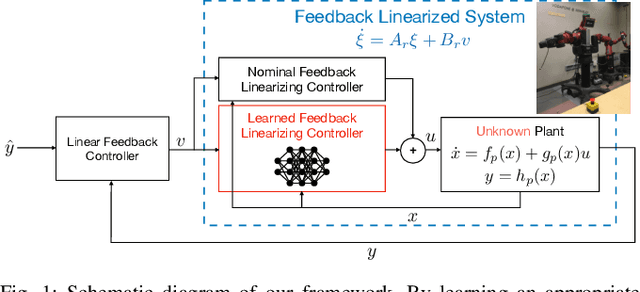
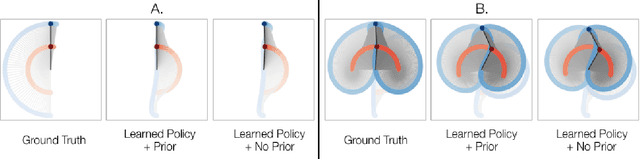
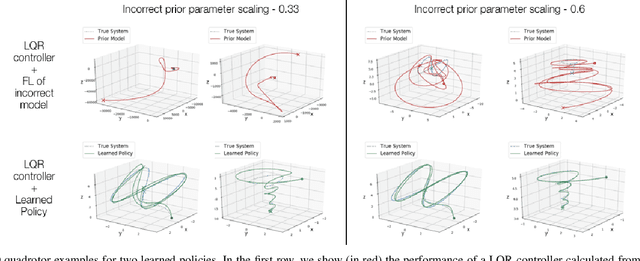
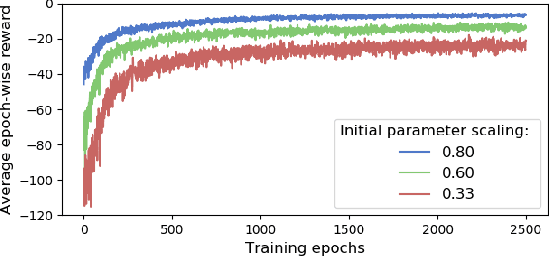
We present a novel approach to control design for nonlinear systems, which leverages reinforcement learning techniques to learn a linearizing controller for a physical plant with unknown dynamics. Feedback linearization is a technique from nonlinear control which renders the input-output dynamics of a nonlinear plant \emph{linear} under application of an appropriate feedback controller. Once a linearizing controller has been constructed, desired output trajectories for the nonlinear plant can be tracked using a variety of linear control techniques. A single learned policy then serves to track arbitrary desired reference signals provided by a higher-level planner. We present theoretical results which provide conditions under which the learning problem has a unique solution which exactly linearizes the plant. We demonstrate the performance of our approach on two simulated problems and a physical robotic platform. For the simulated environments, we observe that the learned feedback linearizing policies can achieve arbitrary tracking of reference trajectories for a fully actuated double pendulum and a 14 dimensional quadrotor. In hardware, we demonstrate that our approach significantly improves tracking performance on a 7-DOF Baxter robot after less than two hours of training.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge