Distributed Evolution Strategies for Black-box Stochastic Optimization
Paper and Code
Apr 09, 2022


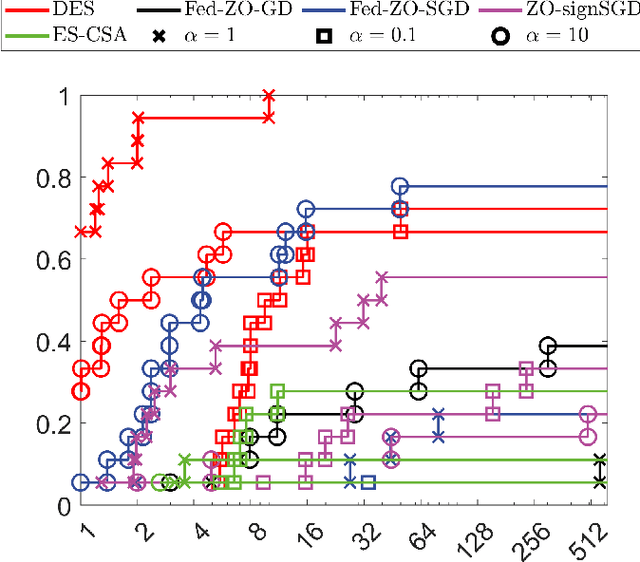
This work concerns the evolutionary approaches to distributed stochastic black-box optimization, in which each worker can individually solve an approximation of the problem with nature-inspired algorithms. We propose a distributed evolution strategy (DES) algorithm grounded on a proper modification to evolution strategies, a family of classic evolutionary algorithms, as well as a careful combination with existing distributed frameworks. On smooth and nonconvex landscapes, DES has a convergence rate competitive to existing zeroth-order methods, and can exploit the sparsity, if applicable, to match the rate of first-order methods. The DES method uses a Gaussian probability model to guide the search and avoids the numerical issue resulted from finite-difference techniques in existing zeroth-order methods. The DES method is also fully adaptive to the problem landscape, as its convergence is guaranteed with any parameter setting. We further propose two alternative sampling schemes which significantly improve the sampling efficiency while leading to similar performance. Simulation studies on several machine learning problems suggest that the proposed methods show much promise in reducing the convergence time and improving the robustness to parameter settings.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge