Comparing Baseline Shapley and Integrated Gradients for Local Explanation: Some Additional Insights

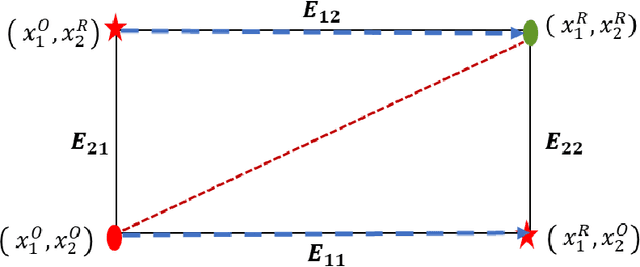
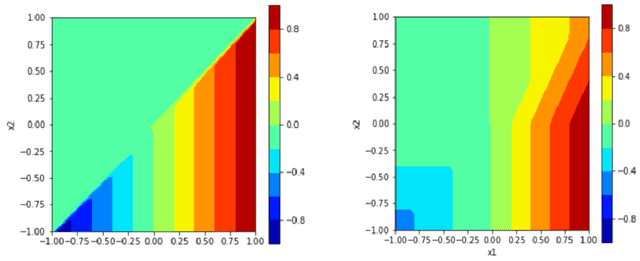
There are many different methods in the literature for local explanation of machine learning results. However, the methods differ in their approaches and often do not provide same explanations. In this paper, we consider two recent methods: Integrated Gradients (Sundararajan, Taly, & Yan, 2017) and Baseline Shapley (Sundararajan and Najmi, 2020). The original authors have already studied the axiomatic properties of the two methods and provided some comparisons. Our work provides some additional insights on their comparative behavior for tabular data. We discuss common situations where the two provide identical explanations and where they differ. We also use simulation studies to examine the differences when neural networks with ReLU activation function is used to fit the models.

Shapley Computations Using Surrogate Model-Based Trees

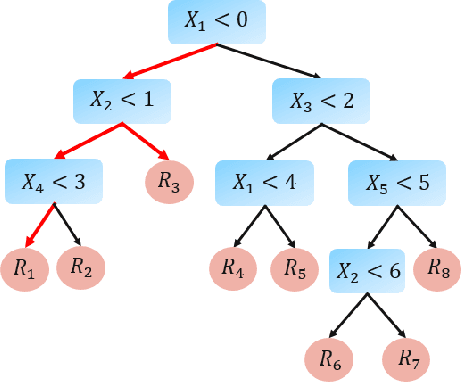
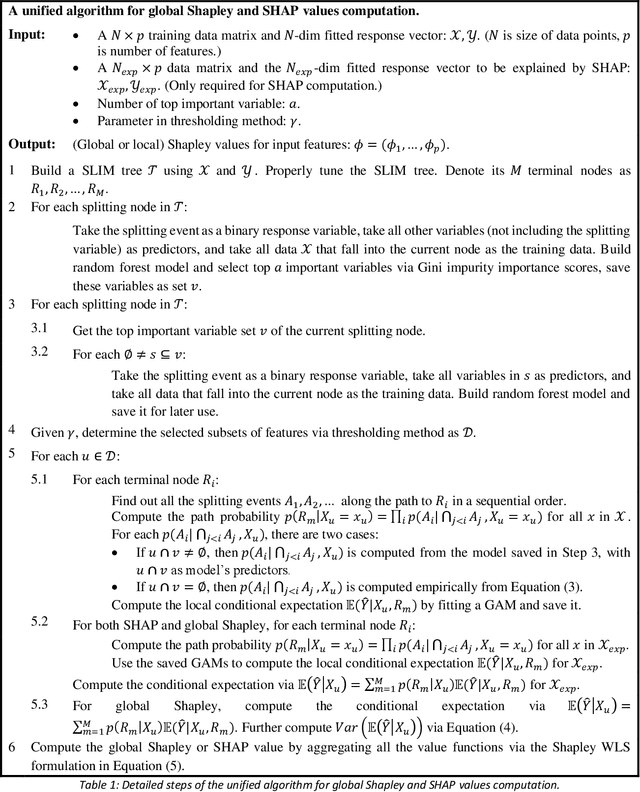
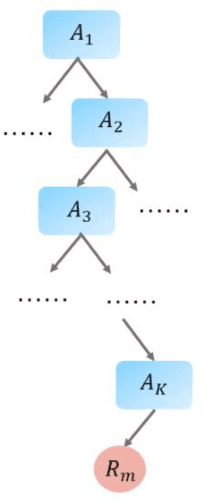
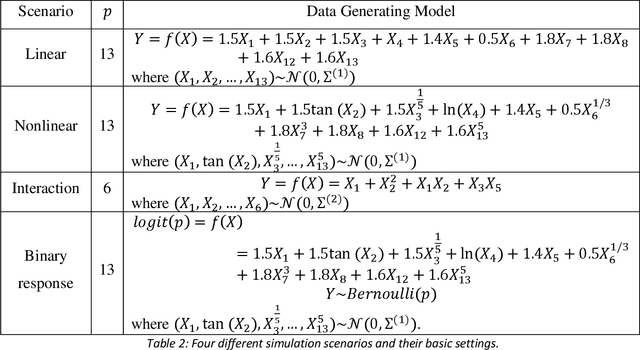
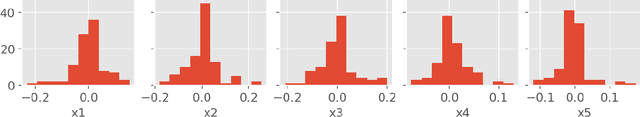
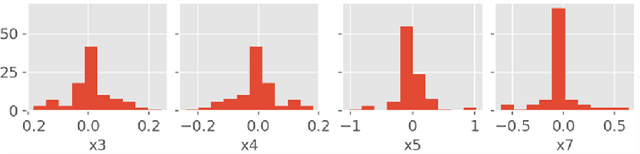
Shapley-related techniques have gained attention as both global and local interpretation tools because of their desirable properties. However, their computation using conditional expectations is computationally expensive. Approximation methods suggested in the literature have limitations. This paper proposes the use of a surrogate model-based tree to compute Shapley and SHAP values based on conditional expectation. Simulation studies show that the proposed algorithm provides improvements in accuracy, unifies global Shapley and SHAP interpretation, and the thresholding method provides a way to trade-off running time and accuracy.