Unveiling Processing--Property Relationships in Laser Powder Bed Fusion: The Synergy of Machine Learning and High-throughput Experiments

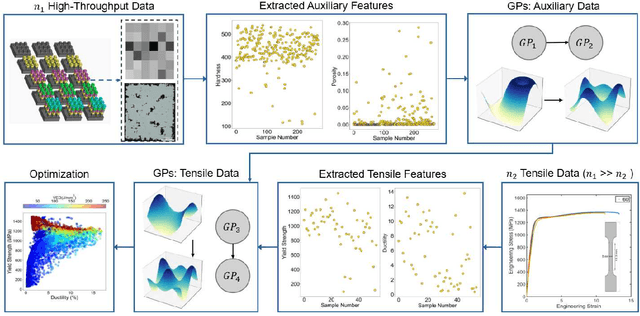
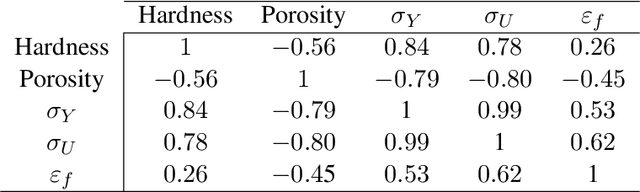
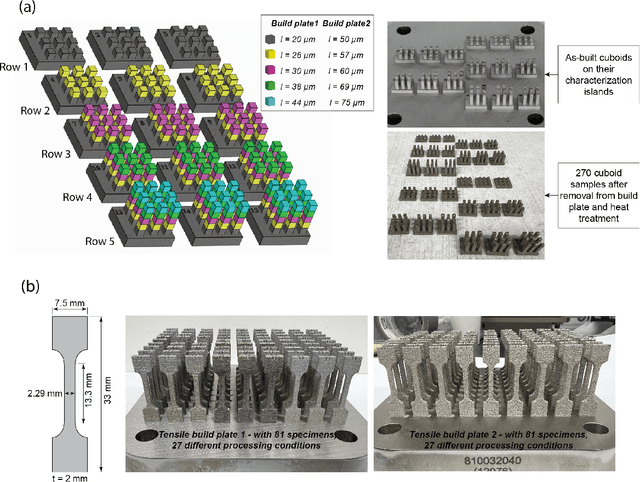
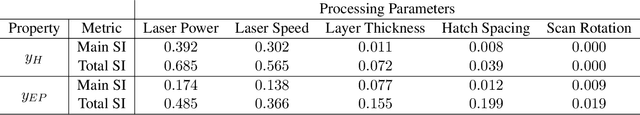
Achieving desired mechanical properties in additive manufacturing requires many experiments and a well-defined design framework becomes crucial in reducing trials and conserving resources. Here, we propose a methodology embracing the synergy between high-throughput (HT) experimentation and hierarchical machine learning (ML) to unveil the complex relationships between a large set of process parameters in Laser Powder Bed Fusion (LPBF) and selected mechanical properties (tensile strength and ductility). The HT method envisions the fabrication of small samples for rapid automated hardness and porosity characterization, and a smaller set of tensile specimens for more labor-intensive direct measurement of yield strength and ductility. The ML approach is based on a sequential application of Gaussian processes (GPs) where the correlations between process parameters and hardness/porosity are first learnt and subsequently adopted by the GPs that relate strength and ductility to process parameters. Finally, an optimization scheme is devised that leverages these GPs to identify the processing parameters that maximize combinations of strength and ductility. By founding the learning on larger easy-to-collect and smaller labor-intensive data, we reduce the reliance on expensive characterization and enable exploration of a large processing space. Our approach is material-agnostic and herein we demonstrate its application on 17-4PH stainless steel.

GP+: A Python Library for Kernel-based learning via Gaussian Processes

In this paper we introduce GP+, an open-source library for kernel-based learning via Gaussian processes (GPs) which are powerful statistical models that are completely characterized by their parametric covariance and mean functions. GP+ is built on PyTorch and provides a user-friendly and object-oriented tool for probabilistic learning and inference. As we demonstrate with a host of examples, GP+ has a few unique advantages over other GP modeling libraries. We achieve these advantages primarily by integrating nonlinear manifold learning techniques with GPs' covariance and mean functions. As part of introducing GP+, in this paper we also make methodological contributions that (1) enable probabilistic data fusion and inverse parameter estimation, and (2) equip GPs with parsimonious parametric mean functions which span mixed feature spaces that have both categorical and quantitative variables. We demonstrate the impact of these contributions in the context of Bayesian optimization, multi-fidelity modeling, sensitivity analysis, and calibration of computer models.

On the Effects of Heterogeneous Errors on Multi-fidelity Bayesian Optimization

Bayesian optimization (BO) is a sequential optimization strategy that is increasingly employed in a wide range of areas including materials design. In real world applications, acquiring high-fidelity (HF) data through physical experiments or HF simulations is the major cost component of BO. To alleviate this bottleneck, multi-fidelity (MF) methods are used to forgo the sole reliance on the expensive HF data and reduce the sampling costs by querying inexpensive low-fidelity (LF) sources whose data are correlated with HF samples. However, existing multi-fidelity BO (MFBO) methods operate under the following two assumptions that rarely hold in practical applications: (1) LF sources provide data that are well correlated with the HF data on a global scale, and (2) a single random process can model the noise in the fused data. These assumptions dramatically reduce the performance of MFBO when LF sources are only locally correlated with the HF source or when the noise variance varies across the data sources. In this paper, we dispense with these incorrect assumptions by proposing an MF emulation method that (1) learns a noise model for each data source, and (2) enables MFBO to leverage highly biased LF sources which are only locally correlated with the HF source. We illustrate the performance of our method through analytical examples and engineering problems on materials design.

Unsupervised Anomaly Detection via Nonlinear Manifold Learning

Anomalies are samples that significantly deviate from the rest of the data and their detection plays a major role in building machine learning models that can be reliably used in applications such as data-driven design and novelty detection. The majority of existing anomaly detection methods either are exclusively developed for (semi) supervised settings, or provide poor performance in unsupervised applications where there is no training data with labeled anomalous samples. To bridge this research gap, we introduce a robust, efficient, and interpretable methodology based on nonlinear manifold learning to detect anomalies in unsupervised settings. The essence of our approach is to learn a low-dimensional and interpretable latent representation (aka manifold) for all the data points such that normal samples are automatically clustered together and hence can be easily and robustly identified. We learn this low-dimensional manifold by designing a learning algorithm that leverages either a latent map Gaussian process (LMGP) or a deep autoencoder (AE). Our LMGP-based approach, in particular, provides a probabilistic perspective on the learning task and is ideal for high-dimensional applications with scarce data. We demonstrate the superior performance of our approach over existing technologies via multiple analytic examples and real-world datasets.

Multi-Fidelity Cost-Aware Bayesian Optimization

Bayesian optimization (BO) is increasingly employed in critical applications such as materials design and drug discovery. An increasingly popular strategy in BO is to forgo the sole reliance on high-fidelity data and instead use an ensemble of information sources which provide inexpensive low-fidelity data. The overall premise of this strategy is to reduce the overall sampling costs by querying inexpensive low-fidelity sources whose data are correlated with high-fidelity samples. Here, we propose a multi-fidelity cost-aware BO framework that dramatically outperforms the state-of-the-art technologies in terms of efficiency, consistency, and robustness. We demonstrate the advantages of our framework on analytic and engineering problems and argue that these benefits stem from our two main contributions: (1) we develop a novel acquisition function for multi-fidelity cost-aware BO that safeguards the convergence against the biases of low-fidelity data, and (2) we tailor a newly developed emulator for multi-fidelity BO which enables us to not only simultaneously learn from an ensemble of multi-fidelity datasets, but also identify the severely biased low-fidelity sources that should be excluded from BO.