Study of filtered-x logarithmic recursive least $p$-power algorithm

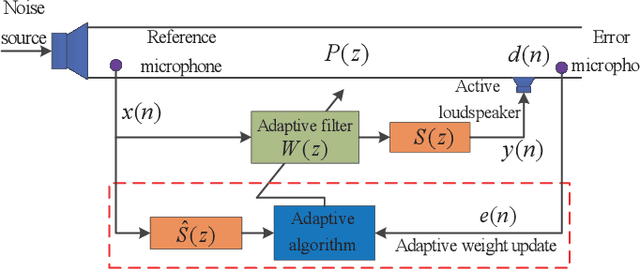
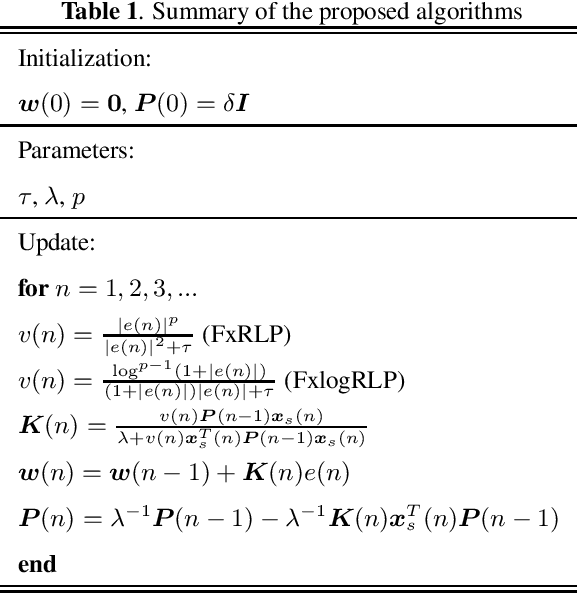
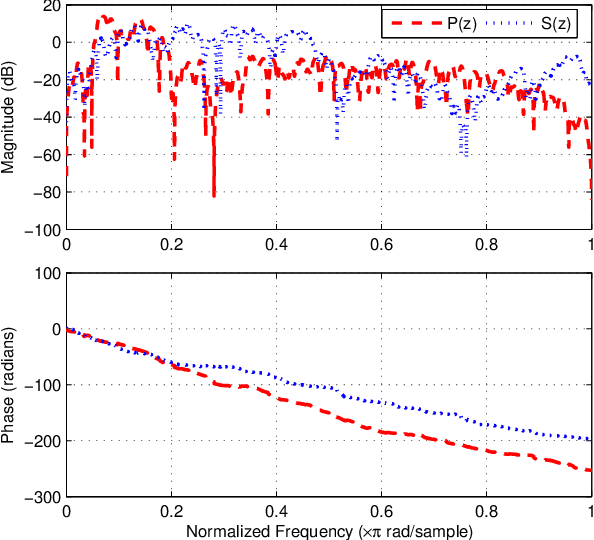
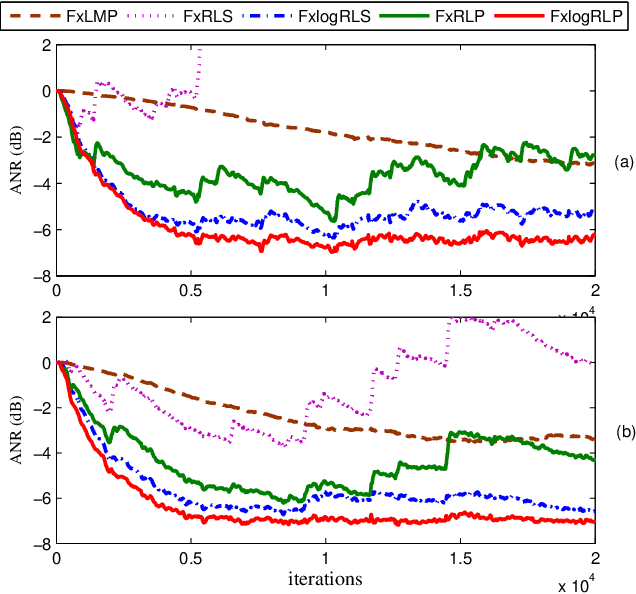
For active impulsive noise control, a filtered-x recursive least $p$-power (FxRLP) algorithm is proposed by minimizing the weighted summation of the $p$-power of the \emph{a posteriori} errors. Since the characteristic of the target noise is investigated, the FxRLP algorithm achieves good performance and robustness. To obtain a better performance, we develop a filtered-x logarithmic recursive least $p$-power (FxlogRLP) algorithm which integrates the $p$-order moment with the logarithmic-order moment. Simulation results demonstrate that the FxlogRLP algorithm is superior to the existing algorithms in terms of convergence rate and noise reduction.

Robust DCD-Based Recursive Adaptive Algorithms

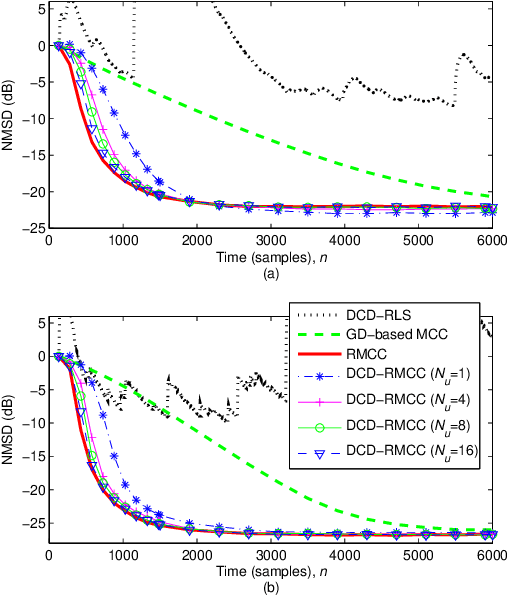
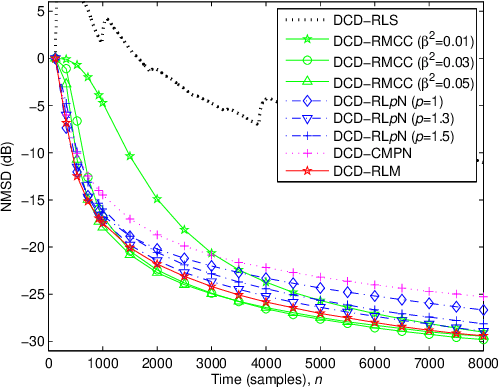
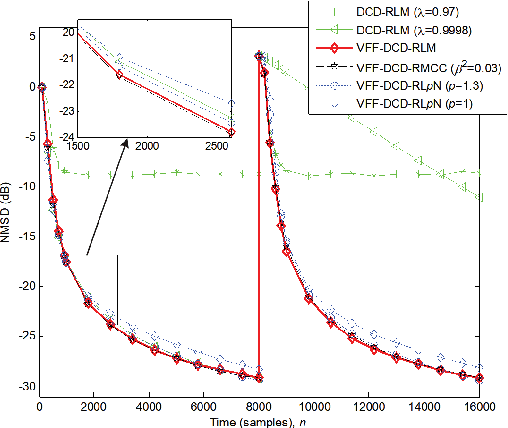
The dichotomous coordinate descent (DCD) algorithm has been successfully used for significant reduction in the complexity of recursive least squares (RLS) algorithms. In this work, we generalize the application of the DCD algorithm to RLS adaptive filtering in impulsive noise scenarios and derive a unified update formula. By employing different robust strategies against impulsive noise, we develop novel computationally efficient DCD-based robust recursive algorithms. Furthermore, to equip the proposed algorithms with the ability to track abrupt changes in unknown systems, a simple variable forgetting factor mechanism is also developed. Simulation results for channel identification scenarios in impulsive noise demonstrate the effectiveness of the proposed algorithms.