One-Bit Target Detection in Collocated MIMO Radar with Colored Background Noise

One-bit sampling has emerged as a promising technique in multiple-input multiple-output (MIMO) radar systems due to its ability to significantly reduce data volume and processing requirements. Nevertheless, current detection methods have not adequately addressed the impact of colored noise, which is frequently encountered in real scenarios. In this paper, we present a novel detection method that accounts for colored noise in MIMO radar systems. Specifically, we derive Rao's test by computing the derivative of the likelihood function with respect to the target reflectivity parameter and the Fisher information matrix, resulting in a detector that takes the form of a weighted matched filter. To ensure the constant false alarm rate (CFAR) property, we also consider noise covariance uncertainty and examine its effect on the probability of false alarm. The detection probability is also studied analytically. Simulation results demonstrate that the proposed detector provides considerable performance gains in the presence of colored noise.

One-Bit Spectrum Sensing for Cognitive Radio

Spectrum sensing in cognitive radio necessitates effective monitoring of wide bandwidths, which requires high-rate sampling. Traditional spectrum sensing methods employing high-precision analog-to-digital converters (ADCs) result in increased power consumption and expensive hardware costs. In this paper, we explore blind spectrum sensing utilizing one-bit ADCs. We derive a closed-form detector based on Rao's test and demonstrate its equivalence with the second-order eigenvalue-moment-ratio test. Furthermore, a near-exact distribution based on the moment-based method, and an approximate distribution in the low signal-to-noise ratio (SNR) regime with the use of the central limit theorem, are obtained. Theoretical analysis is then performed and our results show that the performance loss of the proposed detector is approximately $2$ dB ($\pi/2$) compared to detectors employing $\infty$-bit ADCs when SNR is low. This loss can be compensated for by using approximately $2.47$ ($\pi^2/4$) times more samples. In addition, we unveil that the efficiency of incoherent accumulation in one-bit detection is the square root of that of coherent accumulation. Simulation results corroborate the correctness of our theoretical calculations.

One-Bit Covariance Reconstruction with Non-zero Thresholds: Algorithm and Performance Analysis

Covariance matrix reconstruction is a topic of great significance in the field of one-bit signal processing and has numerous practical applications. Despite its importance, the conventional arcsine law with zero threshold is incapable of recovering the diagonal elements of the covariance matrix. To address this limitation, recent studies have proposed the use of non-zero clipping thresholds. However, the relationship between the estimation error and the sampling threshold is not yet known. In this paper, we undertake an analysis of the mean squared error by computing the Fisher information matrix for a given threshold. Our results reveal that the optimal threshold can vary considerably, depending on the variances and correlation coefficients. As a result, it is inappropriate to use a constant threshold to encompass parameters that vary widely. To mitigate this issue, we present a recovery scheme that incorporates time-varying thresholds. Our approach differs from existing methods in that it utilizes the exact values of the threshold, rather than its statistical properties, to enhance the estimation performance. Our simulations, including the direction-of-arrival estimation problem, demonstrate the efficacy of the developed scheme, especially in complex scenarios where the covariance elements are widely separated.

One-Bit Target Detection in Collocated MIMO Radar and Performance Degradation Analysis

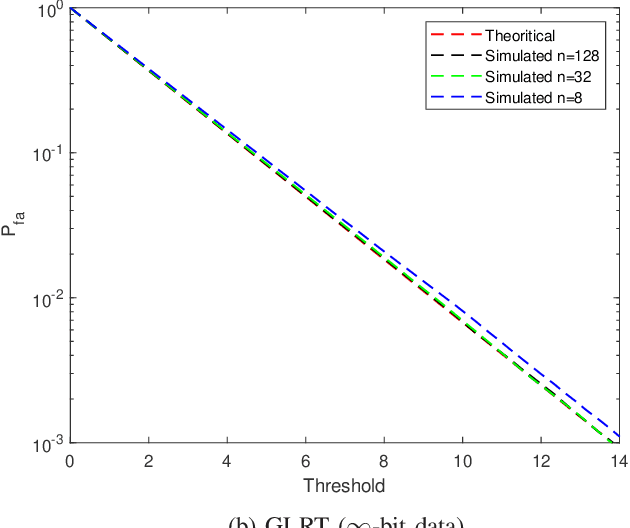
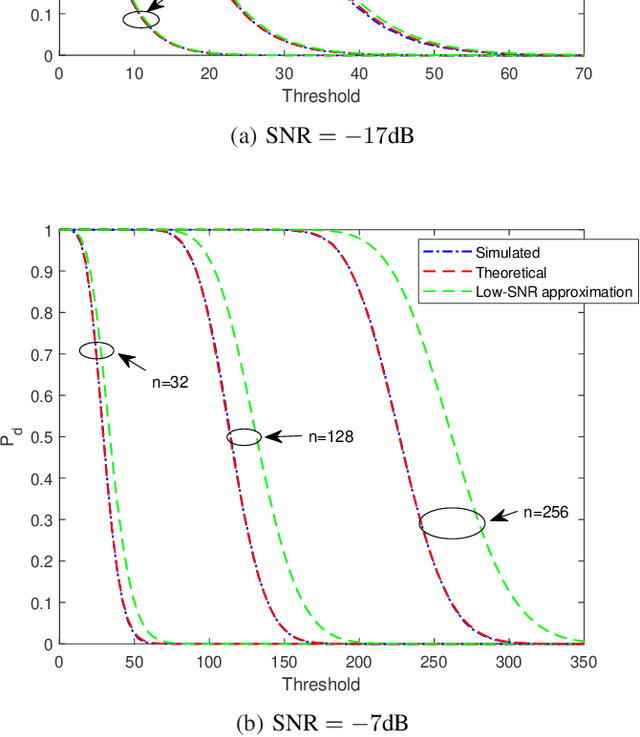
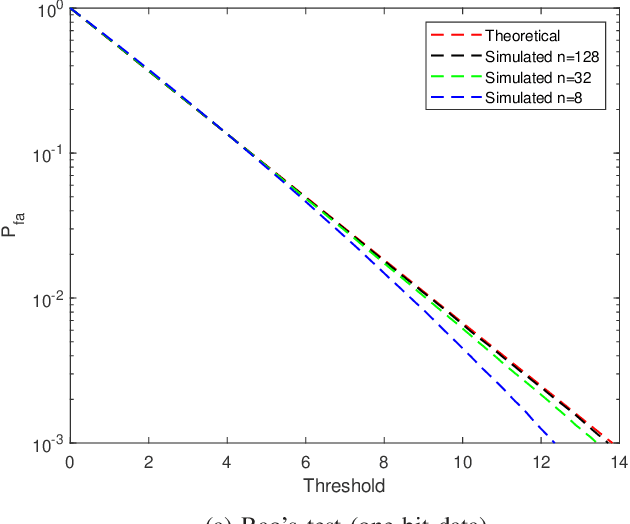
Target detection is an important problem in multiple-input multiple-output (MIMO) radar. Many existing target detection algorithms were proposed without taking into consideration the quantization error caused by analog-to-digital converters (ADCs). This paper addresses the problem of target detection for MIMO radar with one-bit ADCs and derives a Rao's test-based detector. The proposed method has several appealing features: 1) it is a closed-form detector; 2) it allows us to handle sign measurements straightforwardly; 3) there are closed-form approximations of the detector's distributions, which allow us to theoretically evaluate its performance. Moreover, the closed-form distributions allow us to study the performance degradation due to the one-bit ADCs, yielding an approximate $2$ dB loss in the low-signal-to-noise-ratio (SNR) regime compared to $\infty$-bit ADCs. Simulation results are included to showcase the advantage of the proposed detector and validate the accuracy of the theoretical results.