Distributionally Robust Kalman Filtering over Finite and Infinite Horizon

This paper investigates the distributionally robust filtering of signals generated by state-space models driven by exogenous disturbances with noisy observations in finite and infinite horizon scenarios. The exact joint probability distribution of the disturbances and noise is unknown but assumed to reside within a Wasserstein-2 ambiguity ball centered around a given nominal distribution. We aim to derive a causal estimator that minimizes the worst-case mean squared estimation error among all possible distributions within this ambiguity set. We remove the iid restriction in prior works by permitting arbitrarily time-correlated disturbances and noises. In the finite horizon setting, we reduce this problem to a semi-definite program (SDP), with computational complexity scaling with the time horizon. For infinite horizon settings, we characterize the optimal estimator using Karush-Kuhn-Tucker (KKT) conditions. Although the optimal estimator lacks a rational form, i.e., a finite-dimensional state-space realization, it can be fully described by a finite-dimensional parameter. {Leveraging this parametrization, we propose efficient algorithms that compute the optimal estimator with arbitrary fidelity in the frequency domain.} Moreover, given any finite degree, we provide an efficient convex optimization algorithm that finds the finite-dimensional state-space estimator that best approximates the optimal non-rational filter in ${\cal H}_\infty$ norm. This facilitates the practical implementation of the infinite horizon filter without having to grapple with the ill-scaled SDP from finite time. Finally, numerical simulations demonstrate the effectiveness of our approach in practical scenarios.

Achieving Low Complexity Neural Decoders via Iterative Pruning

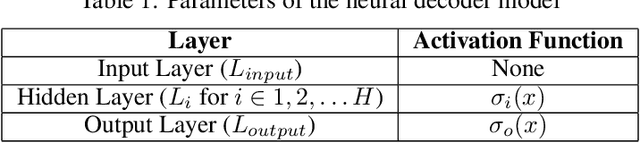
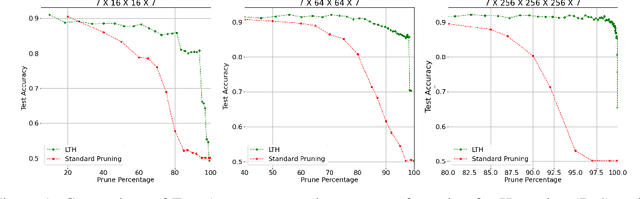
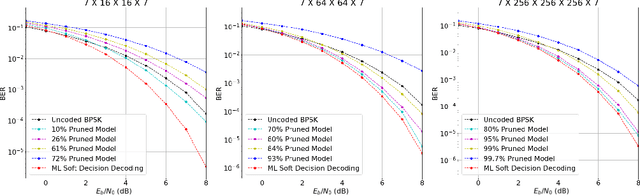
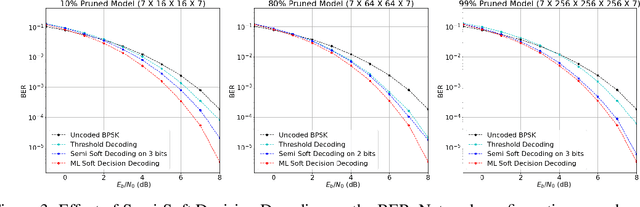
The advancement of deep learning has led to the development of neural decoders for low latency communications. However, neural decoders can be very complex which can lead to increased computation and latency. We consider iterative pruning approaches (such as the lottery ticket hypothesis algorithm) to prune weights in neural decoders. Decoders with fewer number of weights can have lower latency and lower complexity while retaining the accuracy of the original model. This will make neural decoders more suitable for mobile and other edge devices with limited computational power. We also propose semi-soft decision decoding for neural decoders which can be used to improve the bit error rate performance of the pruned network.