An Analytic Solution to the 3D CSC Dubins Path Problem

We present an analytic solution to the 3D Dubins path problem for paths composed of an initial circular arc, a straight component, and a final circular arc. These are commonly called CSC paths. By modeling the start and goal configurations of the path as the base frame and final frame of an RRPRR manipulator, we treat this as an inverse kinematics problem. The kinematic features of the 3D Dubins path are built into the constraints of our manipulator model. Furthermore, we show that the number of solutions is not constant, with up to seven valid CSC path solutions even in non-singular regions. An implementation of solution is available at https://github.com/aabecker/dubins3D.

Minimum-Time Planar Paths with up to Two Constant Acceleration Inputs and $L_2$ Velocity and Acceleration Constraints

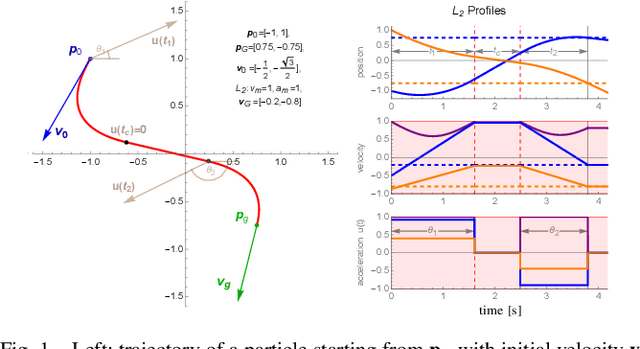
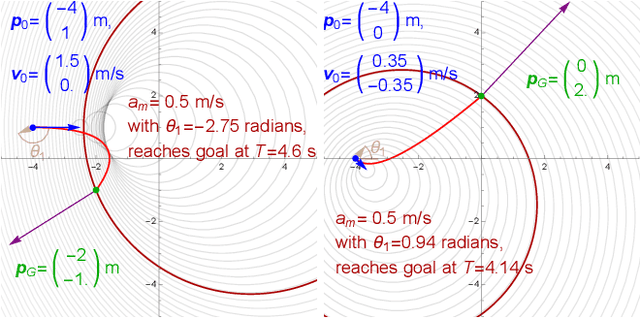
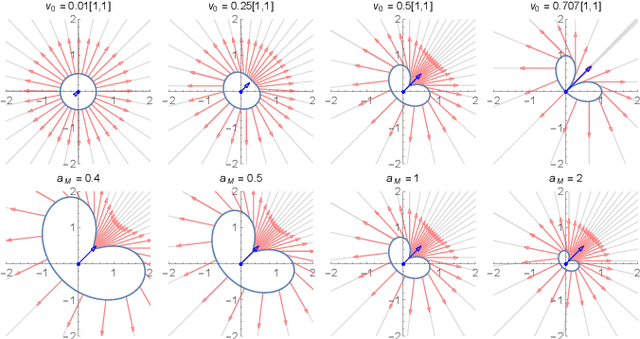
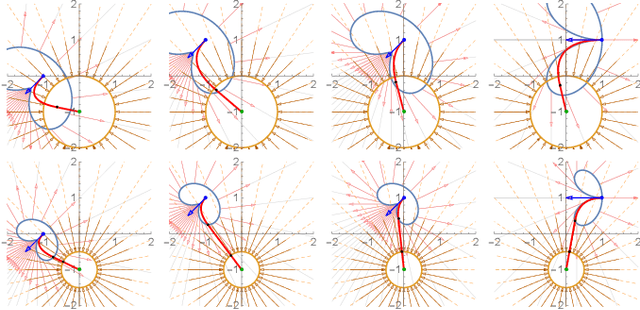
Given starting and ending positions and velocities, $L_2$ bounds on the acceleration and velocity, and the restriction to no more than two constant control inputs, this paper provides routines to compute the minimal-time path. Closed form solutions are provided for reaching a position in minimum time with and without a velocity bound, and for stopping at the goal position. A numeric solver is used to reach a goal position and velocity with no more than two constant control inputs. If a cruising phase at the terminal velocity is needed, this requires solving a non-linear equation with a single parameter. Code is provided on GitHub at https://github.com/RoboticSwarmControl/MinTimeL2pathsConstraints.