Geometric Spatio-Spectral Total Variation for Hyperspectral Image Denoising and Destriping

This article proposes a novel regularization method, named Geometric Spatio-Spectral Total Variation (GeoSSTV), for hyperspectral (HS) image denoising and destriping. HS images are inevitably affected by various types of noise due to the measurement equipment and environment. Total Variation (TV)-based regularization methods that model the spatio-spectral piecewise smoothness inherent in HS images are promising approaches for HS image denoising and destriping. However, existing TV-based methods are based on classical anisotropic and isotropic TVs, which cause staircase artifacts and lack rotation invariance, respectively, making it difficult to accurately recover round structures and oblique edges. To address this issue, GeoSSTV introduces a geometrically consistent formulation of TV that measures variations across all directions in a Euclidean manner. Through this formulation, GeoSSTV removes noise while preserving round structures and oblique edges. Furthermore, we formulate the HS image denoising problem as a constrained convex optimization problem involving GeoSSTV and develop an efficient algorithm based on a preconditioned primal-dual splitting method. Experimental results on HS images contaminated with mixed noise demonstrate the superiority of the proposed method over existing approaches.

Sampling Method for Generalized Graph Signals with Pre-selected Vertices via DC Optimization

This paper proposes a method for vertex-wise flexible sampling of a broad class of graph signals, designed to attain the best possible recovery based on the generalized sampling theory. This is achieved by designing a sampling operator by an optimization problem, which is inherently non-convex, as the best possible recovery imposes a rank constraint. An existing method for vertex-wise flexible sampling is able to control the number of active vertices but cannot incorporate prior knowledge of mandatory or forbidden vertices. To address these challenges, we formulate the operator design as a problem that handles a constraint of the number of active vertices and prior knowledge on specific vertices for sampling, mandatory inclusion or exclusion. We transformed this constrained problem into a difference-of-convex (DC) optimization problem by using the nuclear norm and a DC penalty for vertex selection. To solve this, we develop a convergent solver based on the general double-proximal gradient DC algorithm. The effectiveness of our method is demonstrated through experiments on various graph signal models, including real-world data, showing superior performance in the recovery accuracy by comparing to existing methods.

Efficient and Accurate Full-Waveform Inversion with Total Variation Constraint

This paper proposes a computationally efficient algorithm to address the Full-Waveform Inversion (FWI) problem with a Total Variation (TV) constraint, designed to accurately reconstruct subsurface properties from seismic data. FWI, as an ill-posed inverse problem, requires effective regularizations or constraints to ensure accurate and stable solutions. Among these, the TV constraint is widely known as a powerful prior for modeling the piecewise smooth structure of subsurface properties. However, solving the optimization problem is challenging because of the nonlinear observation process combined with the non-smoothness of the TV constraint. Conventional methods rely on inner loops and/or approximations, which lead to high computational cost and/or inappropriate solutions. To address these limitations, we develop a novel algorithm based on a primal-dual splitting method, achieving computational efficiency by eliminating inner loops and ensuring high accuracy by avoiding approximations. We also demonstrate the effectiveness of the proposed method through experiments using the SEG/EAGE Salt and Overthrust Models. The source code will be available at https://www.mdi.c.titech.ac.jp/publications/fwiwtv.

Convergent Primal-Dual Plug-and-Play Image Restoration: A General Algorithm and Applications

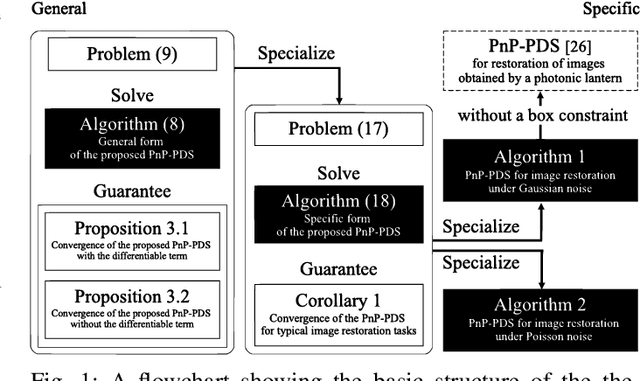
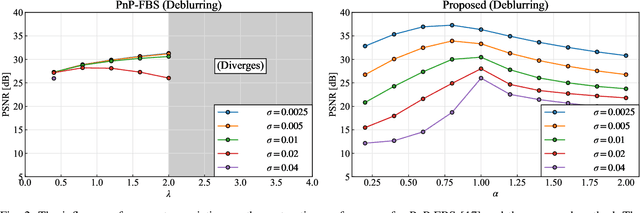
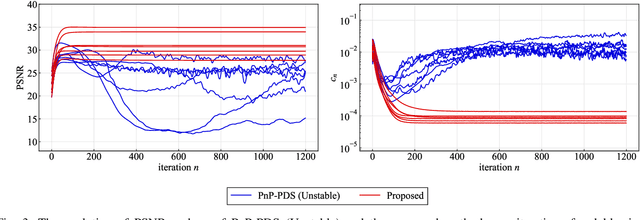
We propose a general deep plug-and-play (PnP) algorithm with a theoretical convergence guarantee. PnP strategies have demonstrated outstanding performance in various image restoration tasks by exploiting the powerful priors underlying Gaussian denoisers. However, existing PnP methods often lack theoretical convergence guarantees under realistic assumptions due to their ad-hoc nature, resulting in inconsistent behavior. Moreover, even when convergence guarantees are provided, they are typically designed for specific settings or require a considerable computational cost in handling non-quadratic data-fidelity terms and additional constraints, which are key components in many image restoration scenarios. To tackle these challenges, we integrate the PnP paradigm with primal-dual splitting (PDS), an efficient proximal splitting methodology for solving a wide range of convex optimization problems, and develop a general convergent PnP framework. Specifically, we establish theoretical conditions for the convergence of the proposed PnP algorithm under a reasonable assumption. Furthermore, we show that the problem solved by the proposed PnP algorithm is not a standard convex optimization problem but a more general monotone inclusion problem, where we provide a mathematical representation of the solution set. Our approach efficiently handles a broad class of image restoration problems with guaranteed theoretical convergence. Numerical experiments on specific image restoration tasks validate the practicality and effectiveness of our theoretical results.

Introducing Graph Learning over Polytopic Uncertain Graph

This extended abstract introduces a class of graph learning applicable to cases where the underlying graph has polytopic uncertainty, i.e., the graph is not exactly known, but its parameters or properties vary within a known range. By incorporating this assumption that the graph lies in a polytopic set into two established graph learning frameworks, we find that our approach yields better results with less computation.

Spatio-Spectral Structure Tensor Total Variation for Hyperspectral Image Denoising and Destriping

This paper proposes a novel regularization method, named Spatio-Spectral Structure Tensor Total Variation (S3TTV), for denoising and destriping of hyperspectral (HS) images. HS images are inevitably contaminated by various types of noise, during acquisition process, due to the measurement equipment and the environment. For HS image denoising and destriping tasks, Spatio-Spectral Total Variation (SSTV), defined using second-order spatio-spectral differences, is widely known as a powerful regularization approach that models the underlying spatio-spectral properties. However, since SSTV refers only to adjacent pixels/bands, semi-local spatial structures are not preserved during denoising process. To address this problem, we newly design S3TTV, defined by the sum of the nuclear norms of matrices consisting of second-order spatio-spectral differences in small spectral blocks (we call these matrices as spatio-spectral structure tensors). The proposed regularization method simultaneously models the spatial piecewise-smoothness, the spatial similarity between adjacent bands, and the spectral correlation across all bands in small spectral blocks, leading to effective noise removal while preserving the semi-local spatial structures. Furthermore, we formulate the HS image denoising and destriping problem as a convex optimization problem involving S3TTV and develop an algorithm based on a preconditioned primal-dual splitting method to solve this problem efficiently. Finally, we demonstrate the effectiveness of S3TTV by comparing it with existing methods, including state-of-the-art ones through denoising and destriping experiments.

Towards Robust Hyperspectral Anomaly Detection: Decomposing Background, Anomaly, and Mixed Noise via Convex Optimization

We propose a novel hyperspectral (HS) anomaly detection method that is robust to various types of noise. Most of existing HS anomaly detection methods are designed for cases where a given HS image is noise-free or is contaminated only by small Gaussian noise. However, in real-world situations, observed HS images are often degraded by various types of noise, such as sparse noise and stripe noise, due to sensor failure or calibration errors, significantly affecting the detection performance. To address this problem, this article establishes a robust HS anomaly detection method with a mechanism that can properly remove mixed noise while separating background and anomaly parts. Specifically, we newly formulate a constrained convex optimization problem to decompose background and anomaly parts, and three types of noise from a given HS image. Then, we develop an efficient algorithm based on a preconditioned variant of a primal-dual splitting method to solve this problem. Through comparison with existing methods, including state-of-the-art ones, we illustrate that the proposed method achieves a detection accuracy comparable to state-of-the-art methods in noise-free cases and is significantly more robust than these methods in noisy cases.

Robust Spatiotemporal Fusion of Satellite Images: A Constrained Convex Optimization Approach

This paper proposes a novel spatiotemporal (ST) fusion framework for satellite images, named Robust Optimization-based Spatiotemporal Fusion (ROSTF). ST fusion is a promising approach to resolve a trade-off between the temporal and spatial resolution of satellite images. Although many ST fusion methods have been proposed, most of them are not designed to explicitly account for noise in observed images, despite the inevitable influence of noise caused by the measurement equipment and environment. Our ROSTF addresses this challenge by treating the noise removal of the observed images and the estimation of the target high-resolution image as a single optimization problem. Specifically, first, we define observation models for satellite images possibly contaminated with random noise, outliers, and/or missing values, and then introduce certain assumptions that would naturally hold between the observed images and the target high-resolution image. Then, based on these models and assumptions, we formulate the fusion problem as a constrained optimization problem and develop an efficient algorithm based on a preconditioned primal-dual splitting method for solving the problem. The performance of ROSTF was verified using simulated and real data. The results show that ROSTF performs comparably to several state-of-the-art ST fusion methods in noiseless cases and outperforms them in noisy cases.

Graph Signal Sampling Under Smoothness Priors: A Difference-of-Convex Approach

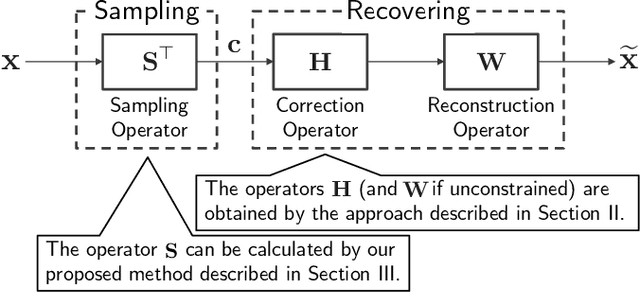
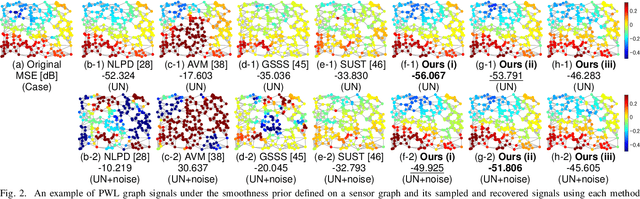
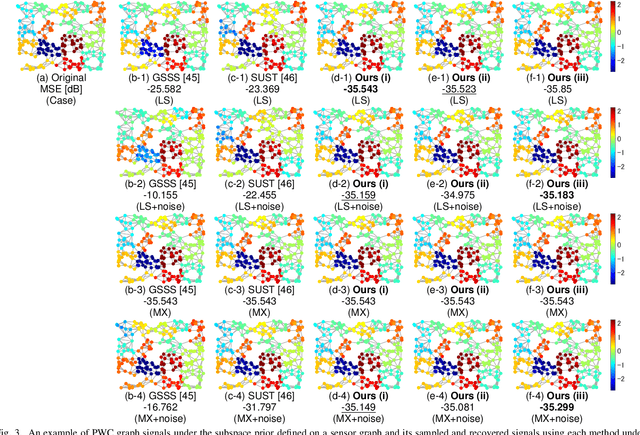
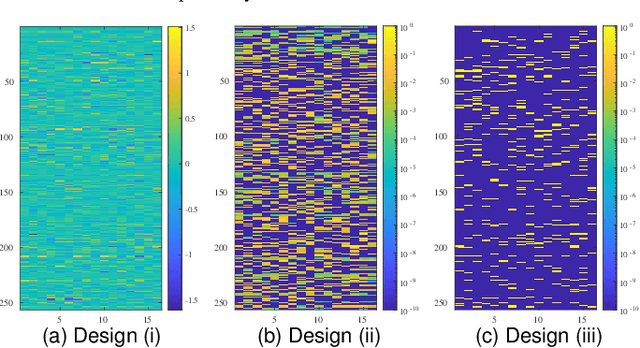
This paper proposes a method for properly sampling graph signals under smoothness priors. Unlike many existing approaches that assume bandlimited graph signals, our method designs a sampling operator for graph signals that are not necessarily bandlimited based on the generalized sampling theory. First, we formulate the sampling operator design under smoothness priors as a feasibility problem. Then, we transform this problem into a Difference-of-Convex (DC) problem by relaxing a certain invertibility constraint in the original problem using the nuclear norm. Furthermore, we develop an efficient algorithm to solve this DC problem based on the proximal linearized difference-of-convex algorithm and guarantee its convergence to a critical point of the problem. Finally, we demonstrate the effectiveness of our method for several graph signal models through sampling-and-reconstruction experiments.

Robust Constrained Hyperspectral Unmixing Using Reconstructed-Image Regularization

Hyperspectral (HS) unmixing is the process of decomposing an HS image into material-specific spectra (endmembers) and their spatial distributions (abundance maps). Existing unmixing methods have two limitations with respect to noise robustness. First, if the input HS image is highly noisy, even if the balance between sparse and piecewise-smooth regularizations for abundance maps is carefully adjusted, noise may remain in the estimated abundance maps or undesirable artifacts may appear. Second, existing methods do not explicitly account for the effects of stripe noise, which is common in HS measurements, in their formulations, resulting in significant degradation of unmixing performance when such noise is present in the input HS image. To overcome these limitations, we propose a new robust hyperspectral unmixing method based on constrained convex optimization. Our method employs, in addition to the two regularizations for the abundance maps, regularizations for the HS image reconstructed by mixing the estimated abundance maps and endmembers. This strategy makes the unmixing process much more robust in highly-noisy scenarios, under the assumption that the abundance maps used to reconstruct the HS image with desirable spatio-spectral structure are also expected to have desirable properties. Furthermore, our method is designed to accommodate a wider variety of noise including stripe noise. To solve the formulated optimization problem, we develop an efficient algorithm based on a preconditioned primal-dual splitting method, which can automatically determine appropriate stepsizes based on the problem structure. Experiments on synthetic and real HS images demonstrate the advantages of our method over existing methods.