Solving the Traveling Salesman Problem via Different Quantum Computing Architectures

We study the application of emerging photonic and quantum computing architectures to solving the Traveling Salesman Problem (TSP), a well-known NP-hard optimization problem. We investigate several approaches: Simulated Annealing (SA), Quadratic Unconstrained Binary Optimization (QUBO-Ising) methods implemented on quantum annealers and Optical Coherent Ising Machines, as well as the Quantum Approximate Optimization Algorithm (QAOA) and the Quantum Phase Estimation (QPE) algorithm on gate-based quantum computers. QAOA and QPE were tested on the IBM Quantum platform. The QUBO-Ising method was explored using the D-Wave quantum annealer, which operates on superconducting Josephson junctions, and the QCI Dirac machine, a nonlinear optoelectronic Ising machine. Gate-based quantum computers demonstrated accurate results for small TSP instances in simulation. However, real quantum devices are hindered by noise and limited scalability. Circuit complexity grows with problem size, restricting performance to TSP instances with a maximum of 6 nodes. In contrast, Ising-based architectures show improved scalability for larger problem sizes. SQUID-based Ising machines can handle TSP instances with up to 12 nodes, while nonlinear optoelectronic Ising machines extend this capability to 18 nodes. Nevertheless, the solutions tend to be suboptimal due to hardware limitations and challenges in achieving ground state convergence as the problem size increases. Despite these limitations, Ising machines demonstrate significant time advantages over classical methods, making them a promising candidate for solving larger-scale TSPs efficiently.

A comparative study of forecasting Corporate Credit Ratings using Neural Networks, Support Vector Machines, and Decision Trees

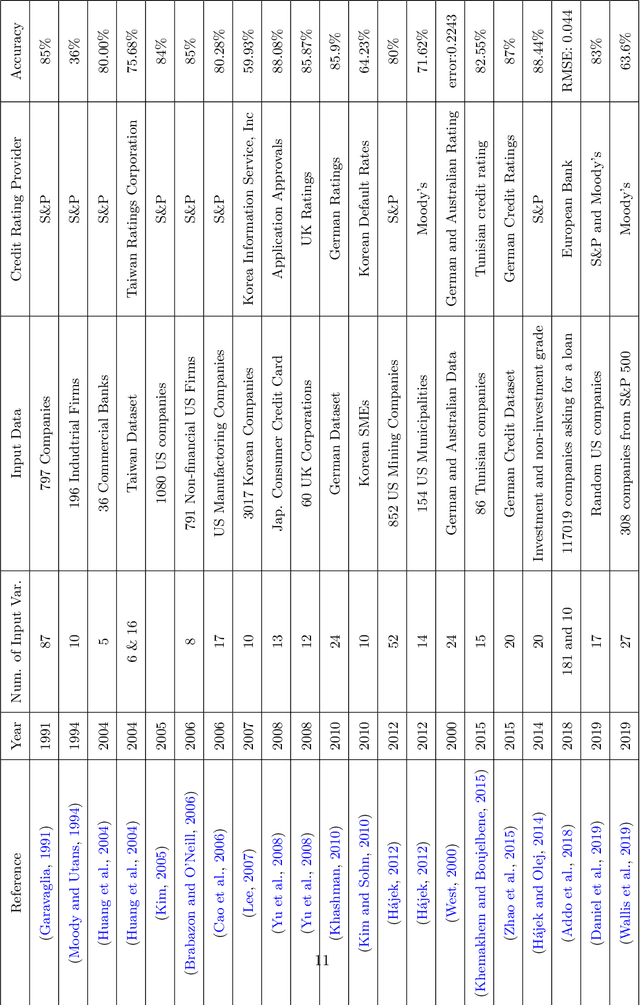
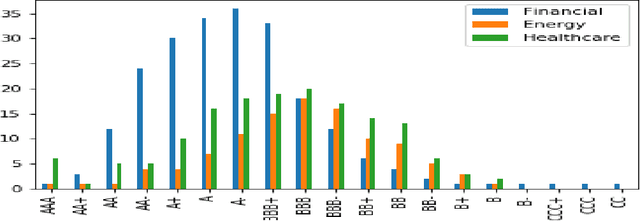
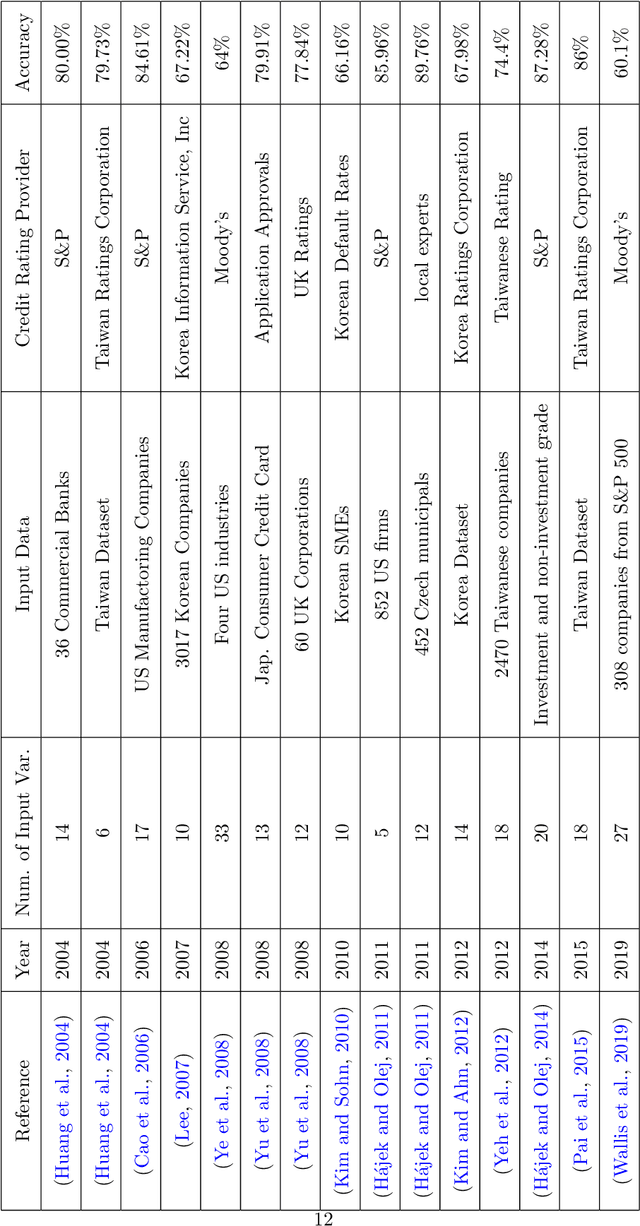
Credit ratings are one of the primary keys that reflect the level of riskiness and reliability of corporations to meet their financial obligations. Rating agencies tend to take extended periods of time to provide new ratings and update older ones. Therefore, credit scoring assessments using artificial intelligence has gained a lot of interest in recent years. Successful machine learning methods can provide rapid analysis of credit scores while updating older ones on a daily time scale. Related studies have shown that neural networks and support vector machines outperform other techniques by providing better prediction accuracy. The purpose of this paper is two fold. First, we provide a survey and a comparative analysis of results from literature applying machine learning techniques to predict credit rating. Second, we apply ourselves four machine learning techniques deemed useful from previous studies (Bagged Decision Trees, Random Forest, Support Vector Machine and Multilayer Perceptron) to the same datasets. We evaluate the results using a 10-fold cross validation technique. The results of the experiment for the datasets chosen show superior performance for decision tree based models. In addition to the conventional accuracy measure of classifiers, we introduce a measure of accuracy based on notches called "Notch Distance" to analyze the performance of the above classifiers in the specific context of credit rating. This measure tells us how far the predictions are from the true ratings. We further compare the performance of three major rating agencies, Standard $\&$ Poors, Moody's and Fitch where we show that the difference in their ratings is comparable with the decision tree prediction versus the actual rating on the test dataset.

Quantum Unsupervised and Supervised Learning on Superconducting Processors

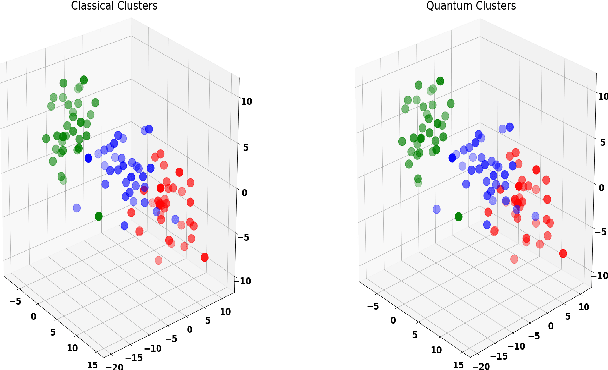
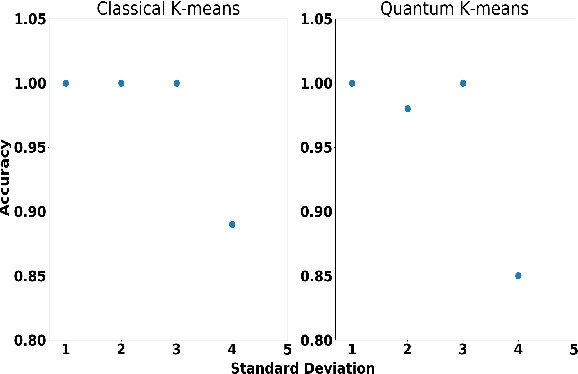
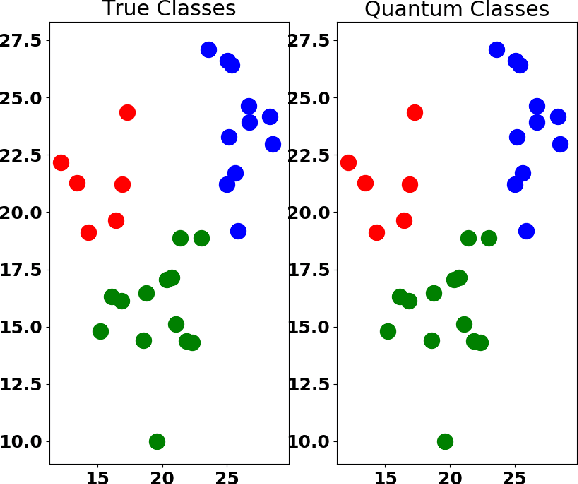
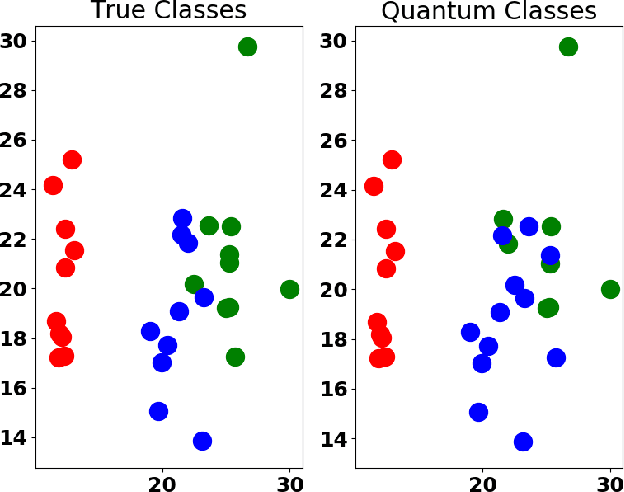
Machine learning algorithms perform well on identifying patterns in many datasets due to their versatility. However, as one increases the size of the data, the time for training and using these statistical models grows quickly. Here, we propose and implement on the IBMQ a quantum analogue to K-means clustering, and compare it to a previously developed quantum support vector machine. We find the algorithm's accuracy comparable to classical K-means for clustering and classification problems, and find that it becomes less computationally expensive to implement for large datasets.