Intuitionistic Linear Temporal Logics

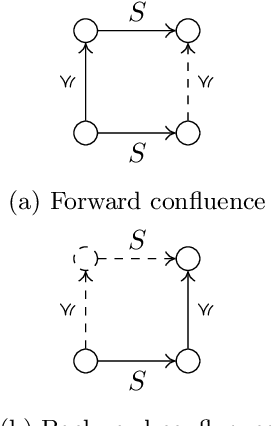
We consider intuitionistic variants of linear temporal logic with `next', `until' and `release' based on expanding posets: partial orders equipped with an order-preserving transition function. This class of structures gives rise to a logic which we denote $\iltl$, and by imposing additional constraints we obtain the logics $\itlb$ of persistent posets and $\itlht$ of here-and-there temporal logic, both of which have been considered in the literature. We prove that $\iltl$ has the effective finite model property and hence is decidable, while $\itlb$ does not have the finite model property. We also introduce notions of bounded bisimulations for these logics and use them to show that the `until' and `release' operators are not definable in terms of each other, even over the class of persistent posets.

Exploring the bidimensional space: A dynamic logic point of view

We present a family of logics for reasoning about agents' positions and motion in the plane which have several potential applications in the area of multi-agent systems (MAS), such as multi-agent planning and robotics. The most general logic includes (i) atomic formulas for representing the truth of a given fact or the presence of a given agent at a certain position of the plane, (ii) atomic programs corresponding to the four basic orientations in the plane (up, down, left, right) as well as the four program constructs of propositional dynamic logic (sequential composition, nondeterministic composition, iteration and test). As this logic is not computably enumerable, we study some interesting decidable and axiomatizable fragments of it. We also present a decidable extension of the iteration-free fragment of the logic by special programs representing motion of agents in the plane.