Transferable Deep Learning Power System Short-Term Voltage Stability Assessment with Physics-Informed Topological Feature Engineering

Deep learning (DL) algorithms have been widely applied to short-term voltage stability (STVS) assessment in power systems. However, transferring the knowledge learned in one power grid to other power grids with topology changes is still a challenging task. This paper proposed a transferable DL-based model for STVS assessment by constructing the topology-aware voltage dynamic features from raw PMU data. Since the reactive power flow and grid topology are essential to voltage stability, the topology-aware and physics-informed voltage dynamic features are utilized to effectively represent the topological and temporal patterns from post-disturbance system dynamic trajectories. The proposed DL-based STVS assessment model is tested under random operating conditions on the New England 39-bus system. It has 99.99\% classification accuracy of the short-term voltage stability status using the topology-aware and physics-informed voltage dynamic features. In addition to high accuracy, the experiments show good adaptability to PMU errors. Moreover, The proposed STVS assessment method has outstanding performance on new grid topologies after fine-tuning. In particular, the highest accuracy reaches 99.68\% in evaluation, which demonstrates a good knowledge transfer ability of the proposed model for power grid topology change.

Fast Transient Stability Prediction Using Grid-informed Temporal and Topological Embedding Deep Neural Network

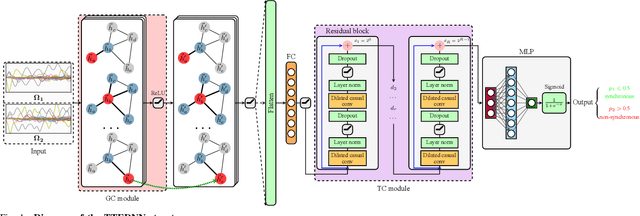
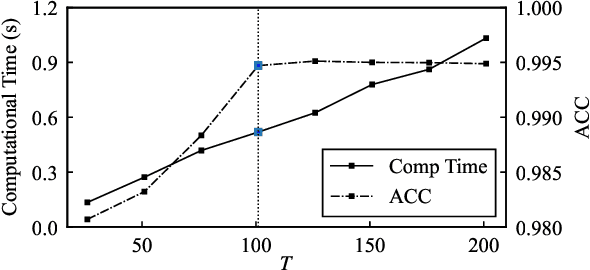
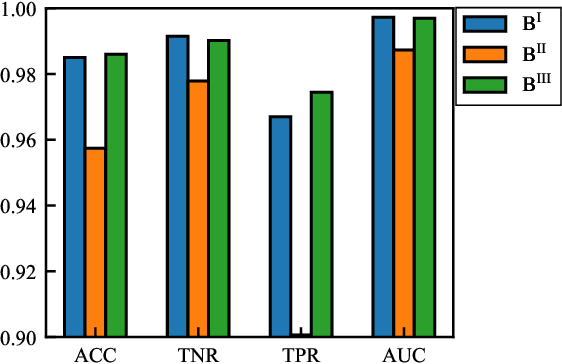
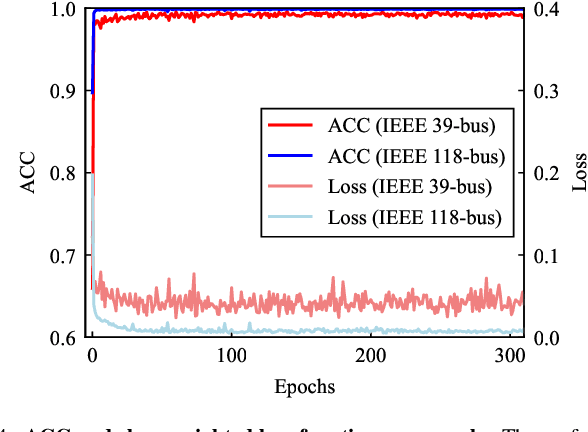
Transient stability prediction is critically essential to the fast online assessment and maintaining the stable operation in power systems. The wide deployment of phasor measurement units (PMUs) promotes the development of data-driven approaches for transient stability assessment. This paper proposes the temporal and topological embedding deep neural network (TTEDNN) model to forecast transient stability with the early transient dynamics. The TTEDNN model can accurately and efficiently predict the transient stability by extracting the temporal and topological features from the time-series data of the early transient dynamics. The grid-informed adjacency matrix is used to incorporate the power grid structural and electrical parameter information. The transient dynamics simulation environments under the single-node and multiple-node perturbations are used to test the performance of the TTEDNN model for the IEEE 39-bus and IEEE 118-bus power systems. The results show that the TTEDNN model has the best and most robust prediction performance. Furthermore, the TTEDNN model also demonstrates the transfer capability to predict the transient stability in the more complicated transient dynamics simulation environments.

Hyperbolic Multiplex Network Embedding with Maps of Random Walk

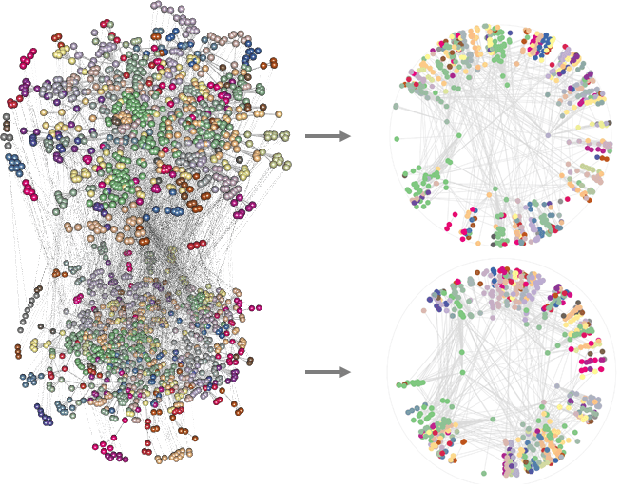
Recent research on network embedding in hyperbolic space have proven successful in several applications. However, nodes in real world networks tend to interact through several distinct channels. Simple aggregation or ignorance of this multiplexity will lead to misleading results. On the other hand, there exists redundant information between different interaction patterns between nodes. Recent research reveals the analogy between the community structure and the hyperbolic coordinate. To learn each node's effective embedding representation while reducing the redundancy of multiplex network, we then propose a unified framework combing multiplex network hyperbolic embedding and multiplex community detection. The intuitive rationale is that high order node embedding approach is expected to alleviate the observed network's sparse and noisy structure which will benefit the community detection task. On the contrary, the improved community structure will also guide the node embedding task. To incorporate the common features between channels while preserving unique features, a random walk approach which traversing in latent multiplex hyperbolic space is proposed to detect the community across channels and bridge the connection between node embedding and community detection. The proposed framework is evaluated on several network tasks using different real world dataset. The results demonstrates that our framework is effective and efficiency compared with state-of-the-art approaches.

Arena Model: Inference About Competitions

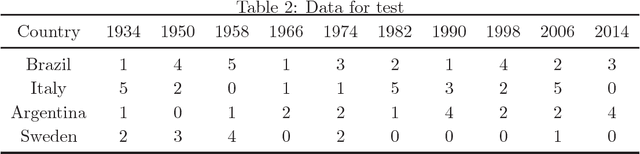
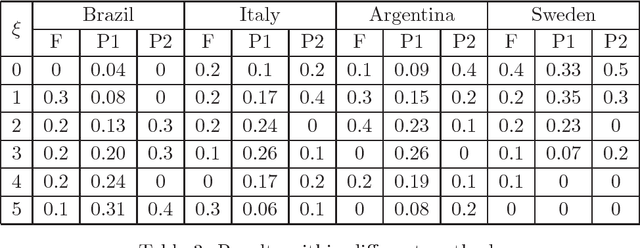
The authors propose a parametric model called the arena model for prediction in paired competitions, i.e. paired comparisons with eliminations and bifurcations. The arena model has a number of appealing advantages. First, it predicts the results of competitions without rating many individuals. Second, it takes full advantage of the structure of competitions. Third, the model provides an easy method to quantify the uncertainty in competitions. Fourth, some of our methods can be directly generalized for comparisons among three or more individuals. Furthermore, the authors identify an invariant Bayes estimator with regard to the prior distribution and prove the consistency of the estimations of uncertainty. Currently, the arena model is not effective in tracking the change of strengths of individuals, but its basic framework provides a solid foundation for future study of such cases.