On multipolar magnetic anomaly detection: multipolar signal subspaces, an analytical orthonormal basis, multipolar truncature and detection performance

In this paper, we consider the magnetic anomaly detection problem which aims to find hidden ferromagnetic masses by estimating the weak perturbation they induce on local Earth's magnetic field. We consider classical detection schemes that rely on signals recorded on a moving sensor, and modeling of the source as a function of unknown parameters. As the usual spherical harmonic decomposition of the anomaly has to be truncated in practice, we study the signal vector subspaces induced by each multipole of the decomposition, proving they are not in direct sum, and discussing the impact it has on the choice of the truncation order. Further, to ease the detection strategy based on generalized likelihood ratio test, we rely on orthogonal polynomials theory to derive an analytical set of orthonormal functions (multipolar orthonormal basis functions) that spans the space of the noise-free measured signal. Finally, based on the subspace structure of the multipole vector spaces, we study the impact of the truncation order on the detection performance, beyond the issue of potential surparametrization, and the behaviour of the information criteria used to choose this order.

Initialization Free Graph Based Clustering

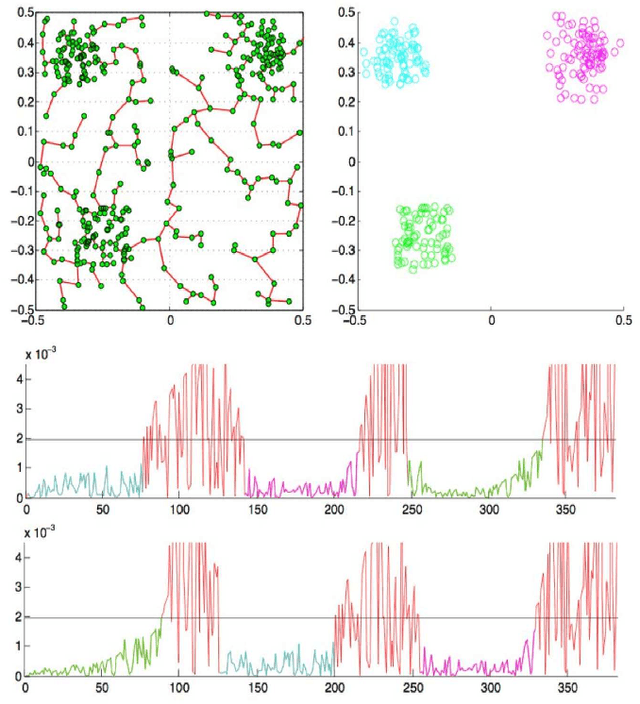
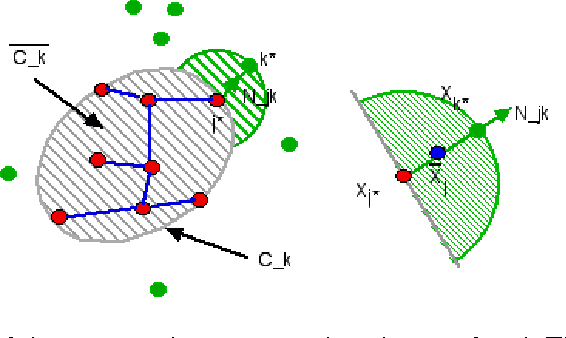
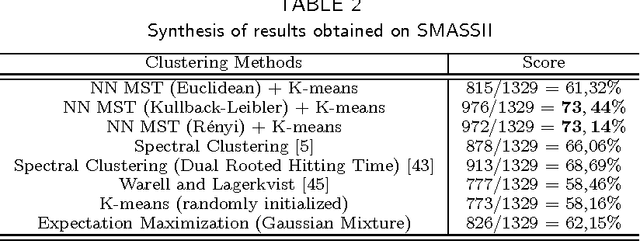
This paper proposes an original approach to cluster multi-component data sets, including an estimation of the number of clusters. From the construction of a minimal spanning tree with Prim's algorithm, and the assumption that the vertices are approximately distributed according to a Poisson distribution, the number of clusters is estimated by thresholding the Prim's trajectory. The corresponding cluster centroids are then computed in order to initialize the generalized Lloyd's algorithm, also known as $K$-means, which allows to circumvent initialization problems. Some results are derived for evaluating the false positive rate of our cluster detection algorithm, with the help of approximations relevant in Euclidean spaces. Metrics used for measuring similarity between multi-dimensional data points are based on symmetrical divergences. The use of these informational divergences together with the proposed method leads to better results, compared to other clustering methods for the problem of astrophysical data processing. Some applications of this method in the multi/hyper-spectral imagery domain to a satellite view of Paris and to an image of the Mars planet are also presented. In order to demonstrate the usefulness of divergences in our problem, the method with informational divergence as similarity measure is compared with the same method using classical metrics. In the astrophysics application, we also compare the method with the spectral clustering algorithms.