Niranjan Saikumar
Time regularization as a solution to mitigate quantization induced performance degradation
Oct 30, 2020
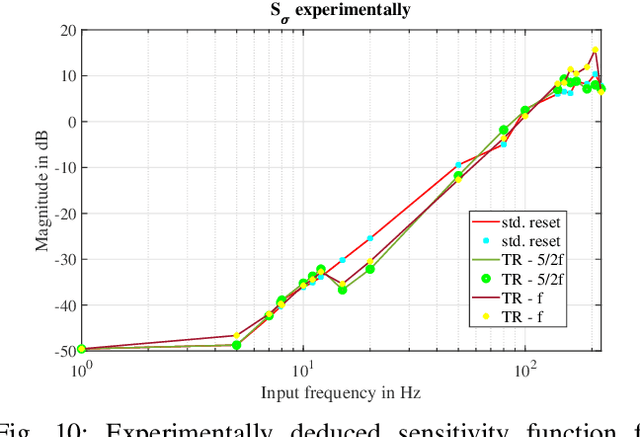
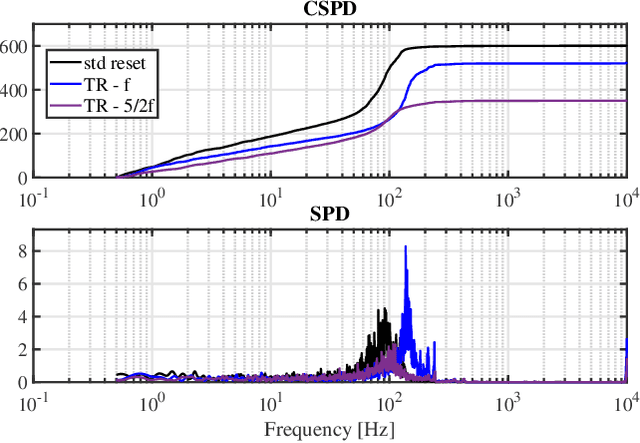
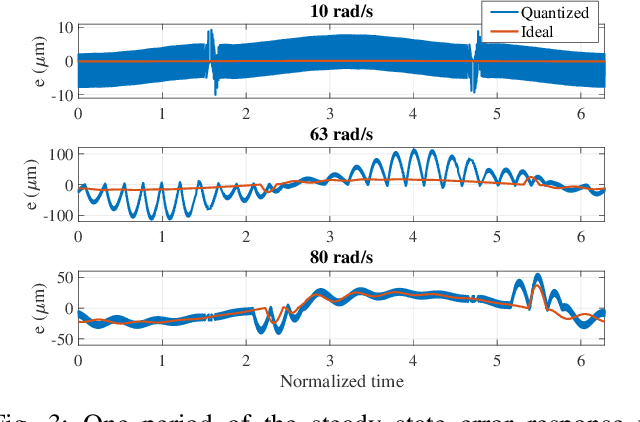
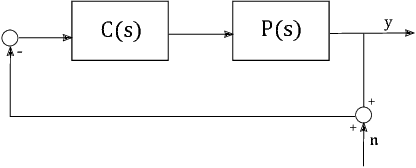
Abstract:Reset control is known to be able to outperform PID and the like linear controllers. However, in motion control systems, quantization can cause severe performance degradation. This paper shows the application of time regularization to mitigate this practical issue in reset control systems. Numerical simulations have been conducted in order to analyze the cause of the quantization induced performance degradation and the effectiveness of time regularization to mitigate this degradation; with tuning guidelines for the time regularization parameter also provided. Moreover, a robustness analysis is performed. The solution is also tested experimentally on a high precision motion system for validation. It is estimated by numerical simulations that time regularization can reduce quantization induced performance degradation by almost 10 dB. Experiments have similarly shown a reduction of several dB for the high precision motion stage.
Reset band for mitigatation of quantization induced performance degradation
Oct 30, 2020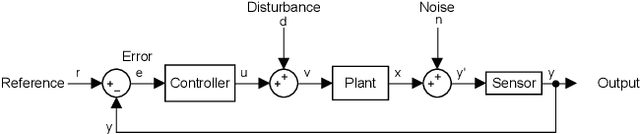


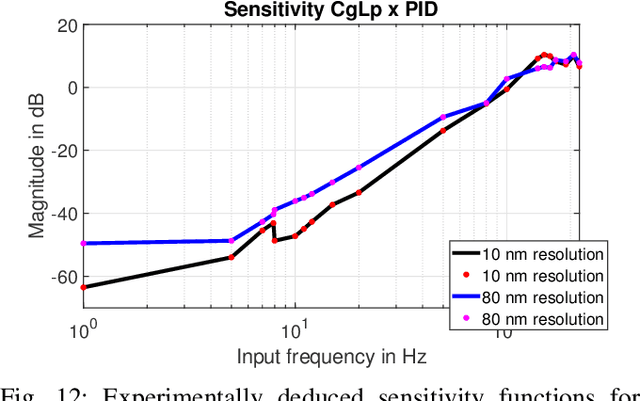
Abstract:Reset control has emerged as a viable alternative to popular PID, capable of outperforming and overcoming the linear limitations. However, in motion control systems, quantization can cause severe performance degradation. This paper investigates this degradation in practical systems and re-purposes the reset band condition in order to mitigate the same. Numerical simulations have been conducted on a mass based positioning system to analyze the cause of the quantization induced performance degradation. Moreover, a performance and robustness analysis was performed with the proposed solution. Finally, novel tuning guidelines are provided for estimating the required reset band parameter. Additionally, practical experiments have been conducted on a high precision motion system for validation. The experiments show by example that the reset band can reduce the error in the problematic region by up to 285% and hence shows the need and effectiveness of the proposed solution.
Adaptive Feedforward Control For Reset Feedback Control Systems -- Application in Precision Motion Control
Oct 30, 2020

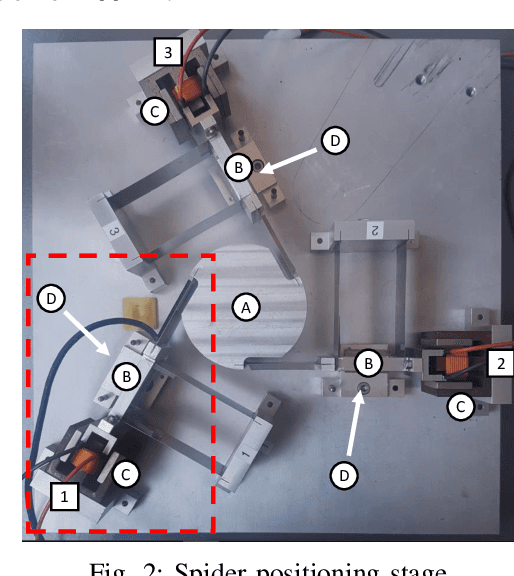
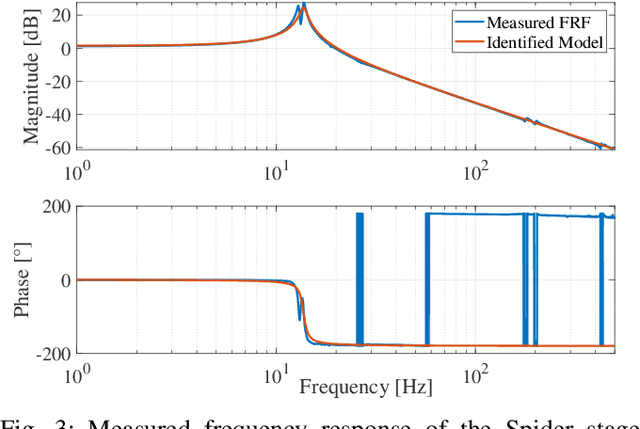
Abstract:This paper presents a novel adaptive feedforward controller design for reset control systems. The combination of feedforward and reset feedback control promises high performance as the feedforward guarantees reference tracking, while the non-linear feedback element rejects disturbances. To overcome inevitable model mismatches, the feedforward controller adapts to increase precision in reference tracking. Where linear existing adaptive feedforward controllers do not guarantee convergence in the presence of reset, this work presents a novel adaptive law based on converging and diverging regions of adaptation to achieve good tracking. Experimental results demonstrate the claimed advantage of the novel method.
Loop-shaping for reset control systems -- A higher-order sinusoidal-input describing functions approach
Aug 25, 2020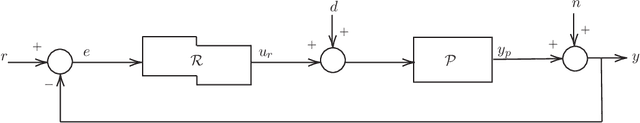

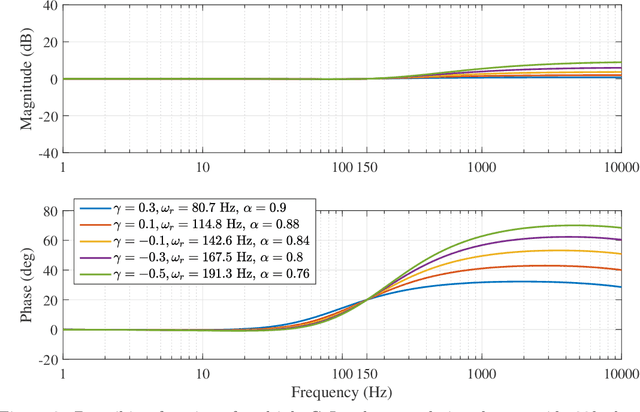

Abstract:The ever-growing demands on speed and precision from the precision motion industry have pushed control requirements to reach the limitations of linear control theory. Nonlinear controllers like reset provide a viable alternative since they can be easily integrated into the existing linear controller structure and designed using industry-preferred loop-shaping techniques. However, currently, loop-shaping is achieved using the describing function (DF) and performance analysed using linear control sensitivity functions not applicable for reset control systems, resulting in a significant deviation between expected and practical results. We overcome this major bottleneck to the wider adaptation of reset control with two contributions in this paper. First, we present the extension of frequency-domain tools for reset controllers in the form of higher-order sinusoidal-input describing functions (HOSIDFs) providing greater insight into their behaviour. Second, we propose a novel method which uses the DF and HOSIDFs of the open-loop reset control system for the estimation of the closed-loop sensitivity functions, establishing for the first time - the relation between open-loop and closed-loop behaviour of reset control systems in the frequency domain. The accuracy of the proposed solution is verified in both simulation and practice on a precision positioning stage and these results are further analysed to obtain insights into the tuning considerations for reset controllers.
No More Differentiator in PID:Development of Nonlinear Lead for Precision Mechatronics
May 24, 2018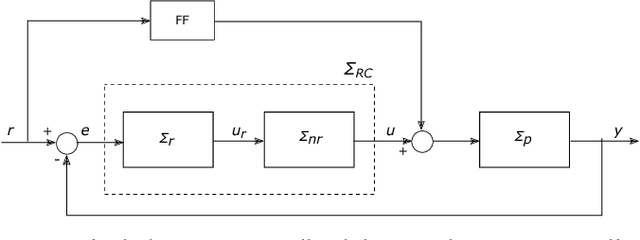


Abstract:Industrial PID consists of three elements: Lag (integrator), Lead (Differentiator) and Low Pass Filters (LPF). PID being a linear control method is inherently bounded by the waterbed effect due to which there exists a trade-off between precision \& tracking, provided by Lag and LPF on one side and stability \& robustness, provided by Lead on the other side. Nonlinear reset strategies applied in Lag and LPF elements have been very effective in reducing this trade-off. However, there is lack of study in developing a reset Lead element. In this paper, we develop a novel lead element which provides higher precision and stability compared to the linear lead filter and can be used as a replacement for the same. The concept is presented and validated on a Lorentz-actuated nanometer precision stage. Improvements in precision, tracking and bandwidth are shown through two separate designs. Performance is validated in both time and frequency domain to ensure that phase margin achieved on the practical setup matches design theories.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge