Bilevel Learning with Inexact Stochastic Gradients

Bilevel learning has gained prominence in machine learning, inverse problems, and imaging applications, including hyperparameter optimization, learning data-adaptive regularizers, and optimizing forward operators. The large-scale nature of these problems has led to the development of inexact and computationally efficient methods. Existing adaptive methods predominantly rely on deterministic formulations, while stochastic approaches often adopt a doubly-stochastic framework with impractical variance assumptions, enforces a fixed number of lower-level iterations, and requires extensive tuning. In this work, we focus on bilevel learning with strongly convex lower-level problems and a nonconvex sum-of-functions in the upper-level. Stochasticity arises from data sampling in the upper-level which leads to inexact stochastic hypergradients. We establish their connection to state-of-the-art stochastic optimization theory for nonconvex objectives. Furthermore, we prove the convergence of inexact stochastic bilevel optimization under mild assumptions. Our empirical results highlight significant speed-ups and improved generalization in imaging tasks such as image denoising and deblurring in comparison with adaptive deterministic bilevel methods.

An Adaptively Inexact Method for Bilevel Learning Using Primal-Dual Style Differentiation

We consider a bilevel learning framework for learning linear operators. In this framework, the learnable parameters are optimized via a loss function that also depends on the minimizer of a convex optimization problem (denoted lower-level problem). We utilize an iterative algorithm called `piggyback' to compute the gradient of the loss and minimizer of the lower-level problem. Given that the lower-level problem is solved numerically, the loss function and thus its gradient can only be computed inexactly. To estimate the accuracy of the computed hypergradient, we derive an a-posteriori error bound, which provides guides for setting the tolerance for the lower-level problem, as well as the piggyback algorithm. To efficiently solve the upper-level optimization, we also propose an adaptive method for choosing a suitable step-size. To illustrate the proposed method, we consider a few learned regularizer problems, such as training an input-convex neural network.

Dynamic Bilevel Learning with Inexact Line Search

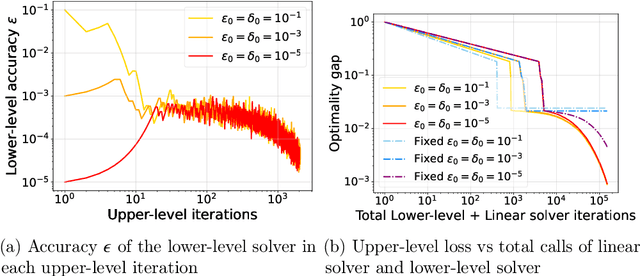
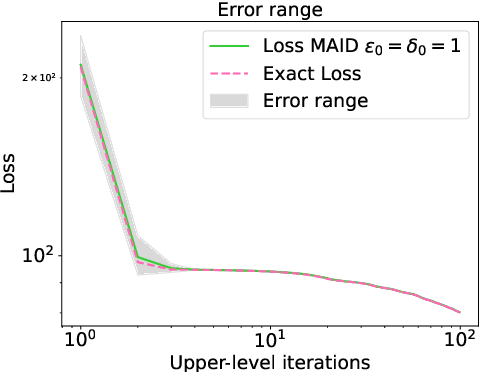
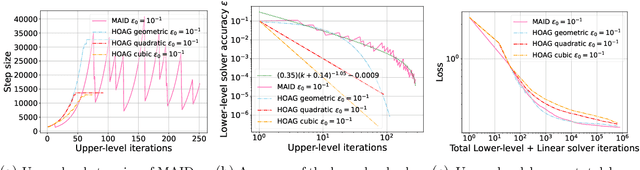
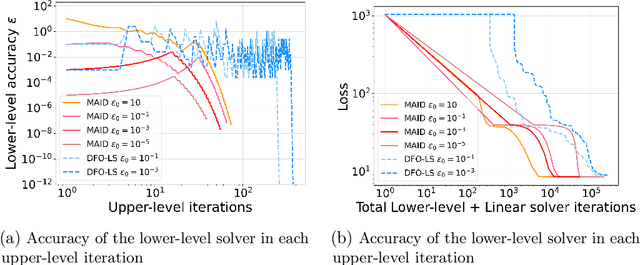
In various domains within imaging and data science, particularly when addressing tasks modeled utilizing the variational regularization approach, manually configuring regularization parameters presents a formidable challenge. The difficulty intensifies when employing regularizers involving a large number of hyperparameters. To overcome this challenge, bilevel learning is employed to learn suitable hyperparameters. However, due to the use of numerical solvers, the exact gradient with respect to the hyperparameters is unattainable, necessitating the use of methods relying on approximate gradients. State-of-the-art inexact methods a priori select a decreasing summable sequence of the required accuracy and only assure convergence given a sufficiently small fixed step size. Despite this, challenges persist in determining the Lipschitz constant of the hypergradient and identifying an appropriate fixed step size. Conversely, computing exact function values is not feasible, impeding the use of line search. In this work, we introduce a provably convergent inexact backtracking line search involving inexact function evaluations and hypergradients. We show convergence to a stationary point of the loss with respect to hyperparameters. Additionally, we propose an algorithm to determine the required accuracy dynamically. Our numerical experiments demonstrate the efficiency and feasibility of our approach for hyperparameter estimation in variational regularization problems, alongside its robustness in terms of the initial accuracy and step size choices.