Quasi-Newton OMP Approach for Super-Resolution Channel Estimation and Extrapolation

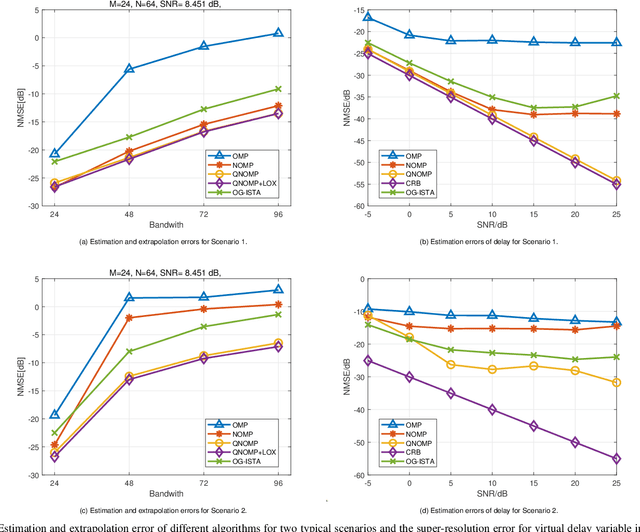
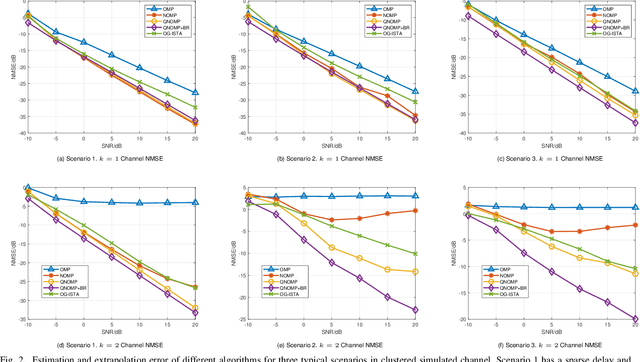
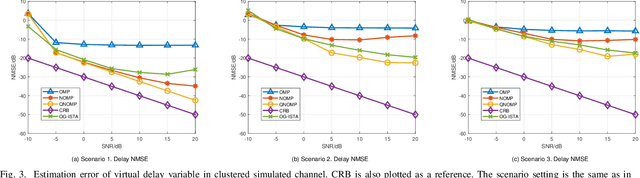
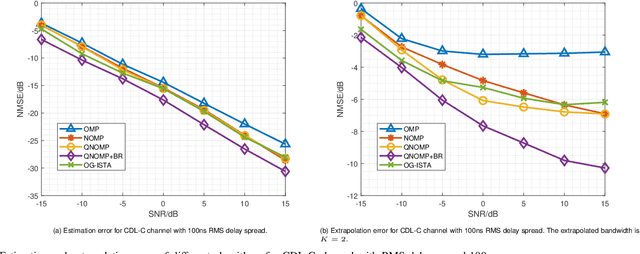
Channel estimation and extrapolation are fundamental issues in MIMO communication systems. In this paper, we proposed the quasi-Newton orthogonal matching pursuit (QNOMP) approach to overcome these issues with high efficiency while maintaining accuracy. The algorithm consists of two stages on the super-resolution recovery: we first performed a cheap on-grid OMP estimation of channel parameters in the sparsity domain (e.g., delay or angle), then an off-grid optimization to achieve the super-resolution. In the off-grid stage, we employed the BFGS quasi-Newton method to jointly estimate the parameters through a multipath model, which improved the speed and accuracy significantly. Furthermore, we derived the optimal extrapolated solution in the linear minimum mean squared estimator criterion, revealed its connection with Slepian basis, and presented a practical algorithm to realize the extrapolation based on the QNOMP results. Special treatment utilizing the block sparsity nature of the considered channels was also proposed. Numerical experiments on the simulated models and CDL-C channels demonstrated the high performance and low computational complexity of QNOMP.

Dynamic Adaptive Resource Scheduling for Phased Array Radar: Enhancing Efficiency through Synthesis Priorities and Pulse Interleaving

To enhance the resource scheduling performance of phased array radar, we propose a dynamic adaptive resource scheduling algorithm based on synthesis priorities and pulse interleaving. This approach addresses the challenges of low efficiency, high loss ratios, and significant subjectivity in task assignment within phased array radar systems. We introduce a task synthesis priority design method that considers the working mode priority, deadlines, and time shift ratios. By implementing this method, we can increase the flexibility of task scheduling and improve the efficiency of radar processing tasks. Additionally, our proposed pulse interleaving method effectively utilizes the waiting periods between receiving and transmitting pulses to process other beams, thereby enhancing resource utilization. Simulation results demonstrate that the proposed scheduling algorithm significantly reduces time deviation ratios and scheduling failure rates while improving scheduling yield and time utilization ratios.

DMRA: An Adaptive Line Spectrum Estimation Method through Dynamical Multi-Resolution of Atoms

We proposed a novel dense line spectrum super-resolution algorithm, the DMRA, that leverages dynamical multi-resolution of atoms technique to address the limitation of traditional compressed sensing methods when handling dense point-source signals. The algorithm utilizes a smooth $\tanh$ relaxation function to replace the $\ell_0$ norm, promoting sparsity and jointly estimating the frequency atoms and complex gains. To reduce computational complexity and improve frequency estimation accuracy, a two-stage strategy was further introduced to dynamically adjust the number of the optimized degrees of freedom. The strategy first increases candidate frequencies through local refinement, then applies a sparse selector to eliminate insignificant frequencies, thereby adaptively adjusting the degrees of freedom to improve estimation accuracy. Theoretical analysis were provided to validate the proposed method for multi-parameter estimations. Computational results demonstrated that this algorithm achieves good super-resolution performance in various practical scenarios and outperforms the state-of-the-art methods in terms of frequency estimation accuracy and computational efficiency.