Learning Differential Equations that are Easy to Solve

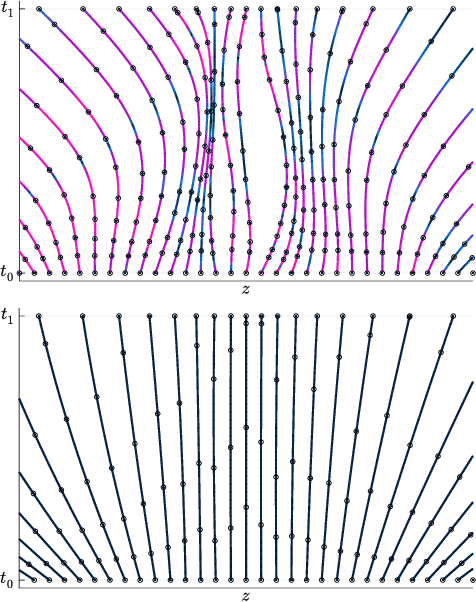
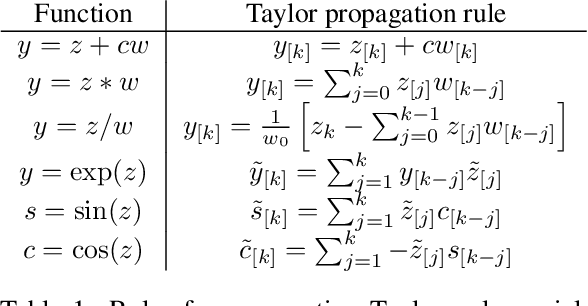
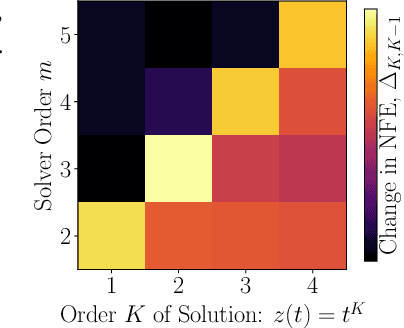
Differential equations parameterized by neural networks become expensive to solve numerically as training progresses. We propose a remedy that encourages learned dynamics to be easier to solve. Specifically, we introduce a differentiable surrogate for the time cost of standard numerical solvers, using higher-order derivatives of solution trajectories. These derivatives are efficient to compute with Taylor-mode automatic differentiation. Optimizing this additional objective trades model performance against the time cost of solving the learned dynamics. We demonstrate our approach by training substantially faster, while nearly as accurate, models in supervised classification, density estimation, and time-series modelling tasks.

Patterns of Scalable Bayesian Inference

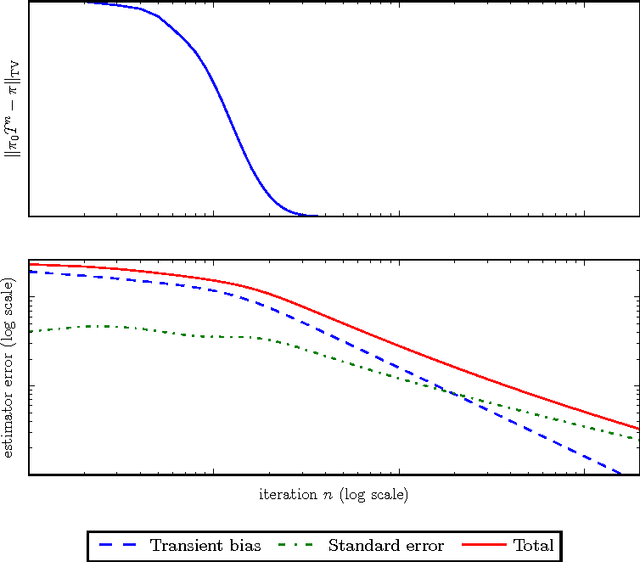
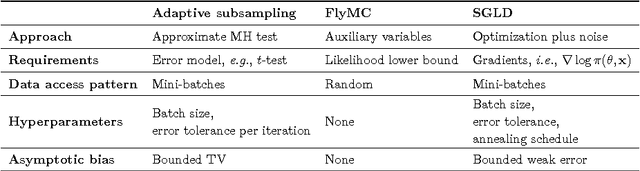
Datasets are growing not just in size but in complexity, creating a demand for rich models and quantification of uncertainty. Bayesian methods are an excellent fit for this demand, but scaling Bayesian inference is a challenge. In response to this challenge, there has been considerable recent work based on varying assumptions about model structure, underlying computational resources, and the importance of asymptotic correctness. As a result, there is a zoo of ideas with few clear overarching principles. In this paper, we seek to identify unifying principles, patterns, and intuitions for scaling Bayesian inference. We review existing work on utilizing modern computing resources with both MCMC and variational approximation techniques. From this taxonomy of ideas, we characterize the general principles that have proven successful for designing scalable inference procedures and comment on the path forward.

A Simple Explanation of A Spectral Algorithm for Learning Hidden Markov Models

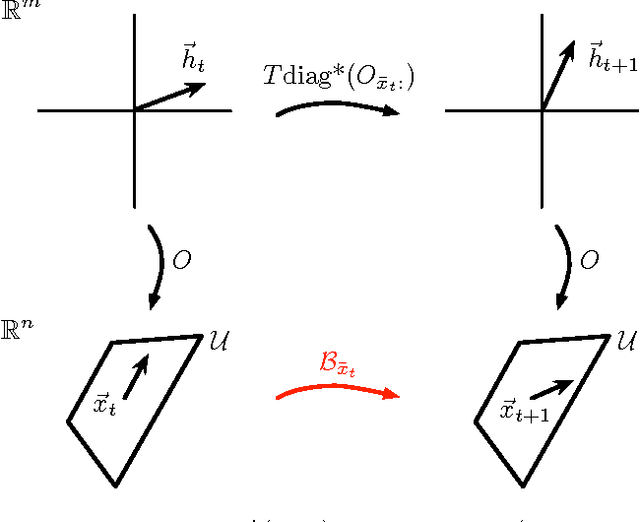
A simple linear algebraic explanation of the algorithm in "A Spectral Algorithm for Learning Hidden Markov Models" (COLT 2009). Most of the content is in Figure 2; the text just makes everything precise in four nearly-trivial claims.