Decomposition During Search for Propagation-Based Constraint Solvers

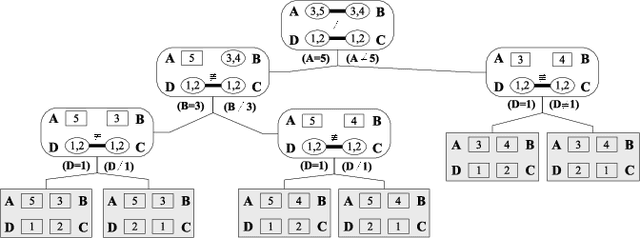
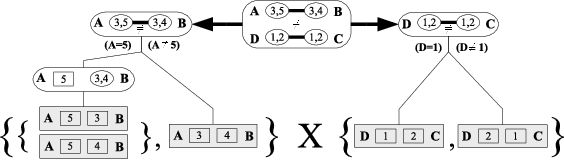
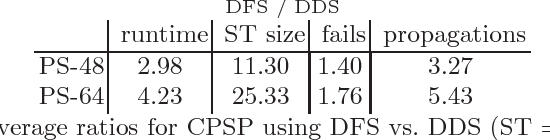
We describe decomposition during search (DDS), an integration of And/Or tree search into propagation-based constraint solvers. The presented search algorithm dynamically decomposes sub-problems of a constraint satisfaction problem into independent partial problems, avoiding redundant work. The paper discusses how DDS interacts with key features that make propagation-based solvers successful: constraint propagation, especially for global constraints, and dynamic search heuristics. We have implemented DDS for the Gecode constraint programming library. Two applications, solution counting in graph coloring and protein structure prediction, exemplify the benefits of DDS in practice.

Decomposition Techniques for Subgraph Matching

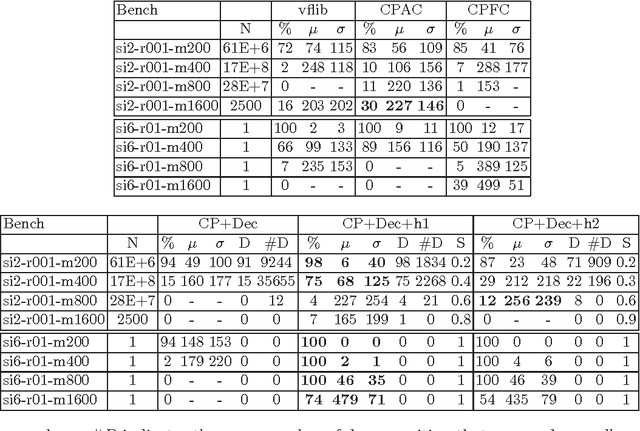
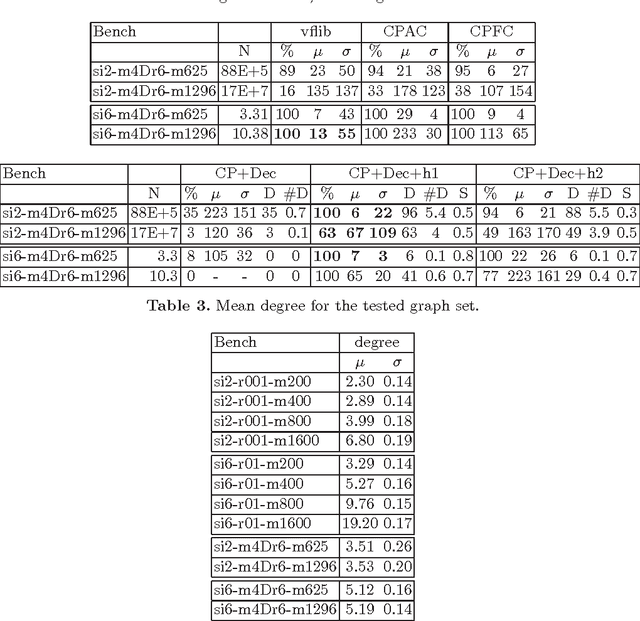
In the constraint programming framework, state-of-the-art static and dynamic decomposition techniques are hard to apply to problems with complete initial constraint graphs. For such problems, we propose a hybrid approach of these techniques in the presence of global constraints. In particular, we solve the subgraph isomorphism problem. Further we design specific heuristics for this hard problem, exploiting its special structure to achieve decomposition. The underlying idea is to precompute a static heuristic on a subset of its constraint network, to follow this static ordering until a first problem decomposition is available, and to switch afterwards to a fully propagated, dynamically decomposing search. Experimental results show that, for sparse graphs, our decomposition method solves more instances than dedicated, state-of-the-art matching algorithms or standard constraint programming approaches.