Finite sample learning of moving targets

We consider a moving target that we seek to learn from samples. Our results extend randomized techniques developed in control and optimization for a constant target to the case where the target is changing. We derive a novel bound on the number of samples that are required to construct a probably approximately correct (PAC) estimate of the target. Furthermore, when the moving target is a convex polytope, we provide a constructive method of generating the PAC estimate using a mixed integer linear program (MILP). The proposed method is demonstrated on an application to autonomous emergency braking.

Sampling-based optimal kinodynamic planning with motion primitives

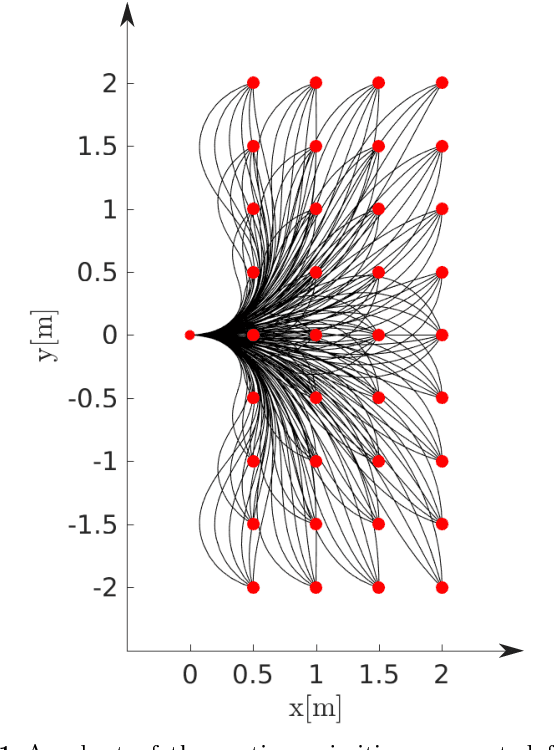
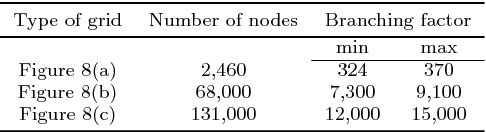
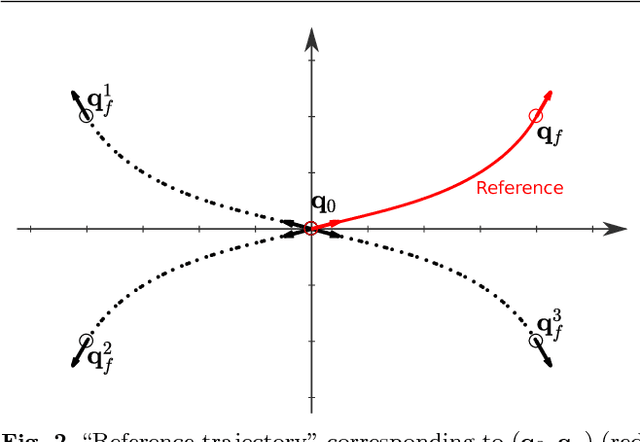
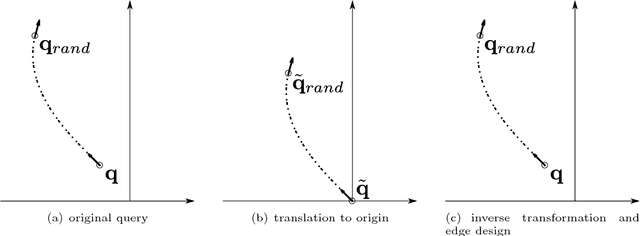
This paper proposes a novel sampling-based motion planner, which integrates in RRT* (Rapidly exploring Random Tree star) a database of pre-computed motion primitives to alleviate its computational load and allow for motion planning in a dynamic or partially known environment. The database is built by considering a set of initial and final state pairs in some grid space, and determining for each pair an optimal trajectory that is compatible with the system dynamics and constraints, while minimizing a cost. Nodes are progressively added to the tree {of feasible trajectories in the RRT* by extracting at random a sample in the gridded state space and selecting the best obstacle-free motion primitive in the database that joins it to an existing node. The tree is rewired if some nodes can be reached from the new sampled state through an obstacle-free motion primitive with lower cost. The computationally more intensive part of motion planning is thus moved to the preliminary offline phase of the database construction at the price of some performance degradation due to gridding. Grid resolution can be tuned so as to compromise between (sub)optimality and size of the database. The planner is shown to be asymptotically optimal as the grid resolution goes to zero and the number of sampled states grows to infinity.

Randomised Algorithm for Feature Selection and Classification

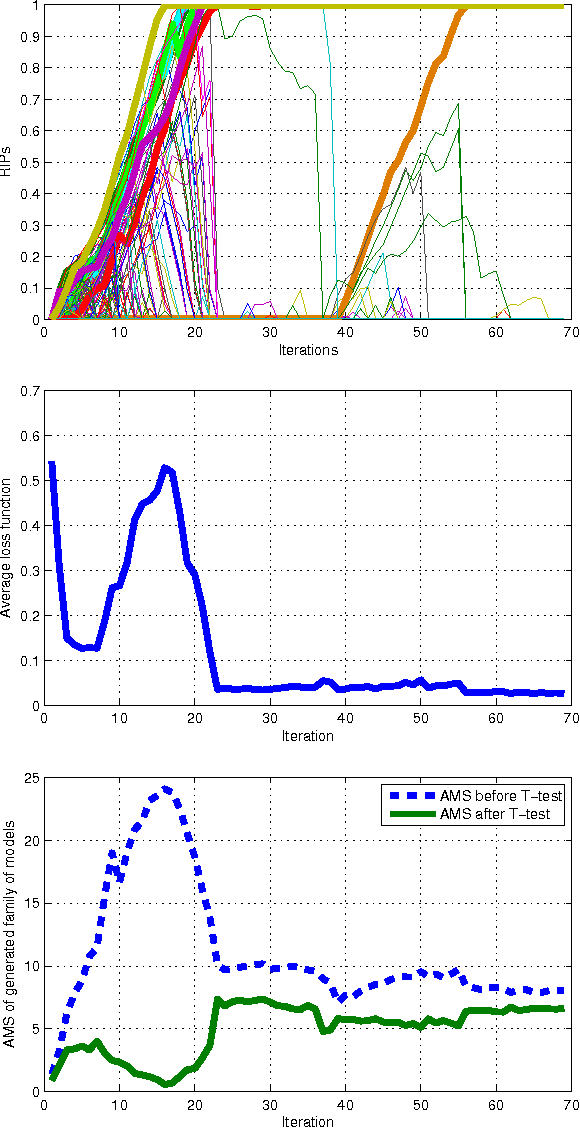
We here introduce a novel classification approach adopted from the nonlinear model identification framework, which jointly addresses the feature selection and classifier design tasks. The classifier is constructed as a polynomial expansion of the original attributes and a model structure selection process is applied to find the relevant terms of the model. The selection method progressively refines a probability distribution defined on the model structure space, by extracting sample models from the current distribution and using the aggregate information obtained from the evaluation of the population of models to reinforce the probability of extracting the most important terms. To reduce the initial search space, distance correlation filtering can be applied as a preprocessing technique. The proposed method is evaluated and compared to other well-known feature selection and classification methods on standard benchmark classification problems. The results show the effectiveness of the proposed method with respect to competitor methods both in terms of classification accuracy and model complexity. The obtained models have a simple structure, easily amenable to interpretation and analysis.