Injective Flows for parametric hypersurfaces

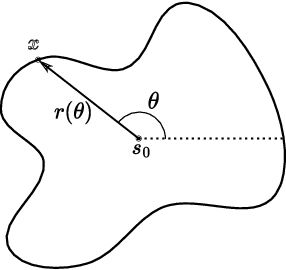
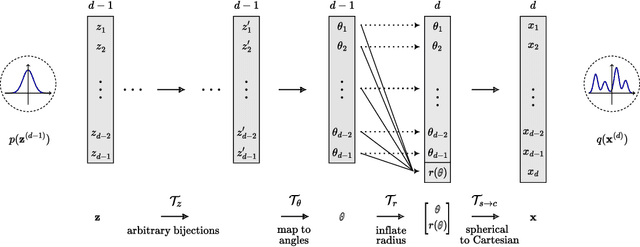
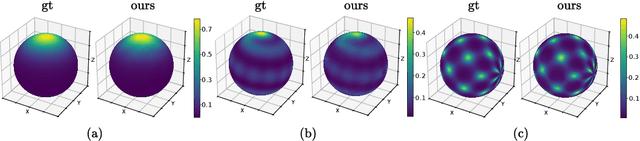
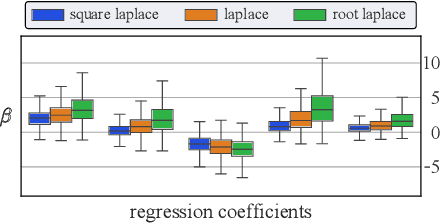
Normalizing Flows (NFs) are powerful and efficient models for density estimation. When modeling densities on manifolds, NFs can be generalized to injective flows but the Jacobian determinant becomes computationally prohibitive. Current approaches either consider bounds on the log-likelihood or rely on some approximations of the Jacobian determinant. In contrast, we propose injective flows for parametric hypersurfaces and show that for such manifolds we can compute the Jacobian determinant exactly and efficiently, with the same cost as NFs. Furthermore, we show that for the subclass of star-like manifolds we can extend the proposed framework to always allow for a Cartesian representation of the density. We showcase the relevance of modeling densities on hypersurfaces in two settings. Firstly, we introduce a novel Objective Bayesian approach to penalized likelihood models by interpreting level-sets of the penalty as star-like manifolds. Secondly, we consider Bayesian mixture models and introduce a general method for variational inference by defining the posterior of mixture weights on the probability simplex.

Conditional Matrix Flows for Gaussian Graphical Models

Studying conditional independence structure among many variables with few observations is a challenging task. Gaussian Graphical Models (GGMs) tackle this problem by encouraging sparsity in the precision matrix through an $l_p$ regularization with $p\leq1$. However, since the objective is highly non-convex for sub-$l_1$ pseudo-norms, most approaches rely on the $l_1$ norm. In this case frequentist approaches allow to elegantly compute the solution path as a function of the shrinkage parameter $\lambda$. Instead of optimizing the penalized likelihood, the Bayesian formulation introduces a Laplace prior on the precision matrix. However, posterior inference for different $\lambda$ values requires repeated runs of expensive Gibbs samplers. We propose a very general framework for variational inference in GGMs that unifies the benefits of frequentist and Bayesian frameworks. Specifically, we propose to approximate the posterior with a matrix-variate Normalizing Flow defined on the space of symmetric positive definite matrices. As a key improvement on previous work, we train a continuum of sparse regression models jointly for all regularization parameters $\lambda$ and all $l_p$ norms, including non-convex sub-$l_1$ pseudo-norms. This is achieved by conditioning the flow on $p>0$ and on the shrinkage parameter $\lambda$. We have then access with one model to (i) the evolution of the posterior for any $\lambda$ and for any $l_p$ (pseudo-) norms, (ii) the marginal log-likelihood for model selection, and (iii) we can recover the frequentist solution paths as the MAP, which is obtained through simulated annealing.

Lagrangian Flow Networks for Conservation Laws

We introduce Lagrangian Flow Networks (LFlows) for modeling fluid densities and velocities continuously in space and time. The proposed LFlows satisfy by construction the continuity equation, a PDE describing mass conservation in its differentiable form. Our model is based on the insight that solutions to the continuity equation can be expressed as time-dependent density transformations via differentiable and invertible maps. This follows from classical theory of existence and uniqueness of Lagrangian flows for smooth vector fields. Hence, we model fluid densities by transforming a base density with parameterized diffeomorphisms conditioned on time. The key benefit compared to methods relying on Neural-ODE or PINNs is that the analytic expression of the velocity is always consistent with the density. Furthermore, there is no need for expensive numerical solvers, nor for enforcing the PDE with penalty methods. Lagrangian Flow Networks show improved predictive accuracy on synthetic density modeling tasks compared to competing models in both 2D and 3D. We conclude with a real-world application of modeling bird migration based on sparse weather radar measurements.

Truly Mesh-free Physics-Informed Neural Networks

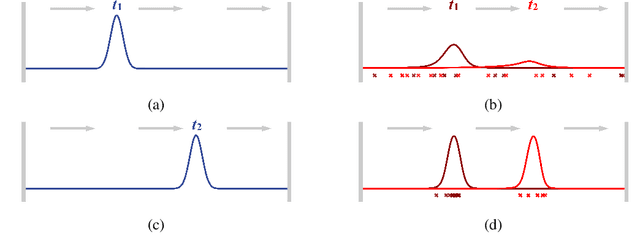
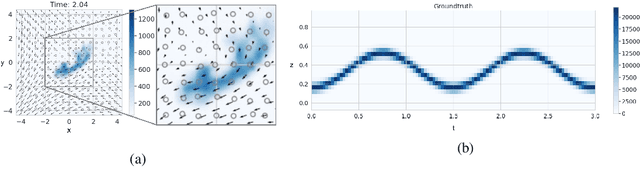
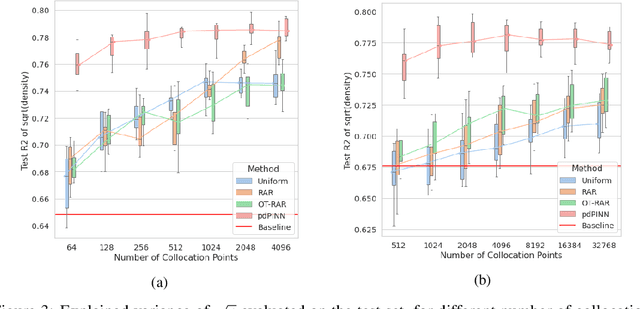
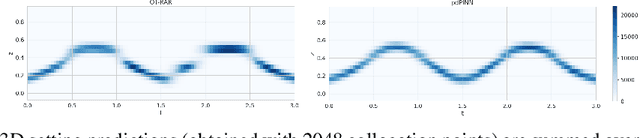
Physics-informed Neural Networks (PINNs) have recently emerged as a principled way to include prior physical knowledge in form of partial differential equations (PDEs) into neural networks. Although generally viewed as being mesh-free, current approaches still rely on collocation points obtained within a bounded region, even in settings with spatially sparse signals. Furthermore, if the boundaries are not known, the selection of such a region may be arbitrary, resulting in a large proportion of collocation points being selected in areas of low relevance. To resolve this, we present a mesh-free and adaptive approach termed particle-density PINN (pdPINN), which is inspired by the microscopic viewpoint of fluid dynamics. Instead of sampling from a bounded region, we propose to sample directly from the distribution over the (fluids) particle positions, eliminating the need to introduce boundaries while adaptively focusing on the most relevant regions. This is achieved by reformulating the modeled fluid density as an unnormalized probability distribution from which we sample with dynamic Monte Carlo methods. We further generalize pdPINNs to different settings that allow interpreting a positive scalar quantity as a particle density, such as the evolution of the temperature in the heat equation. The utility of our approach is demonstrated on experiments for modeling (non-steady) compressible fluids in up to three dimensions and a two-dimensional diffusion problem, illustrating the high flexibility and sample efficiency compared to existing refinement methods for PINNs.