The Geometry of Polynomial Group Convolutional Neural Networks

We study polynomial group convolutional neural networks (PGCNNs) for an arbitrary finite group $G$. In particular, we introduce a new mathematical framework for PGCNNs using the language of graded group algebras. This framework yields two natural parametrizations of the architecture, based on Hadamard and Kronecker products, related by a linear map. We compute the dimension of the associated neuromanifold, verifying that it depends only on the number of layers and the size of the group. We also describe the general fiber of the Kronecker parametrization up to the regular group action and rescaling, and conjecture the analogous description for the Hadamard parametrization. Our conjecture is supported by explicit computations for small groups and shallow networks.

Learning Size and Shape of Calabi-Yau Spaces

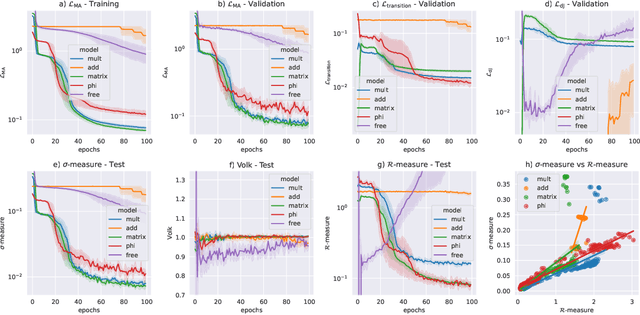
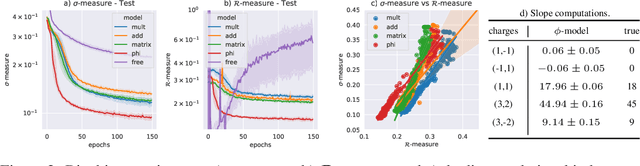
We present a new machine learning library for computing metrics of string compactification spaces. We benchmark the performance on Monte-Carlo sampled integrals against previous numerical approximations and find that our neural networks are more sample- and computation-efficient. We are the first to provide the possibility to compute these metrics for arbitrary, user-specified shape and size parameters of the compact space and observe a linear relation between optimization of the partial differential equation we are training against and vanishing Ricci curvature.

Explore and Exploit with Heterotic Line Bundle Models

We use deep reinforcement learning to explore a class of heterotic $SU(5)$ GUT models constructed from line bundle sums over Complete Intersection Calabi Yau (CICY) manifolds. We perform several experiments where A3C agents are trained to search for such models. These agents significantly outperform random exploration, in the most favourable settings by a factor of 1700 when it comes to finding unique models. Furthermore, we find evidence that the trained agents also outperform random walkers on new manifolds. We conclude that the agents detect hidden structures in the compactification data, which is partly of general nature. The experiments scale well with $h^{(1,1)}$, and may thus provide the key to model building on CICYs with large $h^{(1,1)}$.