Luca Pinello
Robust And Scalable Learning Of Complex Dataset Topologies Via Elpigraph
Jun 20, 2018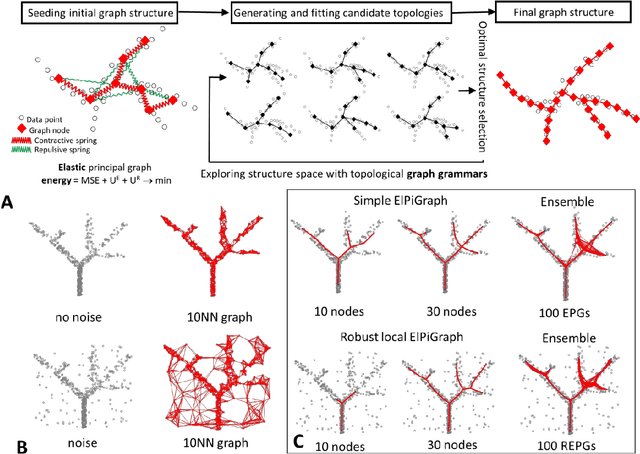
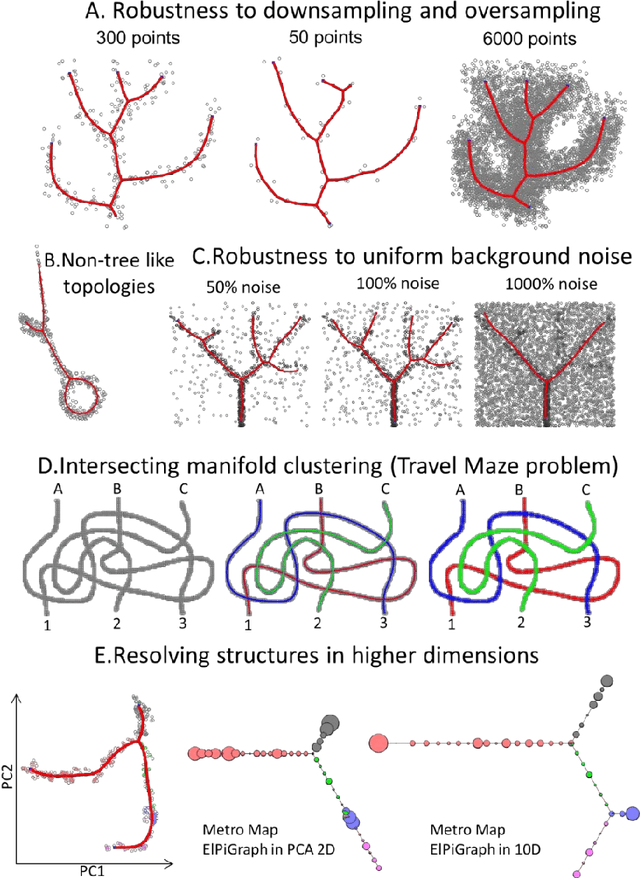
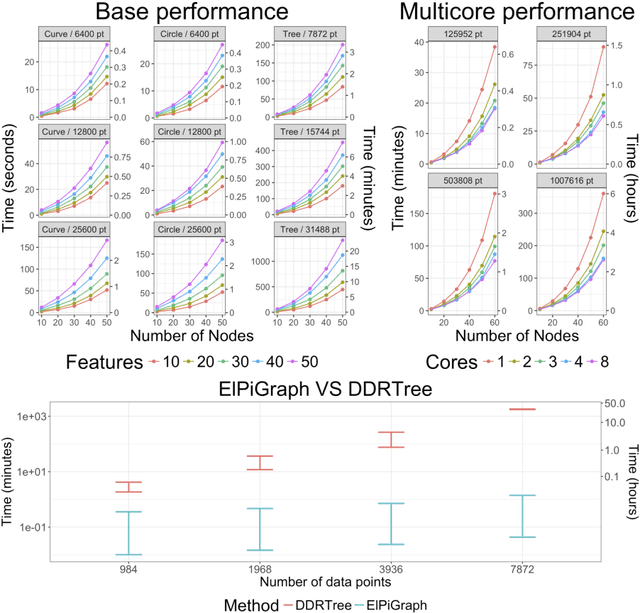
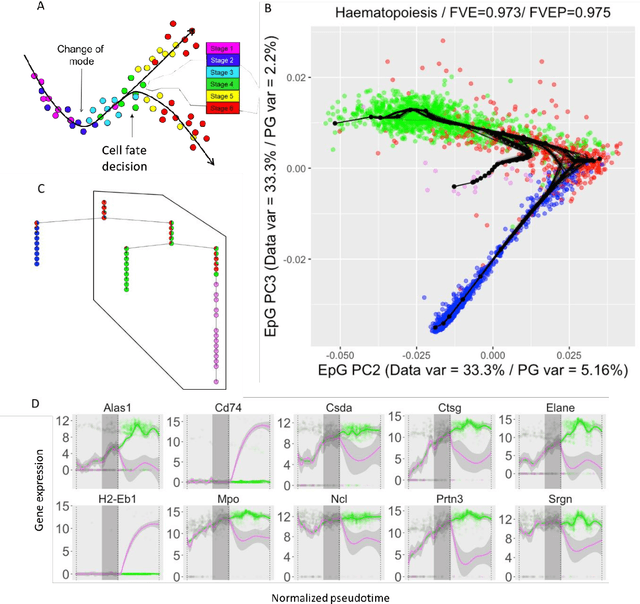
Abstract:Large datasets represented by multidimensional data point clouds often possess non-trivial distributions with branching trajectories and excluded regions, with the recent single-cell transcriptomic studies of developing embryo being notable examples. Reducing the complexity and producing compact and interpretable representations of such data remains a challenging task. Most of the existing computational methods are based on exploring the local data point neighbourhood relations, a step that can perform poorly in the case of multidimensional and noisy data. Here we present ElPiGraph, a scalable and robust method for approximation of datasets with complex structures which does not require computing the complete data distance matrix or the data point neighbourhood graph. This method is able to withstand high levels of noise and is capable of approximating complex topologies via principal graph ensembles that can be combined into a consensus principal graph. ElPiGraph deals efficiently with large and complex datasets in various fields from biology, where it can be used to infer gene dynamics from single-cell RNA-Seq, to astronomy, where it can be used to explore complex structures in the distribution of galaxies.
Multi Layer Analysis
Jul 26, 2011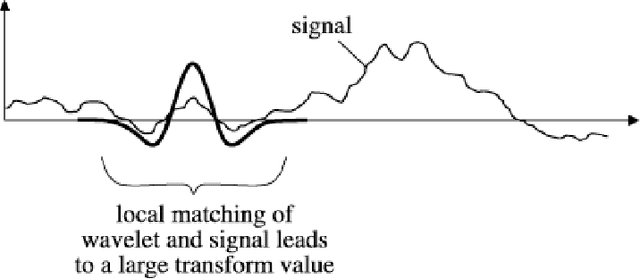
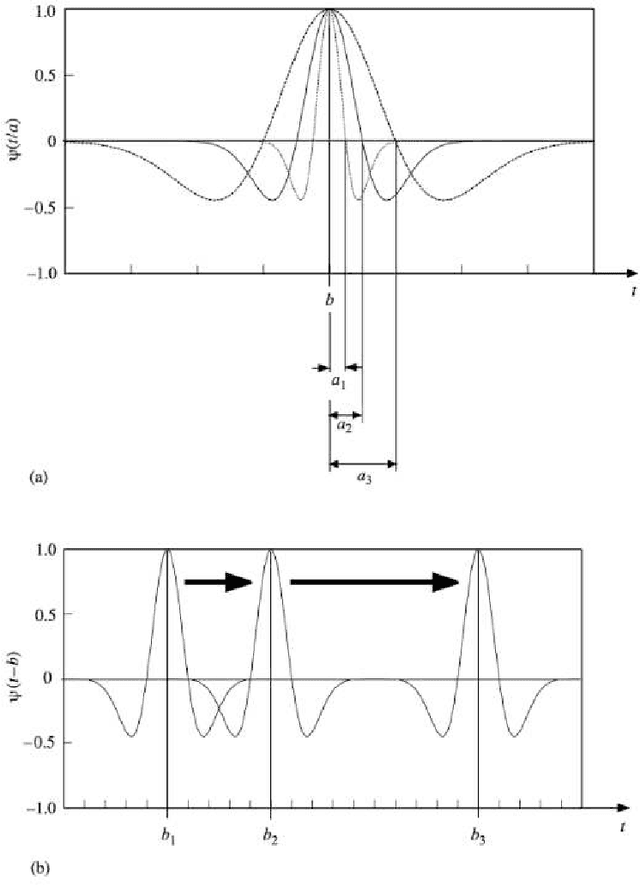
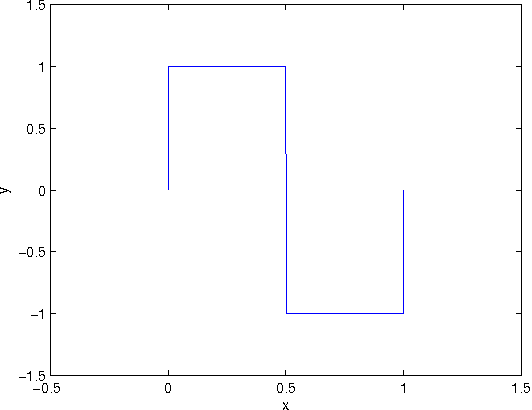
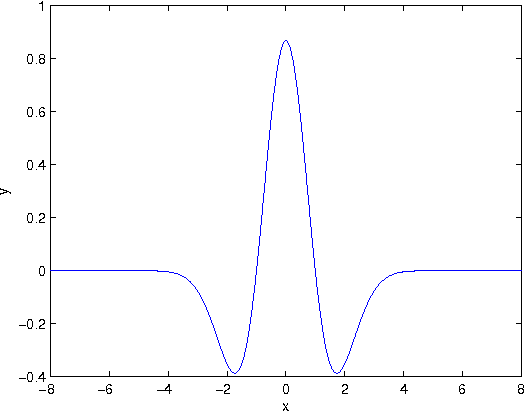
Abstract:This thesis presents a new methodology to analyze one-dimensional signals trough a new approach called Multi Layer Analysis, for short MLA. It also provides some new insights on the relationship between one-dimensional signals processed by MLA and tree kernels, test of randomness and signal processing techniques. The MLA approach has a wide range of application to the fields of pattern discovery and matching, computational biology and many other areas of computer science and signal processing. This thesis includes also some applications of this approach to real problems in biology and seismology.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge