Ligen Shi
Iterative approach to reconstructing neural disparity fields from light-field data
Jul 22, 2024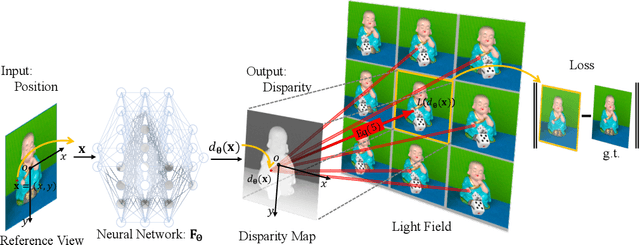
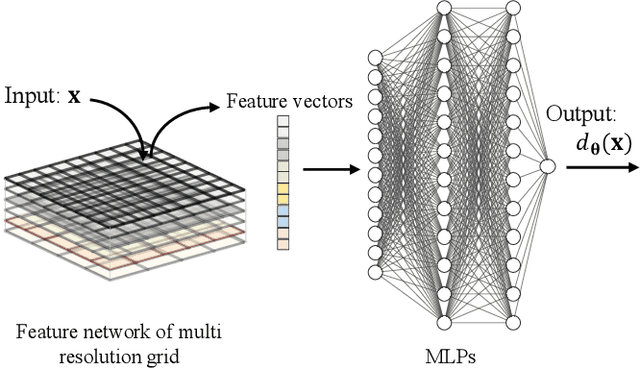
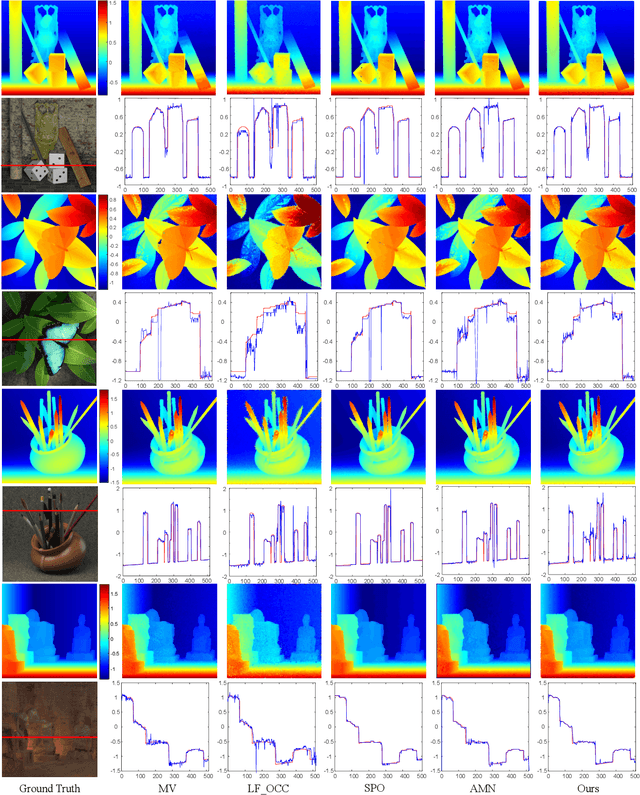
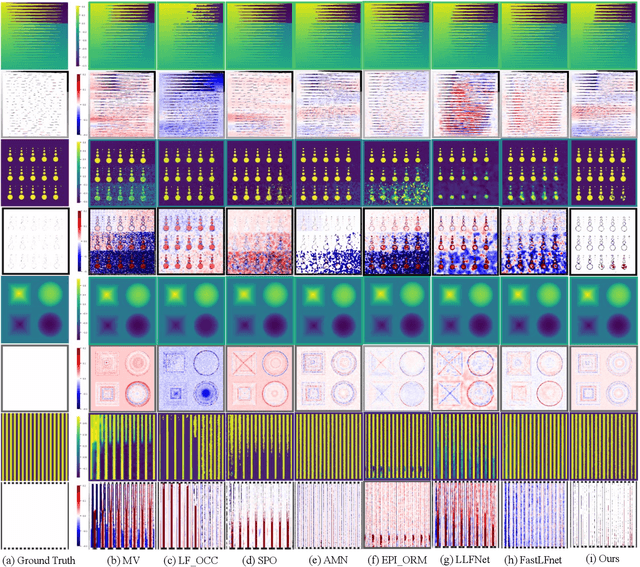
Abstract:This study proposes a neural disparity field (NDF) that establishes an implicit, continuous representation of scene disparity based on a neural field and an iterative approach to address the inverse problem of NDF reconstruction from light-field data. NDF enables seamless and precise characterization of disparity variations in three-dimensional scenes and can discretize disparity at any arbitrary resolution, overcoming the limitations of traditional disparity maps that are prone to sampling errors and interpolation inaccuracies. The proposed NDF network architecture utilizes hash encoding combined with multilayer perceptrons to capture detailed disparities in texture levels, thereby enhancing its ability to represent the geometric information of complex scenes. By leveraging the spatial-angular consistency inherent in light-field data, a differentiable forward model to generate a central view image from the light-field data is developed. Based on the forward model, an optimization scheme for the inverse problem of NDF reconstruction using differentiable propagation operators is established. Furthermore, an iterative solution method is adopted to reconstruct the NDF in the optimization scheme, which does not require training datasets and applies to light-field data captured by various acquisition methods. Experimental results demonstrate that high-quality NDF can be reconstructed from light-field data using the proposed method. High-resolution disparity can be effectively recovered by NDF, demonstrating its capability for the implicit, continuous representation of scene disparities.
Ray-driven Spectral CT Reconstruction Based on Neural Base-Material Fields
Apr 10, 2024Abstract:In spectral CT reconstruction, the basis materials decomposition involves solving a large-scale nonlinear system of integral equations, which is highly ill-posed mathematically. This paper proposes a model that parameterizes the attenuation coefficients of the object using a neural field representation, thereby avoiding the complex calculations of pixel-driven projection coefficient matrices during the discretization process of line integrals. It introduces a lightweight discretization method for line integrals based on a ray-driven neural field, enhancing the accuracy of the integral approximation during the discretization process. The basis materials are represented as continuous vector-valued implicit functions to establish a neural field parameterization model for the basis materials. The auto-differentiation framework of deep learning is then used to solve the implicit continuous function of the neural base-material fields. This method is not limited by the spatial resolution of reconstructed images, and the network has compact and regular properties. Experimental validation shows that our method performs exceptionally well in addressing the spectral CT reconstruction. Additionally, it fulfils the requirements for the generation of high-resolution reconstruction images.
Matching entropy based disparity estimation from light field
Oct 28, 2022Abstract:A major challenge for matching-based depth estimation is to prevent mismatches in occlusion and smooth regions. An effective matching window satisfying three characteristics: texture richness, disparity consistency and anti-occlusion should be able to prevent mismatches to some extent. According to these characteristics, we propose matching entropy in the spatial domain of light field to measure the amount of correct information in a matching window, which provides the criterion for matching window selection. Based on matching entropy regularization, we establish an optimization model for depth estimation with a matching cost fidelity term. To find the optimum, we propose a two-step adaptive matching algorithm. First, the region type is adaptively determined to identify occluding, occluded, smooth and textured regions. Then, the matching entropy criterion is used to adaptively select the size and shape of matching windows, as well as the visible viewpoints. The two-step process can reduce mismatches and redundant calculations by selecting effective matching windows. The experimental results on synthetic and real data show that the proposed method can effectively improve the accuracy of depth estimation in occlusion and smooth regions and has strong robustness for different noise levels. Therefore, high-precision depth estimation from 4D light field data is achieved.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge