The AL$\ell_0$CORE Tensor Decomposition for Sparse Count Data

This paper introduces AL$\ell_0$CORE, a new form of probabilistic non-negative tensor decomposition. AL$\ell_0$CORE is a Tucker decomposition where the number of non-zero elements (i.e., the $\ell_0$-norm) of the core tensor is constrained to a preset value $Q$ much smaller than the size of the core. While the user dictates the total budget $Q$, the locations and values of the non-zero elements are latent variables and allocated across the core tensor during inference. AL$\ell_0$CORE -- i.e., $allo$cated $\ell_0$-$co$nstrained $core$-- thus enjoys both the computational tractability of CP decomposition and the qualitatively appealing latent structure of Tucker. In a suite of real-data experiments, we demonstrate that AL$\ell_0$CORE typically requires only tiny fractions (e.g.,~1%) of the full core to achieve the same results as full Tucker decomposition at only a correspondingly tiny fraction of the cost.

Model-assisted deep learning of rare extreme events from partial observations

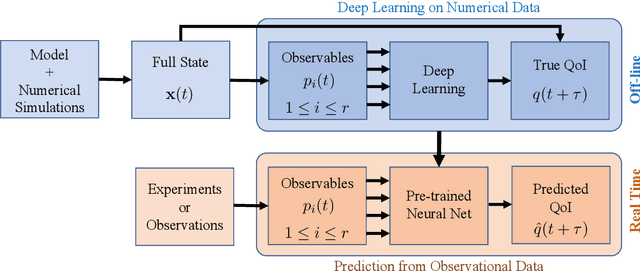
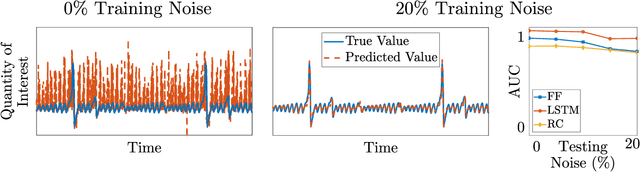
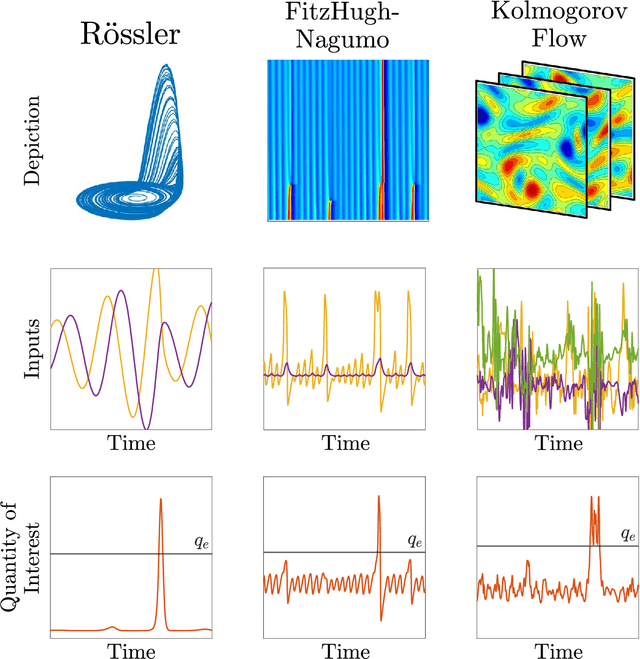
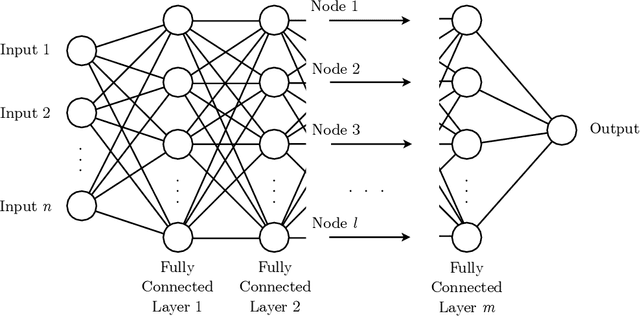
To predict rare extreme events using deep neural networks, one encounters the so-called small data problem because even long-term observations often contain few extreme events. Here, we investigate a model-assisted framework where the training data is obtained from numerical simulations, as opposed to observations, with adequate samples from extreme events. However, to ensure the trained networks are applicable in practice, the training is not performed on the full simulation data; instead we only use a small subset of observable quantities which can be measured in practice. We investigate the feasibility of this model-assisted framework on three different dynamical systems (Rossler attractor, FitzHugh--Nagumo model, and a turbulent fluid flow) and three different deep neural network architectures (feedforward, long short-term memory, and reservoir computing). In each case, we study the prediction accuracy, robustness to noise, reproducibility under repeated training, and sensitivity to the type of input data. In particular, we find long short-term memory networks to be most robust to noise and to yield relatively accurate predictions, while requiring minimal fine-tuning of the hyperparameters.