Robust Counterfactual Policy Optimisation via Nondeterministic Causal Models

Counterfactual inference approaches for sequential decision-making typically assume deterministic causal models, where all randomness stems from latent variables. However, Markov Decision Processes (MDPs) are inherently stochastic. We address this by formalising counterfactual policy optimisation under probabilistic nondeterministic causal models, which properly separates latent confounding from irreducible stochasticity, and here propose a first practical optimisation problem for identifying robust counterfactual policies under a sensitivity analysis framework. We validate our approach on a sepsis treatment simulator, where diabetes status acts as a hidden global confounder.

Robust Counterfactual Inference in Markov Decision Processes

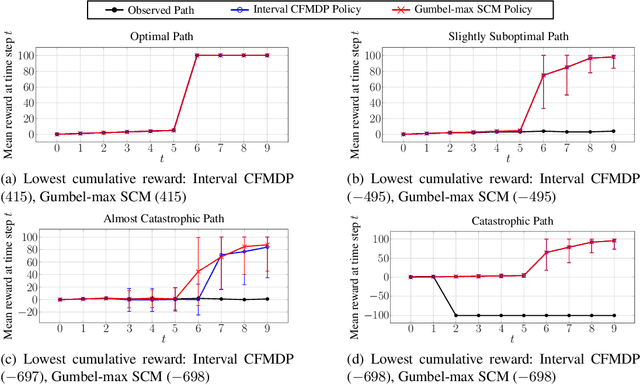
This paper addresses a key limitation in existing counterfactual inference methods for Markov Decision Processes (MDPs). Current approaches assume a specific causal model to make counterfactuals identifiable. However, there are usually many causal models that align with the observational and interventional distributions of an MDP, each yielding different counterfactual distributions, so fixing a particular causal model limits the validity (and usefulness) of counterfactual inference. We propose a novel non-parametric approach that computes tight bounds on counterfactual transition probabilities across all compatible causal models. Unlike previous methods that require solving prohibitively large optimisation problems (with variables that grow exponentially in the size of the MDP), our approach provides closed-form expressions for these bounds, making computation highly efficient and scalable for non-trivial MDPs. Once such an interval counterfactual MDP is constructed, our method identifies robust counterfactual policies that optimise the worst-case reward w.r.t. the uncertain interval MDP probabilities. We evaluate our method on various case studies, demonstrating improved robustness over existing methods.

Counterfactual Influence in Markov Decision Processes

Our work addresses a fundamental problem in the context of counterfactual inference for Markov Decision Processes (MDPs). Given an MDP path $\tau$, this kind of inference allows us to derive counterfactual paths $\tau'$ describing what-if versions of $\tau$ obtained under different action sequences than those observed in $\tau$. However, as the counterfactual states and actions deviate from the observed ones over time, the observation $\tau$ may no longer influence the counterfactual world, meaning that the analysis is no longer tailored to the individual observation, resulting in interventional outcomes rather than counterfactual ones. Even though this issue specifically affects the popular Gumbel-max structural causal model used for MDP counterfactuals, it has remained overlooked until now. In this work, we introduce a formal characterisation of influence based on comparing counterfactual and interventional distributions. We devise an algorithm to construct counterfactual models that automatically satisfy influence constraints. Leveraging such models, we derive counterfactual policies that are not just optimal for a given reward structure but also remain tailored to the observed path. Even though there is an unavoidable trade-off between policy optimality and strength of influence constraints, our experiments demonstrate that it is possible to derive (near-)optimal policies while remaining under the influence of the observation.