A Q-learning Approach for Adherence-Aware Recommendations

In many real-world scenarios involving high-stakes and safety implications, a human decision-maker (HDM) may receive recommendations from an artificial intelligence while holding the ultimate responsibility of making decisions. In this letter, we develop an "adherence-aware Q-learning" algorithm to address this problem. The algorithm learns the "adherence level" that captures the frequency with which an HDM follows the recommended actions and derives the best recommendation policy in real time. We prove the convergence of the proposed Q-learning algorithm to the optimal value and evaluate its performance across various scenarios.

Worst-Case Control and Learning Using Partial Observations Over an Infinite Time-Horizon

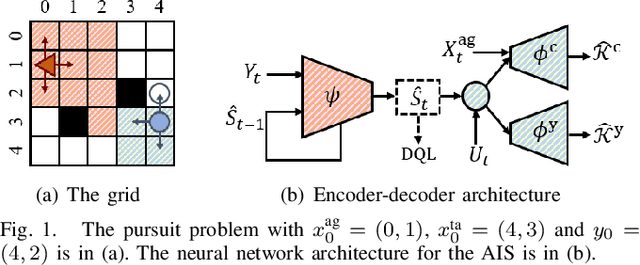
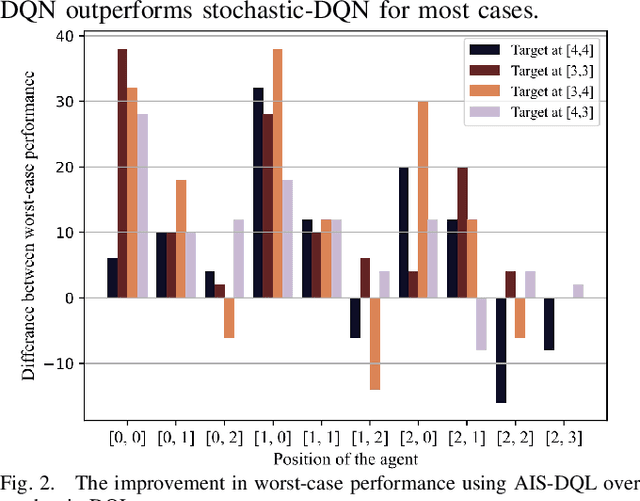
Safety-critical cyber-physical systems require control strategies whose worst-case performance is robust against adversarial disturbances and modeling uncertainties. In this paper, we present a framework for approximate control and learning in partially observed systems to minimize the worst-case discounted cost over an infinite time horizon. We model disturbances to the system as finite-valued uncertain variables with unknown probability distributions. For problems with known system dynamics, we construct a dynamic programming (DP) decomposition to compute the optimal control strategy. Our first contribution is to define information states that improve the computational tractability of this DP without loss of optimality. Then, we describe a simplification for a class of problems where the incurred cost is observable at each time instance. Our second contribution is defining an approximate information state that can be constructed or learned directly from observed data for problems with observable costs. We derive bounds on the performance loss of the resulting approximate control strategy and illustrate the effectiveness of our approach in partially observed decision-making problems with a numerical example.