Closed-form Single UAV-aided Emitter Localization and Trajectory Design Using Doppler and TOA Measurements

In this paper, a single Unmanned-Aerial-Vehicle (UAV)-aided localization algorithm which uses both Doppler and Time of Arrival (ToA) measurements is presented. In contrast to Doppler-based localization algorithms which are based on non-convex functions, exploiting ToA measurements in a Least-Square (LS) Doppler-based cost function, leads to a quadratic convex function whose minimizer lies on a line. Utilizing the ToA measurements in addition to the linear equation of minimizer, a closed form solution is obtained for the emitter location using a constrained LS optimization. In addition, a trajectory design of the UAV is provided which has also closed-form solution. Simulation experiments demonstrate the effectiveness of the proposed algorithm in comparison to some others in the literature.

3D 8-Ary Noise Modulation Using Bayesian- and Kurtosis-based Detectors

This paper presents a novel three-dimensional (3D) 8-ary noise modulation scheme that introduces a new dimension: the mixture probability of a Mixture of Gaussian (MoG) distribution. This proposed approach utilizes the dimensions of mean and variance, in addition to the new probability dimension. Within this framework, each transmitted symbol carries three bits, each corresponding to a distinct sub-channel. For detection, a combination of specialized detectors is employed: a simple threshold based detector for the first sub-channel bit (modulated by the mean), a Maximum-Likelihood (ML) detector for the second sub-channel bit (modulated by the variance), a Kurtosis-based, Jarque-Bera (JB) test, and Bayesian Hypothesis (BHT)-based detectors for the third bit (modulated by the MoG probability). The Kurtosis- and JB-based detectors specifically distinguish between Gaussian (or near-Gaussian) and non-Gaussian MoG distributions by leveraging higher-order statistical measures. The Bit Error Probabilities (BEPs) are derived for the threshold-, Kurtosis-, and BHT-based detectors. The optimum threshold for the Kurtosis-based detector is also derived in a tractable manner. Simulation results demonstrate that a comparably low BEP is achieved for the third sub-channel bit relative to existing two-dimensional (2D) schemes. Simultaneously, the proposed scheme increases the data rate by a factor of 1.5 and 3 compared to the Generalized Quadratic noise modulator and the classical binary KLJN noise modulator, respectively. Furthermore, the Kurtosis-based detector offers a low-complexity solution, achieving an acceptable BEP of approximately 0.06.

Composite Generalized Quadratic Noise Modulation via Signal Addition: Towards Higher Dimensional Noise Modulations

This letter proposes superposing two Generalized Quadratic Noise Modulators (GQNM) by simply adding their outputs. It creates a 16-ary noise modulator that resembles QAM modulators in classical communication. It modulates the information bits on four different means and four different variances. It could also be applied to reach higher-order modulations than 16-ary schemes by adding the outputs of more than two modulators, which is not discussed in detail in this letter and left for future work. By selecting the parameters necessary for satisfying the theoretical distinguishability conditions provided in the paper, we can reach better performances in comparison to the Kirchhoff-Law Johnson Noise (KLJN) modulator and the GQNM modulator, which is verified by the simulations. The better result in terms of smaller Bit Error Probability (BEP) is achieved by increasing the complexity in the modulator, the transmitter, and the detectors in the receiver.

Secure Blind Graph Signal Recovery and Adversary Detection Using Smoothness Maximization

In this letter, we propose a secure blind Graph Signal Recovery (GSR) algorithm that can detect adversary nodes. Some unknown adversaries are assumed to be injecting false data at their respective nodes in the graph. The number and location of adversaries are not known in advance and the goal is to recover the graph signal in the presence of measurement noise and False Data Injection (FDI) caused by the adversaries. Consequently, the proposed algorithm would be a perfect candidate to solve this challenging problem. Moreover, due to the presence of malicious nodes, the proposed method serves as a secure GSR algorithm. For adversary detection, a statistical measure based on differential smoothness is used. Specifically, the difference between the current observed smoothness and the average smoothness excluding the corresponding node. This genuine statistical approach leads to an effective and low-complexity adversary detector. In addition, following malicious node detection, the GSR is performed using a variant of smoothness maximization, which is solved efficiently as a fractional optimization problem using a Dinkelbach's algorithm. Analysis of the detector, which determines the optimum threshold of the detector is also presented. Simulation results show a significant improvement of the proposed method in signal recovery compared to the median GSR algorithm and other competing methods.

Double-Private Distributed Estimation Algorithm Using Differential Privacy and a Key-Like Proportionate Matrix with Its Performance Analysis

In this brief, we present an enhanced privacy-preserving distributed estimation algorithm, referred to as the ``Double-Private Algorithm," which combines the principles of both differential privacy (DP) and cryptography. The proposed algorithm enhances privacy by introducing DP noise into the intermediate estimations of neighboring nodes. Additionally, we employ an inverse of a closed-form reproducible proportionate gain matrix as the cryptographic key matrix to fortify the privacy protection within the proposed double private algorithm. \textcolor{blue}{We improve the algorithm by transmitting alternative variable vectors instead of raw measurements, resulting in enhanced key matrix reconstruction performance. This innovative approach mitigate noise impact, enhancing overall algorithm effectiveness.} We also establish an upper bound for the norm of the error between the non-private Diffusion Least Mean Square (DLMS) algorithm and our double private algorithm. Further, we determine a sufficient condition for the step-size to ensure the mean convergence of the proposed algorithm. Simulation results demonstrate the effectiveness of the proposed algorithm, particularly its ability to attain the final Mean Square Deviation (MSD) comparable to that of the non-private DLMS.

Second-Order Nonlinearity Estimated and Compensated Diffusion LMS Algorithm: Theoretical Upper Bound, Cramer-Rao Lower bound, and Convergence Analysis

In this paper, an algorithm for estimation and compensation of second-order nonlinearity in wireless sensor setwork (WSN) in distributed estimation framework is proposed. First, the effect of second-order nonlinearity on the performance of Diffusion Least Mean Square (DLMS) algorithm is investigated and an upper bound for $l^2$-norm of the error due to nonlinearity is derived mathematically. Second, mean convergence analysis of the DLMS algorithm in presence of second-order nonlinearity is derived. Third, a distributed algorithm is suggested which consists of extra nonlinearity estimation and compensation units. Moreover, considering the second-order nonlinearity, the Cramer-Rao bound (CRB) for estimating both the unknown vector and nonlinearity coefficient vector is calculated, in which the Fisher information matrix is obtained in a closed-form formula. Simulation results demonstrate the effectiveness of the proposed algorithm in improving the performance of distributed estimation in the presence of nonlinear sensors in a WSN.

Markovian Block Sparse Signal Detection Using One Bit Measurements

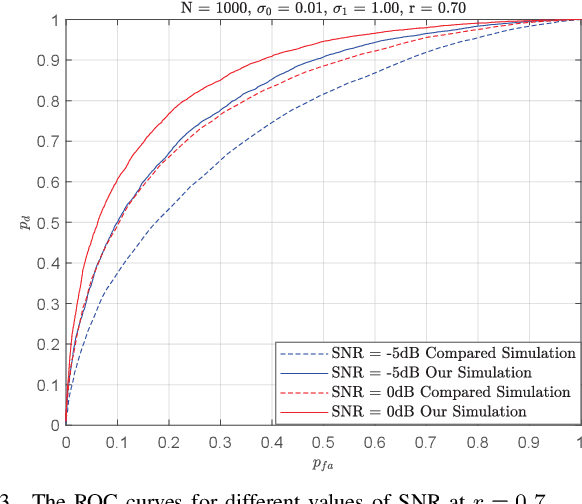
This paper presents a novel sparse signal detection scheme designed for a correlated Markovian Bernoulli-Gaussian sparse signal model, which can equivalently be viewed as a block sparse signal model. Despite the inherent complexity of the model, our approach yields a closed-form detection criterion. Theoretical analyses of the proposed detector are provided, including the computation of false alarm probability and detection probability through closed-form formulas. Simulation results compellingly demonstrate the advantages of our proposed detector compared to an existing detector in the literature.

Proportionate Adaptive Graph Signal Recovery

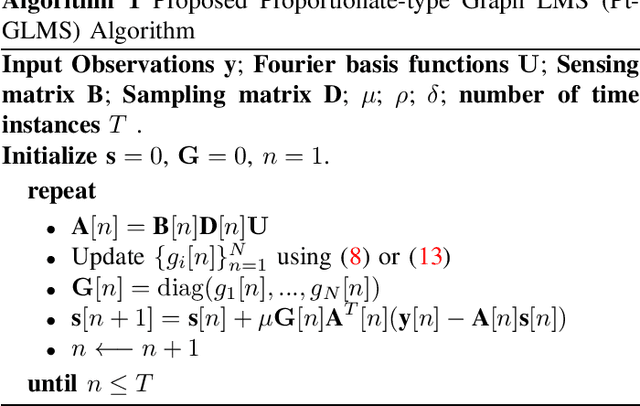
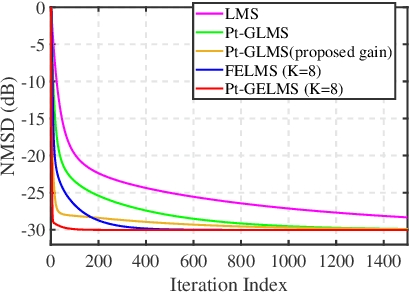
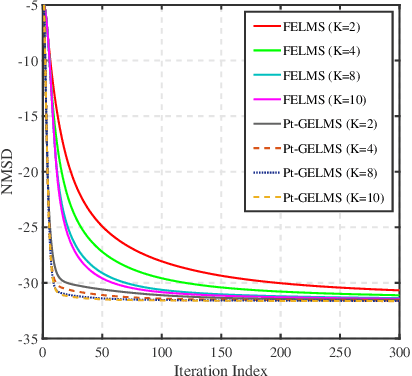
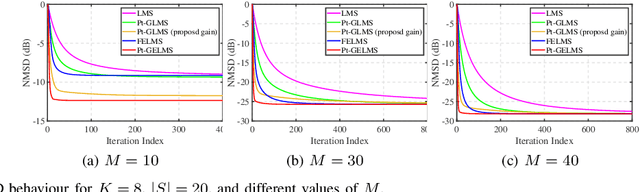
This paper generalizes the proportionate-type adaptive algorithm to the graph signal processing and proposes two proportionate-type adaptive graph signal recovery algorithms. The gain matrix of the proportionate algorithm leads to faster convergence than least mean squares (LMS) algorithm. In this paper, the gain matrix is obtained in a closed-form by minimizing the gradient of the mean-square deviation (GMSD). The first algorithm is the Proportionate-type Graph LMS (Pt-GLMS) algorithm which simply uses a gain matrix in the recursion process of the LMS algorithm and accelerates the convergence of the Pt-GLMS algorithm compared to the LMS algorithm. The second algorithm is the Proportionate-type Graph Extended LMS (Pt-GELMS) algorithm, which uses the previous signal vectors alongside the signal of the current iteration. The Pt-GELMS algorithm utilizes two gain matrices to control the effect of the signal of the previous iterations. The stability analyses of the algorithms are also provided. Simulation results demonstrate the efficacy of the two proposed proportionate-type LMS algorithms.

Robust Adaptive Generalized Correntropy-based Smoothed Graph Signal Recovery with a Kernel Width Learning

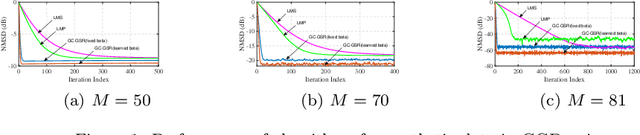
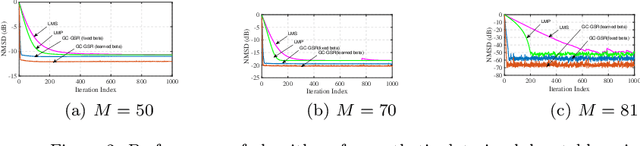
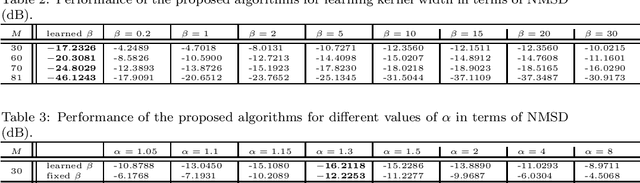
This paper proposes a robust adaptive algorithm for smooth graph signal recovery which is based on generalized correntropy. A proper cost function is defined for this purpose. The proposed algorithm is derived and a kernel width learning-based version of the algorithm is suggested which the simulation results show the superiority of it to the fixed correntropy kernel version of the algorithm. Moreover, some theoretical analysis of the proposed algorithm are provided. In this regard, firstly, the convexity analysis of the cost function is discussed. Secondly, the uniform stability of the algorithm is investigated. Thirdly, the mean convergence analysis is also added. Finally, the complexity analysis of the algorithm is incorporated. In addition, some synthetic and real-world experiments show the advantage of the proposed algorithm in comparison to some other adaptive algorithms in the literature of adaptive graph signal recovery.

Spectral Unmixing of Hyperspectral Images Based on Block Sparse Structure

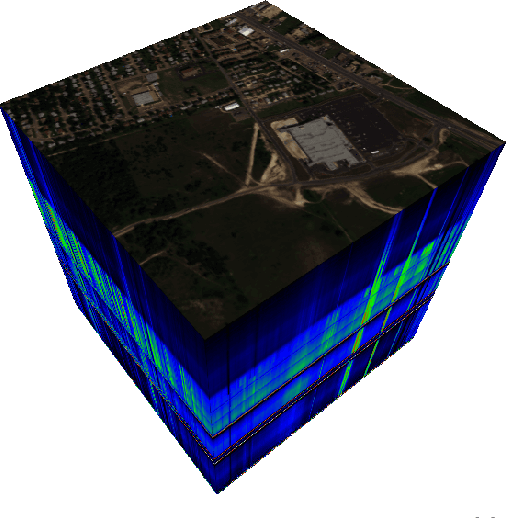
Spectral unmixing (SU) of hyperspectral images (HSIs) is one of the important areas in remote sensing (RS) that needs to be carefully addressed in different RS applications. Despite the high spectral resolution of the hyperspectral data, the relatively low spatial resolution of the sensors may lead to mixture of different pure materials within the image pixels. In this case, the spectrum of a given pixel recorded by the sensor can be a combination of multiple spectra each belonging to a unique material in that pixel. Spectral unmixing is then used as a technique to extract the spectral characteristics of the different materials within the mixed pixels and to recover the spectrum of each pure spectral signature, called endmember. Block-sparsity exists in hyperspectral images as a result of spectral similarity between neighboring pixels. In block-sparse signals, the nonzero samples occur in clusters and the pattern of the clusters is often supposed to be unavailable as prior information. This paper presents an innovative spectral unmixing approach for HSIs based on block-sparse structure and sparse Bayesian learning (SBL) strategy. To evaluate the performance of the proposed SU algorithm, it is tested on both synthetic and real hyperspectral data and the quantitative results are compared to those of other state-of-the-art methods in terms of abundance angel distance (AAD) and mean square error (MSE). The achieved results show the superiority of the proposed algorithm over the other competing methods by a significant margin.