Dim but not entirely dark: Extracting the Galactic Center Excess' source-count distribution with neural nets

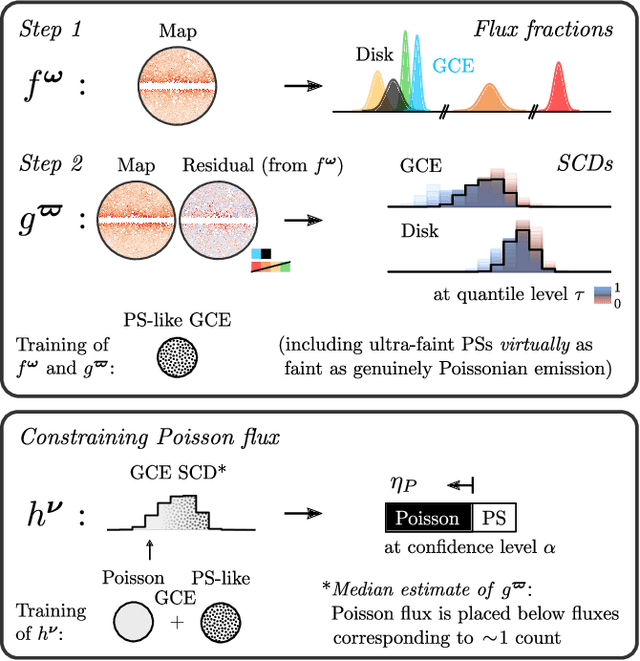
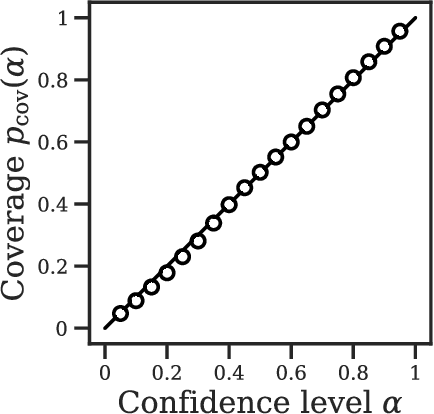
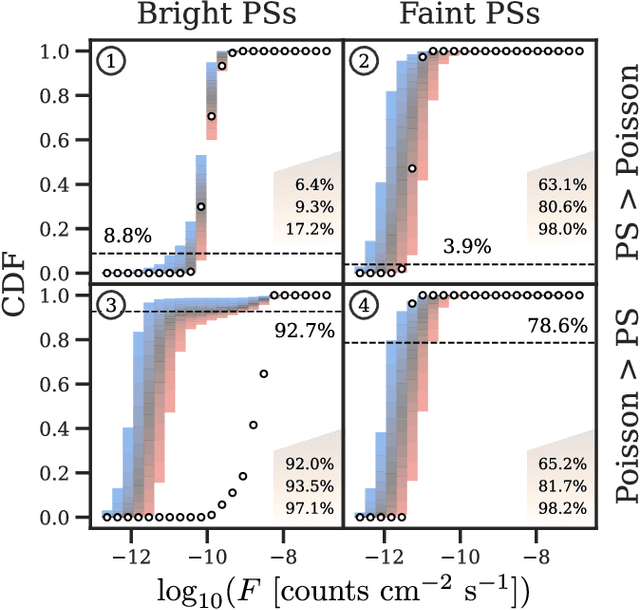
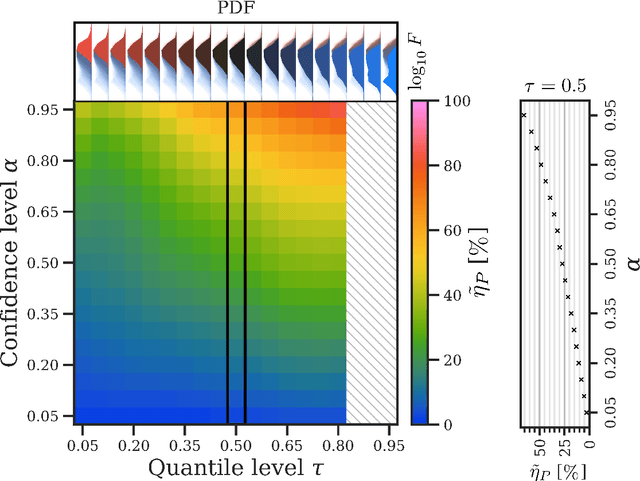
The two leading hypotheses for the Galactic Center Excess (GCE) in the $\textit{Fermi}$ data are an unresolved population of faint millisecond pulsars (MSPs) and dark-matter (DM) annihilation. The dichotomy between these explanations is typically reflected by modeling them as two separate emission components. However, point-sources (PSs) such as MSPs become statistically degenerate with smooth Poisson emission in the ultra-faint limit (formally where each source is expected to contribute much less than one photon on average), leading to an ambiguity that can render questions such as whether the emission is PS-like or Poissonian in nature ill-defined. We present a conceptually new approach that describes the PS and Poisson emission in a unified manner and only afterwards derives constraints on the Poissonian component from the so obtained results. For the implementation of this approach, we leverage deep learning techniques, centered around a neural network-based method for histogram regression that expresses uncertainties in terms of quantiles. We demonstrate that our method is robust against a number of systematics that have plagued previous approaches, in particular DM / PS misattribution. In the $\textit{Fermi}$ data, we find a faint GCE described by a median source-count distribution (SCD) peaked at a flux of $\sim4 \times 10^{-11} \ \text{counts} \ \text{cm}^{-2} \ \text{s}^{-1}$ (corresponding to $\sim3 - 4$ expected counts per PS), which would require $N \sim \mathcal{O}(10^4)$ sources to explain the entire excess (median value $N = \text{29,300}$ across the sky). Although faint, this SCD allows us to derive the constraint $\eta_P \leq 66\%$ for the Poissonian fraction of the GCE flux $\eta_P$ at 95% confidence, suggesting that a substantial amount of the GCE flux is due to PSs.

The GCE in a New Light: Disentangling the $γ$-ray Sky with Bayesian Graph Convolutional Neural Networks

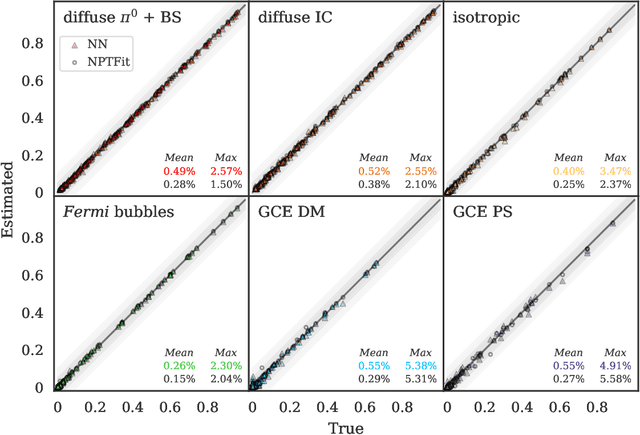
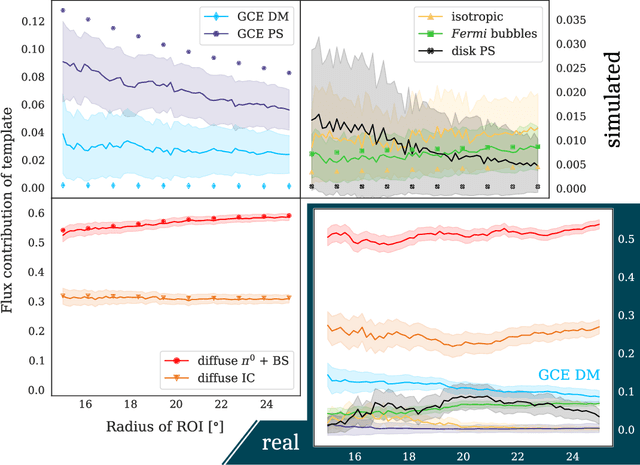
A fundamental question regarding the Galactic Center Excess (GCE) is whether the underlying structure is point-like or smooth. This debate, often framed in terms of a millisecond pulsar or annihilating dark matter (DM) origin for the emission, awaits a conclusive resolution. In this work we weigh in on the problem using Bayesian graph convolutional neural networks. In simulated data, our neural network (NN) is able to reconstruct the flux of inner galaxy emission components to on average $\sim$0.5%, comparable to the non-Poissonian template fit (NPTF). When applied to the actual $\textit{Fermi}$-LAT data, we find that the NN estimates for the flux fractions from the background templates are consistent with the NPTF; however, the GCE is almost entirely attributed to smooth emission. While suggestive, we do not claim a definitive resolution for the GCE, as the NN tends to underestimate the flux of point-sources peaked near the 1$\sigma$ detection threshold. Yet the technique displays robustness to a number of systematics, including reconstructing injected DM, diffuse mismodeling, and unmodeled north-south asymmetries. So while the NN is hinting at a smooth origin for the GCE at present, with further refinements we argue that Bayesian Deep Learning is well placed to resolve this DM mystery.

A unified framework for 21cm tomography sample generation and parameter inference with Progressively Growing GANs

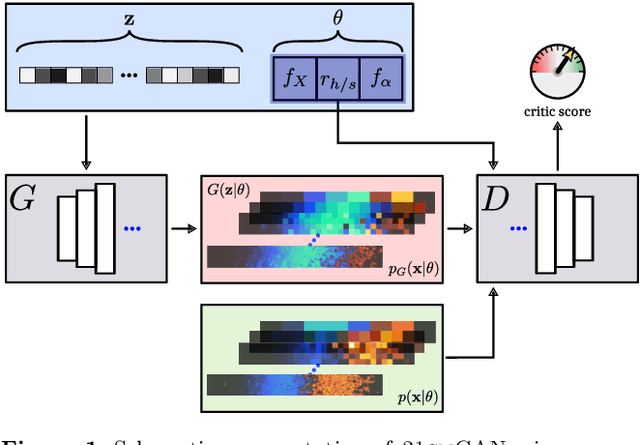
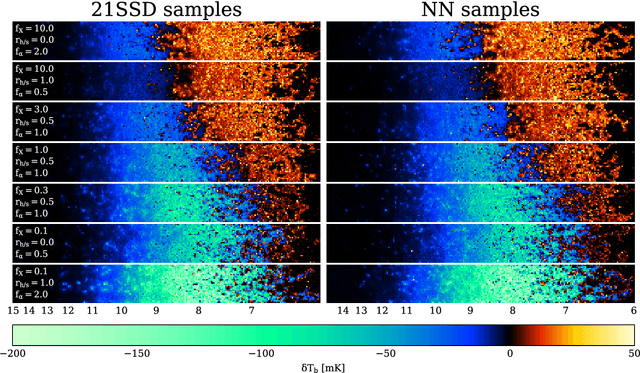
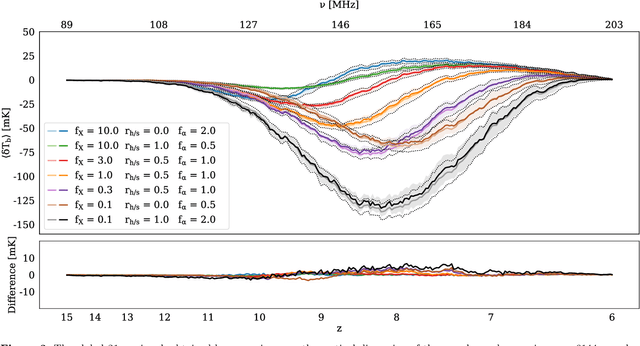
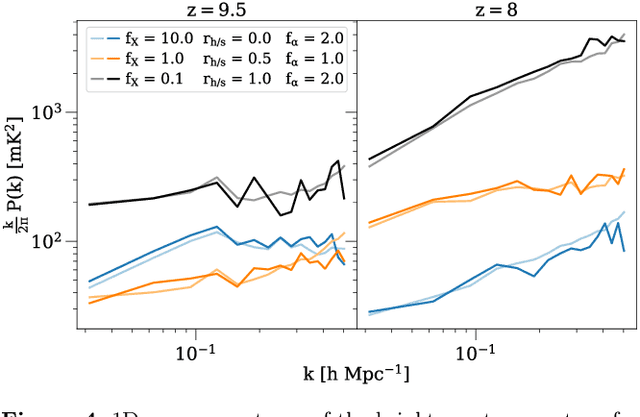
Creating a database of 21cm brightness temperature signals from the Epoch of Reionisation (EoR) for an array of reionisation histories is a complex and computationally expensive task, given the range of astrophysical processes involved and the possibly high-dimensional parameter space that is to be probed. We utilise a specific type of neural network, a Progressively Growing Generative Adversarial Network (PGGAN), to produce realistic tomography images of the 21cm brightness temperature during the EoR, covering a continuous three-dimensional parameter space that models varying X-ray emissivity, Lyman band emissivity, and ratio between hard and soft X-rays. The GPU-trained network generates new samples at a resolution of $\sim 3'$ in a second (on a laptop CPU), and the resulting global 21cm signal, power spectrum, and pixel distribution function agree well with those of the training data, taken from the 21SSD catalogue \citep{Semelin2017}. Finally, we showcase how a trained PGGAN can be leveraged for the converse task of inferring parameters from 21cm tomography samples via Approximate Bayesian Computation.