Online optimal exact identification of a quantum change point

We consider online detection strategies for identifying a change point in a stream of quantum particles allegedly prepared in identical states. We show that the identification of the change point can be done without error via sequential local measurements while attaining the optimal performance bound set by quantum mechanics. In this way, we establish the task of exactly identifying a quantum change point as an instance where local protocols are as powerful as global ones. The optimal online detection strategy requires only one bit of memory between subsequent measurements, and it is amenable to experimental realization with current technology.

Experimentally detecting a quantum change point via Bayesian inference

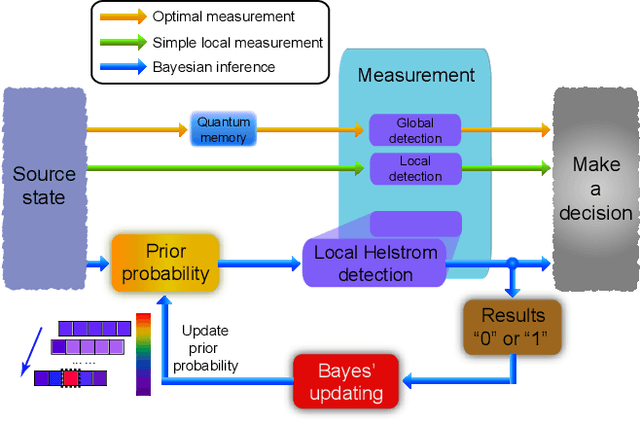
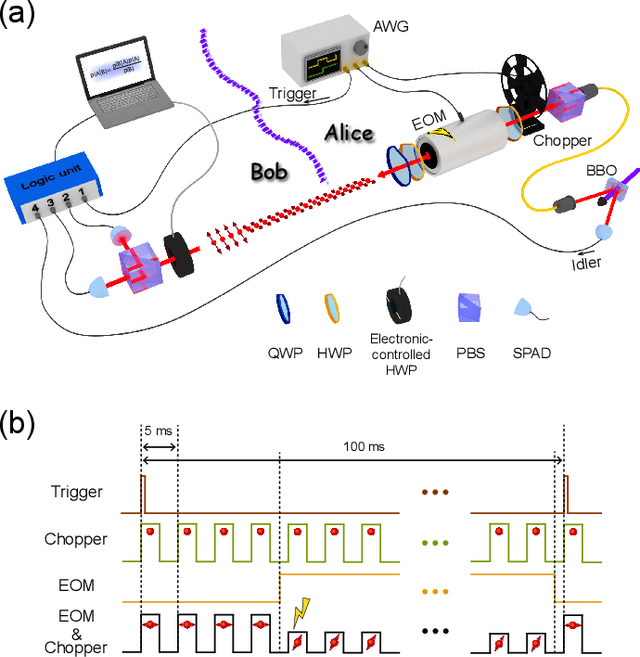
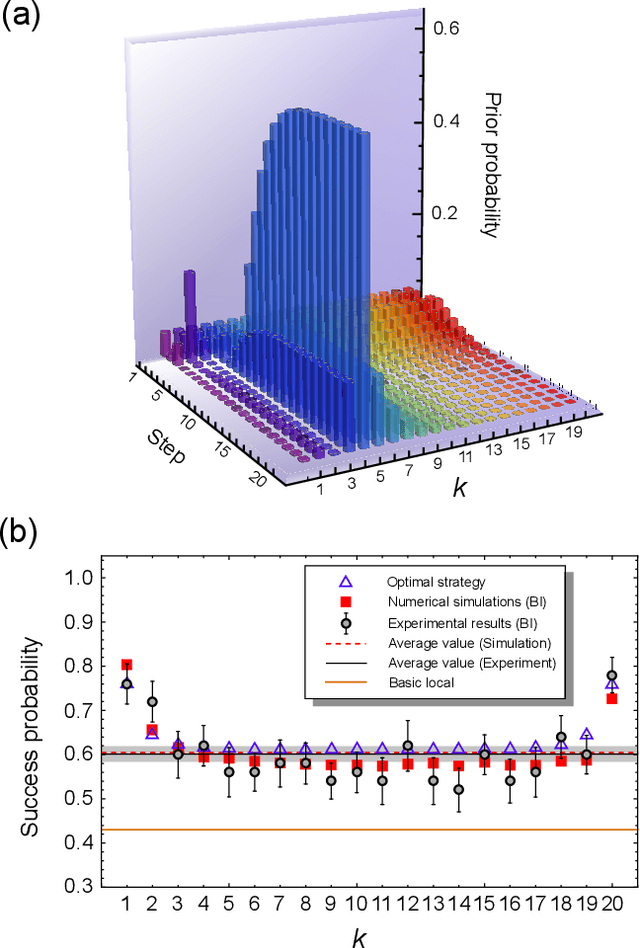
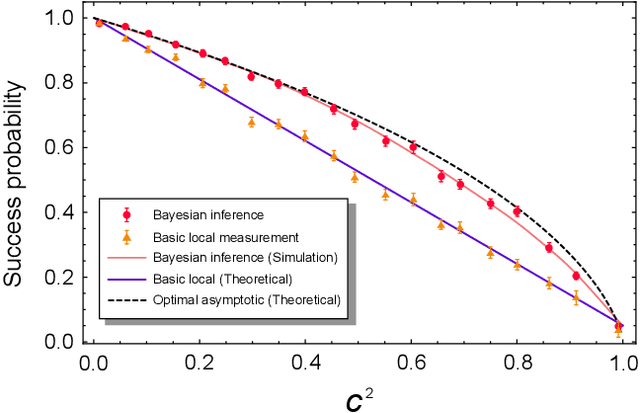
Detecting a change point is a crucial task in statistics that has been recently extended to the quantum realm. A source state generator that emits a series of single photons in a default state suffers an alteration at some point and starts to emit photons in a mutated state. The problem consists in identifying the point where the change took place. In this work, we consider a learning agent that applies Bayesian inference on experimental data to solve this problem. This learning machine adjusts the measurement over each photon according to the past experimental results finds the change position in an online fashion. Our results show that the local-detection success probability can be largely improved by using such a machine learning technique. This protocol provides a tool for improvement in many applications where a sequence of identical quantum states is required.

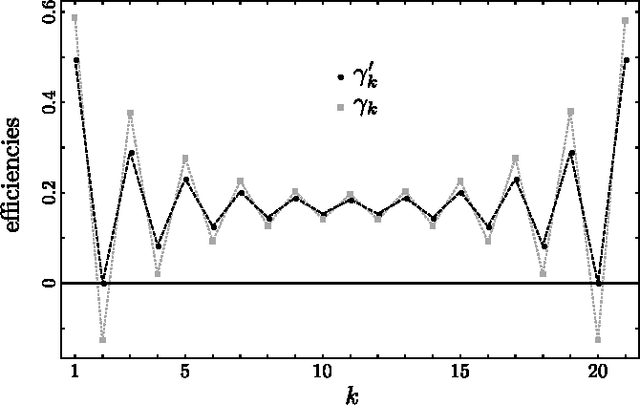
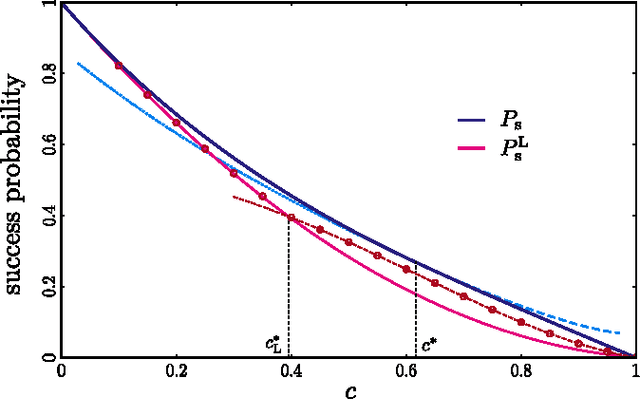
Exact Identification of a Quantum Change Point

The detection of change points is a pivotal task in statistical analysis. In the quantum realm, it is a new primitive where one aims at identifying the point where a source that supposedly prepares a sequence of particles in identical quantum states starts preparing a mutated one. We obtain the optimal procedure to identify the change point with certainty---naturally at the price of having a certain probability of getting an inconclusive answer. We obtain the analytical form of the optimal probability of successful identification for any length of the particle sequence. We show that the conditional success probabilities of identifying each possible change point show an unexpected oscillatory behaviour. We also discuss local (online) protocols and compare them with the optimal procedure.

Inductive supervised quantum learning

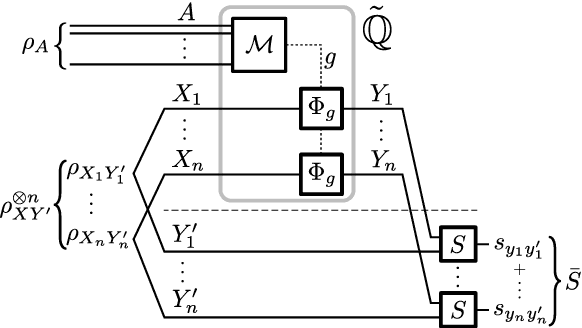
In supervised learning, an inductive learning algorithm extracts general rules from observed training instances, then the rules are applied to test instances. We show that this splitting of training and application arises naturally, in the classical setting, from a simple independence requirement with a physical interpretation of being non-signalling. Thus, two seemingly different definitions of inductive learning happen to coincide. This follows from the properties of classical information that break down in the quantum setup. We prove a quantum de Finetti theorem for quantum channels, which shows that in the quantum case, the equivalence holds in the asymptotic setting, that is, for large number of test instances. This reveals a natural analogy between classical learning protocols and their quantum counterparts, justifying a similar treatment, and allowing to inquire about standard elements in computational learning theory, such as structural risk minimization and sample complexity.