Esmond G. Ng
Deep Learning and Spectral Embedding for Graph Partitioning
Oct 16, 2021

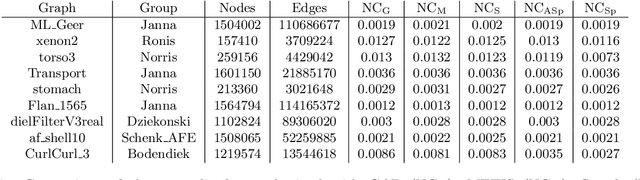

Abstract:We present a graph bisection and partitioning algorithm based on graph neural networks. For each node in the graph, the network outputs probabilities for each of the partitions. The graph neural network consists of two modules: an embedding phase and a partitioning phase. The embedding phase is trained first by minimizing a loss function inspired by spectral graph theory. The partitioning module is trained through a loss function that corresponds to the expected value of the normalized cut. Both parts of the neural network rely on SAGE convolutional layers and graph coarsening using heavy edge matching. The multilevel structure of the neural network is inspired by the multigrid algorithm. Our approach generalizes very well to bigger graphs and has partition quality comparable to METIS, Scotch and spectral partitioning, with shorter runtime compared to METIS and spectral partitioning.
Graph Partitioning and Sparse Matrix Ordering using Reinforcement Learning
Apr 08, 2021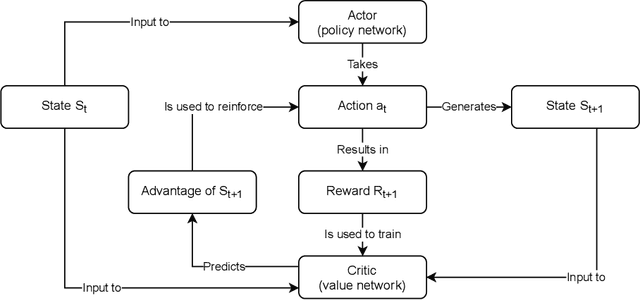
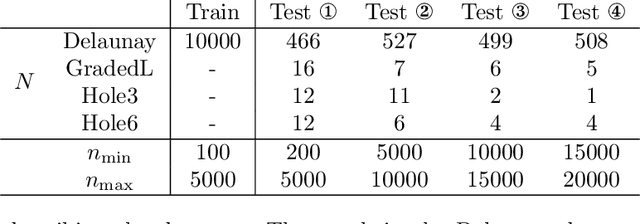

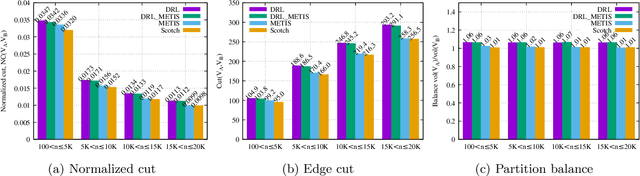
Abstract:We present a novel method for graph partitioning, based on reinforcement learning and graph convolutional neural networks. The new reinforcement learning based approach is used to refine a given partitioning obtained on a coarser representation of the graph, and the algorithm is applied recursively. The neural network is implemented using graph attention layers, and trained using an advantage actor critic (A2C) agent. We present two variants, one for finding an edge separator that minimizes the normalized cut or quotient cut, and one that finds a small vertex separator. The vertex separators are then used to construct a nested dissection ordering for permuting a sparse matrix so that its triangular factorization will incur less fill-in. The partitioning quality is compared with partitions obtained using METIS and Scotch, and the nested dissection ordering is evaluated in the sparse solver SuperLU. Our results show that the proposed method achieves similar partitioning quality than METIS and Scotch. Furthermore, the method generalizes from one class of graphs to another, and works well on a variety of graphs from the SuiteSparse sparse matrix collection.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge